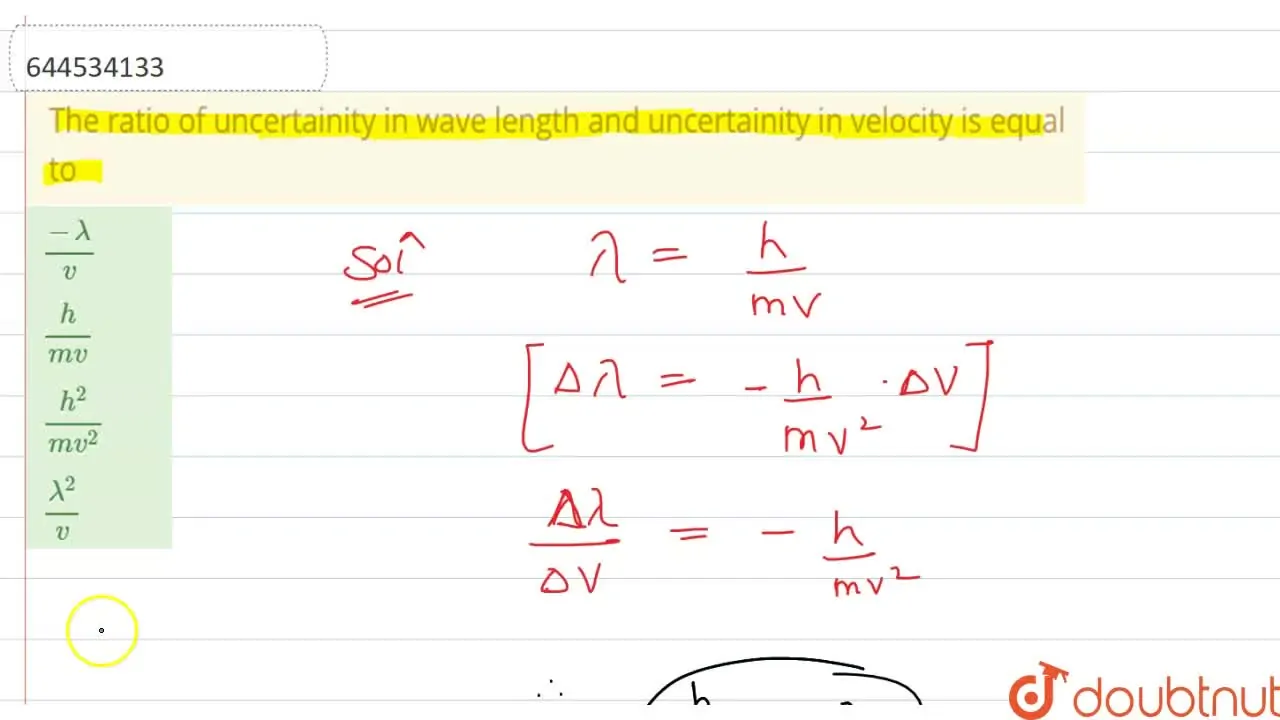
The ratio of uncertainity in wave length and uncertainity in velocity is equal to
−λv
hmv
h2mv2
λ2v
The correct Answer is:A
λ=hmv
∴dλ=−hmv2dv
ΔλΔλ=−hmv×v=−λv
Similar Questions
Calculate the uncertainty in the velocity of an electron, if the uncertainity in position is 100 pm.
View SolutionIf position and momentum of an electron are determined at a time then value of ΔP is obtained 3ΔX, now the uncertainity
in velocity of electron will be :−View SolutionA sine wave has an amplitude A and wavelength λ. The ratio of particle velocity and the wave velocity is equal to (2πA=λ)
View SolutionCalculate the uncertainity in momentum of an electron if uncertainty in its position is 1Å (10−10m).
View SolutionIf uncertainity in position is zero, the uncertainity in momentum of an electron will be
View SolutionCalculate the uncertainity in the position of a cricket ball of mass 150 g if the uncertainity in its velocity is3.53×10−24ms−1
View SolutionCalculate the uncertainity in the position of a cricket ball of mass 150 g if the uncertainity in its velocity is3.53×10−24ms−1
View SolutionState Heisenberg Uncertainity Principle along with its Equation .
View SolutionCalculate the unceertainty in the momentum of a particle if the uncertainity in its position is 6.6×10−32m
View SolutionUncertainity in position of a particles of 15 gram in space is 10−5m. Hence uncertainity in velocity (m//sec) is(h=6.6×10−34Jsec)
View Solution