A travelling wave travelled in string in +x direction with 2cm/s, particle at x=0 oscillates according to equation y (in mm) =2sin(πt+π/3). What will be the slope of the wave at x=3cm and t=1s ?
The correct Answer is:3
To find the slope of the wave at x=3cm and t=1s, we will follow these steps:
Step 1: Write down the wave equation
The given wave equation for the particle at x=0 is:
y(t)=2sin(πt+π3)
Since the wave travels in the +x direction with a speed of 2cm/s, we can express the wave equation as:
y(x,t)=2sin(πt+π3−πx2)
Step 2: Differentiate the wave equation with respect to x
To find the slope of the wave, we need to calculate dydx:
dydx=ddx(2sin(πt+π3−πx2))
Using the chain rule:
dydx=2cos(πt+π3−πx2)⋅(−π2)
This simplifies to:
dydx=−πcos(πt+π3−πx2)
Step 3: Substitute x=3cm and t=1s
Now we substitute x=3cm and t=1s into the slope equation:
dydx=−πcos(π(1)+π3−π(3)2)
Calculating the argument of the cosine:
π(1)+π3−3π2=π+π3−3π2
Finding a common denominator (which is 6):
π=6π6,π3=2π6,3π2=9π6
Thus:
dydx=−πcos(6π6+2π6−9π6)=−πcos(−1π6)
Using the property of cosine:
cos(−θ)=cos(θ)⇒cos(−π6)=cos(π6)=√32
So:
dydx=−π⋅√32=−π√32
Final Answer
The slope of the wave at x=3cm and t=1s is:
dydx=−π√32
---
The given wave equation for the particle at x=0 is:
y(t)=2sin(πt+π3)
Since the wave travels in the +x direction with a speed of 2cm/s, we can express the wave equation as:
y(x,t)=2sin(πt+π3−πx2)
To find the slope of the wave, we need to calculate dydx:
dydx=ddx(2sin(πt+π3−πx2))
Using the chain rule:
dydx=2cos(πt+π3−πx2)⋅(−π2)
This simplifies to:
dydx=−πcos(πt+π3−πx2)
Now we substitute x=3cm and t=1s into the slope equation:
dydx=−πcos(π(1)+π3−π(3)2)
Calculating the argument of the cosine:
π(1)+π3−3π2=π+π3−3π2
Finding a common denominator (which is 6):
π=6π6,π3=2π6,3π2=9π6
Thus:
dydx=−πcos(6π6+2π6−9π6)=−πcos(−1π6)
Using the property of cosine:
cos(−θ)=cos(θ)⇒cos(−π6)=cos(π6)=√32
So:
dydx=−π⋅√32=−π√32
The slope of the wave at x=3cm and t=1s is:
dydx=−π√32
Similar Questions
From a wave equation
y=0.5sin(2π3.2)(64t−x).
the frequency of the wave is
View SolutionA wave is described by the equation y=(1.0mm)sinπ(x2.0cm−t0.01s).
(a) Find time period and wavelength.
(b) Find the speed of particle at x=1.0cm and time t=0.01s.
( c ) What are the speed of the partcle at x=3.0cm, 5.0cmand7.0cm at t=0.01s?
(d) What are the speeds of the partcle at x=1.0cm at t=0.011 , 0.012and0.013s?
View Solution
Knowledge Check
The equation of a wave travelling in a string can be written as y=3cosπ(10t−x). Its wavelength is
A100 cmB2 cmC5 cmDnone of the above
A wave is described by the equation y=(1.0mm)sinπ(x2.0cm−t0.01s).
(a) Find time period and wavelength.
(b) Find the speed of particle at x=1.0cm and time t=0.01s.
( c ) What are the speed of the partcle at x=3.0cm, 5.0cmand7.0cm at t=0.01s?
(d) What are the speeds of the partcle at x=1.0cm at t=0.011 , 0.012and0.013s?
View SolutionA particle moves along the x - axis according to x=A[1+sinωt]. What distance does is travel in time interval from t=0 to t=2.5π/ω ?
View SolutionConsider a standing wave formed on a string . It results due to the superposition of two waves travelling in opposite directions . The waves are travelling along the length of the string in the x - direction and displacements of elements on the string are along the y - direction . Individual equations of the two waves can be expressed as
Y1=6(cm)sin[5(rad/cm)x−4(rad/s)t]
Y2=6(cm)sin[5(rad/cm)x+4(rad/s)t] Here x and y are in cm.
Answer the following questions.
Amplitude of simple harmonic motion of a point on the string that is located at x=1.8cm will be
View SolutionConsider a standing wave formed on a string . It results due to the superposition of two waves travelling in opposite directions . The waves are travelling along the length of the string in the x - direction and displacements of elements on the string are along the y - direction . Individual equations of the two waves can be expressed as
Y1=6(cm)sin[5(rad/cm)x−4(rad/s)t]
Y2=6(cm)sin[5(rad/cm)x+4(rad/s)t] Here x and y are in cm.
Answer the following questions.
If one end of the string is at x=0 , positions of the nodes can be described as
View SolutionA sinusoidal wave is traveling on a string with speed 40 cm/s. The displacement of the particle of the string at x = 10 cm varies with time according to
y = (5.0cm) sin(1.0−4.0s−1)t)The linear density of the string is 4.0 g/cm
View SolutionA particle is performing SHM according to the equation x=(3cm)sin(2πt18+π6), where t is in seconds. The distance travelled by the particle in 39 s is
View SolutionConsider a standing wave formed on a string . It results due to the superposition of two waves travelling in opposite directions . The waves are travelling along the length of the string in the x - direction and displacements of elements on the string are along the y - direction . Individual equations of the two waves can be expressed as
Y1=6(cm)sin[5(rad/cm)x−4(rad/s)t]
Y2=6(cm)sin[5(rad/cm)x+4(rad/s)t] Here x and y are in cm.
Answer the following questions.
Figure 7.104( c) shows the standing wave pattern at t=0 due to superposition of waves given by y1 and y2 in Figs.7.104(a) and (b) . In Fig. 7.104 (c ) , N is a node and A and antinode . At this instant say t=0 , instantaneous velocity of points on the string named as A
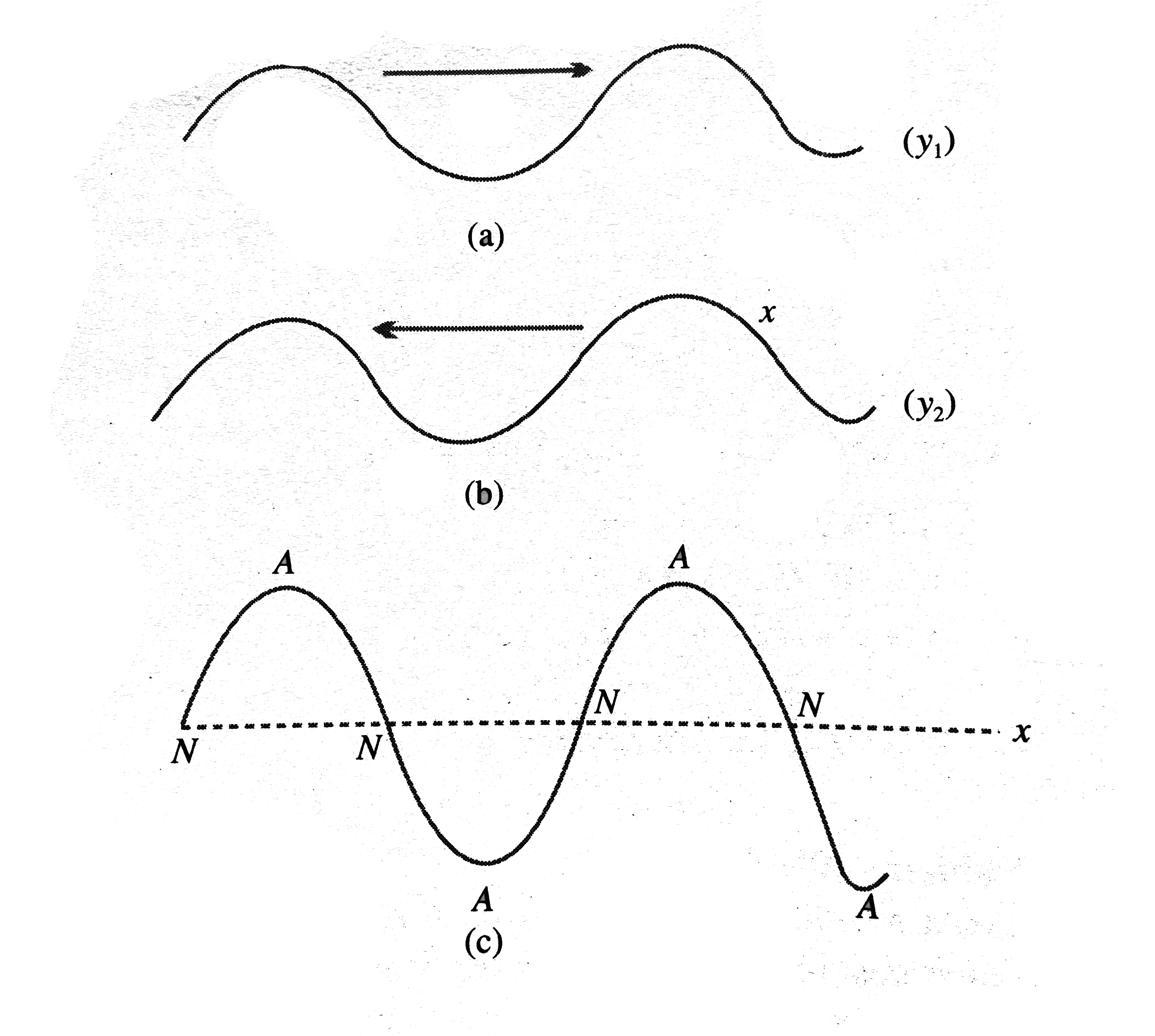
View Solution
