If a×(b×c) is perpendicular to (a×b)×c, we may have
(→a.→c)∣∣→b∣∣2=(→a.→b)(→b.→c)
→a.→b=0
→a.→c=0
→b.→c=0
The correct Answer is:A, C
To solve the problem, we need to analyze the condition given: a×(b×c) is perpendicular to (a×b)×c. This implies that the dot product of these two vectors is zero.
1. Understand the Cross Product:
The cross product of two vectors u and v gives a vector that is perpendicular to both u and v. We will use the vector triple product identity:
u×(v×w)=(u⋅w)v−(u⋅v)w
2. Apply the Triple Product Identity:
For a×(b×c):
a×(b×c)=(a⋅c)b−(a⋅b)c
For (a×b)×c:
(a×b)×c=(c⋅b)a−(c⋅a)b
3. Set Up the Dot Product:
Since the two vectors are perpendicular, we can set up the equation:
((a⋅c)b−(a⋅b)c)⋅((c⋅b)a−(c⋅a)b)=0
4. Expand the Dot Product:
Expanding the dot product gives:
(a⋅c)(c⋅b)(b⋅a)−(a⋅c)(c⋅a)(b⋅b)−(a⋅b)(c⋅b)(b⋅c)+(a⋅b)(c⋅a)(b⋅b)=0
5. Rearranging Terms:
Rearranging and simplifying the equation will lead us to conditions on the vectors a,b, and c.
6. Conclusion:
After simplification, we can conclude that one of the conditions for the vectors to satisfy the perpendicularity condition is:
- a⋅c=0 or b⋅c=0
Final Result
Thus, the conditions that satisfy the given problem are:
- a⋅c=0 (indicating a is perpendicular to c)
- b⋅c=0 (indicating b is perpendicular to c)
The cross product of two vectors u and v gives a vector that is perpendicular to both u and v. We will use the vector triple product identity:
u×(v×w)=(u⋅w)v−(u⋅v)w
For a×(b×c):
a×(b×c)=(a⋅c)b−(a⋅b)c
(a×b)×c=(c⋅b)a−(c⋅a)b
Since the two vectors are perpendicular, we can set up the equation:
((a⋅c)b−(a⋅b)c)⋅((c⋅b)a−(c⋅a)b)=0
Expanding the dot product gives:
(a⋅c)(c⋅b)(b⋅a)−(a⋅c)(c⋅a)(b⋅b)−(a⋅b)(c⋅b)(b⋅c)+(a⋅b)(c⋅a)(b⋅b)=0
Rearranging and simplifying the equation will lead us to conditions on the vectors a,b, and c.
After simplification, we can conclude that one of the conditions for the vectors to satisfy the perpendicularity condition is:
- a⋅c=0 or b⋅c=0
Thus, the conditions that satisfy the given problem are:
- a⋅c=0 (indicating a is perpendicular to c)
- b⋅c=0 (indicating b is perpendicular to c)
Topper's Solved these Questions
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART : 1MATHEMATICS SEC - 1
Explore 1 Video
- TEST PAPERSBook:RESONANCE ENGLISHChapter:TEST PAPERSExercise:PART : 1MATHEMATICS
Explore 9 Videos
- TEST PAPERBook:RESONANCE ENGLISHChapter:TEST PAPERExercise:MATHEMATICS
Explore 48 Videos
- TEST SERIESBook:RESONANCE ENGLISHChapter:TEST SERIESExercise:MATHEMATICS
Explore 132 Videos
Similar Questions
If →a×(→b×→c) is perpendicular to (→a×→b)×→c, we may have
View SolutionIf →a×(→b×→c) is perpendicular to (→a×→b)×→c, we may have a. (→a.→c)∣∣→b∣∣2=(→a.→b)(→b.→c)(→c.→a) b. →a.→b=0 c. →a.→c=0 d. →b.→c=0
View Solution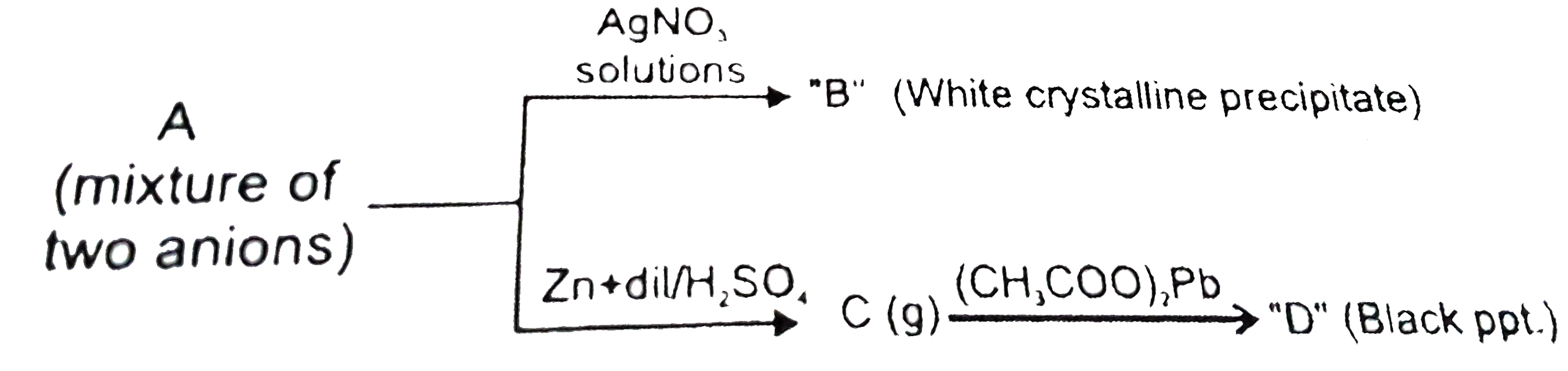
Then A may have :
View SolutionAn object may have
View SolutionAn object may have
View SolutionStatement I: If a is perpendicular to b and c, then a×(b×c)=0 Statement II: if a is perpendicular to b and c, then b×c=0
View SolutionA vector perpendicular to (4ˆi−3ˆj) may be :
View SolutionA vector perpendicular to (4ˆi−3ˆj) may be :
View SolutionIf →b is not perpendicular to →c, then find the vector →r satisfying the equyation →r×→b=→a×→b and →r.→c=0.
View SolutionStatement-I The number of vectors of unit length and perpendicular to both the vectors ˆi+ˆjandˆj+ˆk is zero.
Statement-II a and b are two non-zero and non-parallel vectors it is true that a×b is perpendicular to the plane containing a and b
View Solution