The specific rotation of two glucose anomers are α=+110∘ and β=19∘ and for the constant equilibrium mixtures is +52.7∘. Calculate the percentage compositions of the anomers in the equilibrium mixture.
The correct Answer is:α−anomer =37.2%,β− anomer =62.8%.
To solve the problem of calculating the percentage compositions of the two glucose anomers (alpha and beta) in the equilibrium mixture, we can follow these steps:
1. Define Variables:
- Let the percentage composition of the alpha anomer be X%.
- Therefore, the percentage composition of the beta anomer will be (100−X)%.
2. Use the Formula for Optical Rotation:
- The specific rotation of the equilibrium mixture can be expressed as:
(α)mix=X⋅(α)alpha+(100−X)⋅(α)beta100
- Where:
- (α)alpha=+110∘ (specific rotation of alpha anomer)
- (α)beta=+19∘ (specific rotation of beta anomer)
- (α)mix=+52.7∘ (specific rotation of the equilibrium mixture)
3. Set Up the Equation:
- Substitute the known values into the equation:
52.7=X⋅110+(100−X)⋅19100
4. Multiply Through by 100 to Eliminate the Denominator:
5270=110X+1900−19X
5. Combine Like Terms:
- Combine the X terms:
5270=91X+1900
6. Isolate X:
- Subtract 1900 from both sides:
5270−1900=91X
3370=91X
7. Solve for X:
- Divide both sides by 91:
X=337091≈37.2
8. Calculate the Percentage of Beta:
- Now, calculate the percentage of the beta anomer:
Percentage of beta=100−X=100−37.2=62.8
Final Answer:
- The percentage composition of the alpha anomer is approximately 37.2%.
- The percentage composition of the beta anomer is approximately 62.8%.
- Let the percentage composition of the alpha anomer be X%.
- Therefore, the percentage composition of the beta anomer will be (100−X)%.
- The specific rotation of the equilibrium mixture can be expressed as:
(α)mix=X⋅(α)alpha+(100−X)⋅(α)beta100
- Where:
- (α)alpha=+110∘ (specific rotation of alpha anomer)
- (α)beta=+19∘ (specific rotation of beta anomer)
- (α)mix=+52.7∘ (specific rotation of the equilibrium mixture)
- Substitute the known values into the equation:
52.7=X⋅110+(100−X)⋅19100
5270=110X+1900−19X
- Combine the X terms:
5270=91X+1900
- Subtract 1900 from both sides:
5270−1900=91X
3370=91X
- Divide both sides by 91:
X=337091≈37.2
- Now, calculate the percentage of the beta anomer:
Percentage of beta=100−X=100−37.2=62.8
- The percentage composition of the alpha anomer is approximately 37.2%.
- The percentage composition of the beta anomer is approximately 62.8%.
Topper's Solved these Questions
- BIOMOLECULESBOOK - RESONANCE ENGLISHCHAPTER - BIOMOLECULESEXERCISE - Ex-2(Single correct)Part-II
17 Videos
- BIOMOLECULESBOOK - RESONANCE ENGLISHCHAPTER - BIOMOLECULESEXERCISE - Ex-2(More correct)Part-II
14 Videos
- BIOMOLECULESBOOK - RESONANCE ENGLISHCHAPTER - BIOMOLECULESEXERCISE - Ex-1(Assertion/Reasoning)Part-III
10 Videos
- BASIC CONCEPTSBOOK - RESONANCE ENGLISHCHAPTER - BASIC CONCEPTSEXERCISE - ORGANIC CHEMISTRY(BASIC CONCEPTS)
27 Videos
- BIOMOLECULES & POLYMERBOOK - RESONANCE ENGLISHCHAPTER - BIOMOLECULES & POLYMEREXERCISE - ORGANIC CHEMISTRY(Biomolecules & Polymer)
34 Videos
Similar Questions
An organic compound P exists in two enantiomeric forms, which have specific optical rotation values [α]=±100∘. The optical rotation of a mixture of these two enantiomers is −50∘. Calculate the percentage of that enantiomer which is in lower concentration in the mixture.
View SolutionHow much of the α-anomer and β-anomers are present in an equilibrium mixture with a specific rotation of +52.6∘?
View Solution
Knowledge Check
The optical rotation of the ∝- form of a pyranose is +150.70, that of the β-form is +52.80. In solution and equilibrium mixture of the anomers has an optical rotation of +80.20. The percentage of the ∝- form at equilibrium is
A0.28B0.32C0.68D0.72D (+) Glucose has melting point 146∘C and specific rotation [α]25D is + 112∘C. Another D(+) Glucose has melting point 150∘C and specific rotational [α]25Dis +18.7∘C. The two form have significantly different optical rotation but when an aqueous solution of either form is allowed to stand, it rotation changes. The specific rotation of one form decreases and rotation of other increases until both solution show the same value +52.7∘. The change in rotation towards an equilibrium value is called mutarotation.
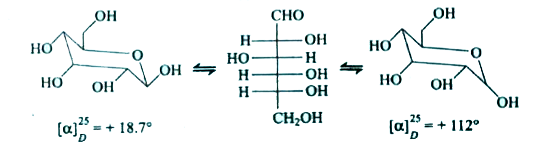
What percentage of β-D-(+) glucopyranose found at equilibrium in the aqueous solution?A50%B≈100%C38%D64%D (+) Glucose has melting point 146∘C and specific rotation [α]25D is + 112∘C. Another D(+) Glucose has melting point 150∘C and specific rotational [α]25Dis +18.7∘C. The two form have significantly different optical rotation but when an aqueous solution of either form is allowed to stand, it rotation changes. The specific rotation of one form decreases and rotation of other increases until both solution show the same value +52.7∘. The change in rotation towards an equilibrium value is called mutarotation.
Mutarotation is characteristic feature of:AEpimerBEnantiomerCAnomerDRing chain isomer
The optical rotation of the α-form of a pyranose is +150.7∘, that of the β-form is +52.8∘. In solution an equilibrium mixture of these anomers has an optical rotation of +80.2∘. The precentage of the α-form in equilibrium mixture is :
View Solutionα−(D) glucose ⇔β−(D) glucose, equilibrium constant for this s 1.8. The percentage of α−(D) glucose at equilibrium is
View Solution3.00 mol of PCl5 kept in 1L closed reaction vessel was allowed to attain equilibrium at 3.80K. Calculate composition of the mixture at equilibrium Kc=1.80
View SolutionThe frequency of two alleles in a gene pool is 0.19 ( A) and 0.81 ( a) . Assume that the population is in Hardy-Weinberg equilibrium and
(a) Calculate the percentage of heterozygous individuals in the population.
(b) Calculate the percentage of homozygous recessive in the population .
View SolutionA container whose volume is V contains an equilibrium mixture that consists of 2 mol each of PCl5,PCl3andCl2 (all as gases) . The pressure is 30.3975 kpa and temperature is T. A certain amount of Cl2 (g) is now introduced keeping the pressure and temperature constant until the equilibrium volume is 2V. Calculate the amount of Cl2 that was added and the value of kp.
View Solution
