Assertion (A) : The electrostatic energy stored in capacitor plus magnetic energy stored in inductor will always be zero in series LCR circuit driven by ac voltage source under condition of resonance.
Reason (R ) : The complete voltage of ac source appears across the resistor in a series LCR circuit driven by ac voltage source under condition of resonance.
Statement-1 is true, Statement-2: is true, Statement-2 is a correct explanation for Statement-1.
Statement-1 is true, Statement-2: is true, Statement-2 is NOT a correct explanation for Statement-1.
Statement-1 is true but statement-2 is false
Statement-1 is false, Statement-2 is true
The correct Answer is:D
To solve the question, we need to evaluate the assertion and reason provided in the context of an LCR circuit driven by an AC voltage source under resonance conditions.
1. Understanding the Assertion (A):
- The assertion states that the electrostatic energy stored in the capacitor plus the magnetic energy stored in the inductor will always be zero in a series LCR circuit at resonance.
- The energy stored in a capacitor is given by UC=12CV2 and the energy stored in an inductor is given by UL=12LI2.
- At resonance, the circuit behaves such that the impedance is minimized and the current reaches its maximum value.
2. Evaluating the Total Energy:
- Since both C (capacitance) and L (inductance) are positive quantities, and V2 and I2 are also positive, we can conclude that:
UC+UL=12CV2+12LI2>0
- Therefore, the total energy stored in the capacitor and inductor cannot be zero. Thus, the assertion is false.
3. Understanding the Reason (R):
- The reason states that the complete voltage of the AC source appears across the resistor in a series LCR circuit under resonance conditions.
- At resonance, the impedance Z of the circuit is minimized and equals the resistance R. This means that:
Z=R
- The voltage across the resistor can be expressed as:
VR=I⋅R
- Since the current I is at its maximum value Imax, the voltage across the resistor equals the total voltage from the AC source:
V=Imax⋅R
- Therefore, the reason is true.
4. Conclusion:
- The assertion (A) is false, and the reason (R) is true. Thus, the correct answer is that the assertion is false, but the reason is true.
Final Answer:
- Assertion (A): False
- Reason (R): True
- The assertion states that the electrostatic energy stored in the capacitor plus the magnetic energy stored in the inductor will always be zero in a series LCR circuit at resonance.
- The energy stored in a capacitor is given by UC=12CV2 and the energy stored in an inductor is given by UL=12LI2.
- At resonance, the circuit behaves such that the impedance is minimized and the current reaches its maximum value.
- Since both C (capacitance) and L (inductance) are positive quantities, and V2 and I2 are also positive, we can conclude that:
UC+UL=12CV2+12LI2>0
- Therefore, the total energy stored in the capacitor and inductor cannot be zero. Thus, the assertion is false.
- The reason states that the complete voltage of the AC source appears across the resistor in a series LCR circuit under resonance conditions.
- At resonance, the impedance Z of the circuit is minimized and equals the resistance R. This means that:
Z=R
- The voltage across the resistor can be expressed as:
VR=I⋅R
- Since the current I is at its maximum value Imax, the voltage across the resistor equals the total voltage from the AC source:
V=Imax⋅R
- Therefore, the reason is true.
- The assertion (A) is false, and the reason (R) is true. Thus, the correct answer is that the assertion is false, but the reason is true.
- Assertion (A): False
- Reason (R): True
Topper's Solved these Questions
Similar Questions
In series LCR AC circuit, the voltage of the source at any instant is equal to
View SolutionIn a series LCR circuit which is connected to an AC vottage source, choose the incorrect statement
View SolutionIn a series LCR circuit, the frequency of the source is more than resonance frequency. The current in the circuit leads the voltage in phases(True/false).
View SolutionIn a series LCR circuit the rms voltage across the inductance , capacitance and resistance are respectively 4 V, 8 V and 5 V . The RMS voltage of the AC source in the circuit is
View SolutionAn AC voltage is applied acrss a series combination of L and R. If the voltage drop across the resistor and inductor are 20V and 15V respectiely, then applied peak voltage is
View SolutionIn series LCR circuit, the voltages across R, L and C are shown in the figure. The voltage of applied source
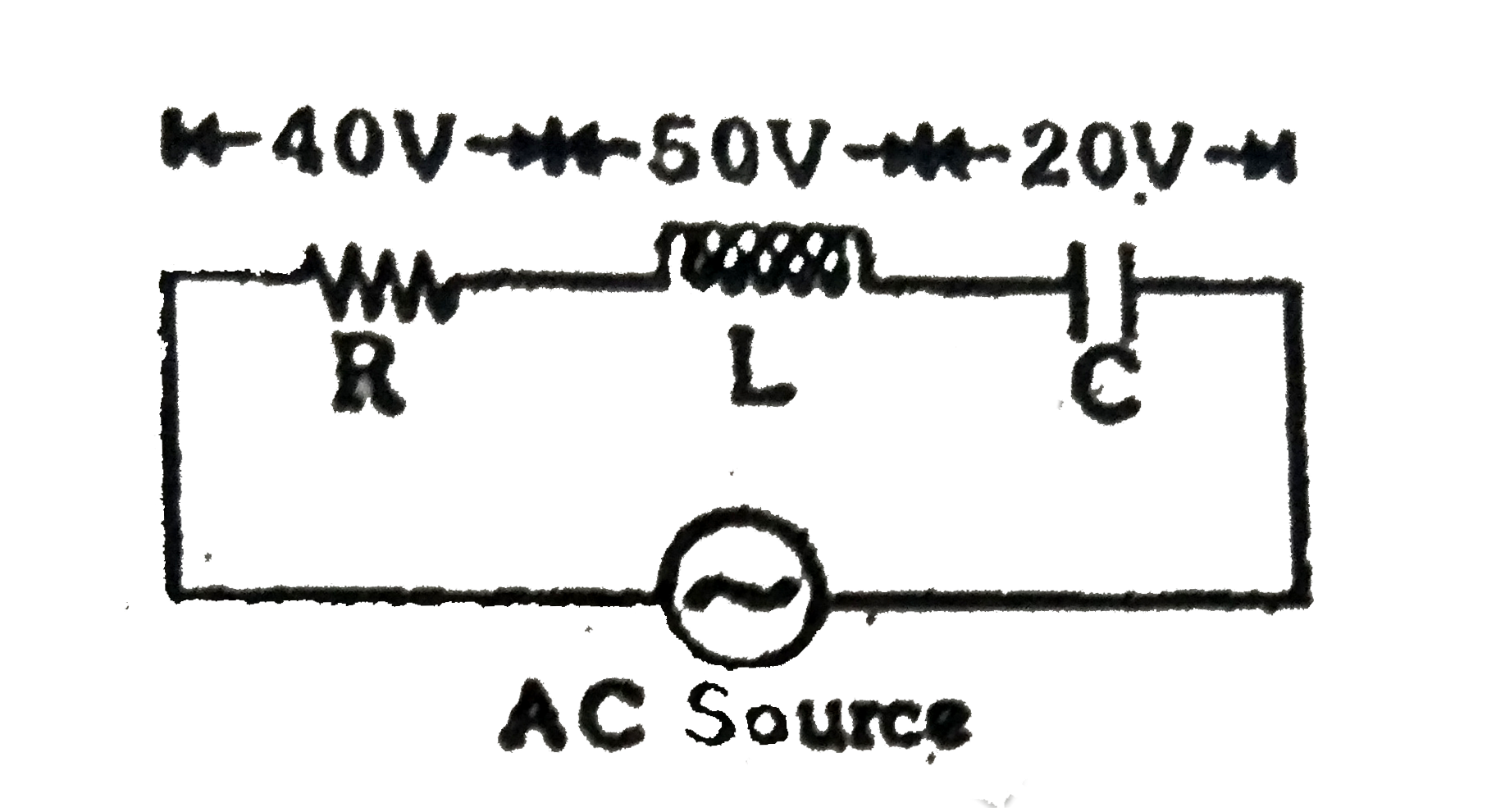
View SolutionIn LCR series circuit source voltage is 120 volt and voltage in inductor 50 volt and resistance is 40 volt then determine voltage in capacitor.
View SolutionFor an LCR series circuit with an ac source of angular frequency ω.
View SolutionCan the peak voltage across the inductor be greater than the peak voltage of the source in an LCR circuit?
View SolutionAssertion : In series L−C−R,AC circuit, current and voltage are in same phase at resonance.
Reason : In series L−C−R,AC circuit, resonant frequency does not depend on the value of resistance. Hence, current at resonance does not depend on resistance.
View Solution