A liquid with coefficient of volume expansion γ is filled in a container of a material having coefficient of linear expansion α . If the liquid overflows on heating, then
γ>3α
γ<3α
γ=3α
none of these
The correct Answer is:A
To solve the problem, we need to analyze the expansion of both the liquid and the container when the temperature increases. Here's the step-by-step solution:
Step 1: Understand the coefficients of expansion
- The coefficient of volume expansion of the liquid is denoted as γ.
- The coefficient of linear expansion of the container material is denoted as α.
Step 2: Relate the coefficients of expansion
- The relationship between the coefficient of volume expansion γ and the coefficient of linear expansion α is given by:
γ=3α
This means that for every unit increase in temperature, the volume of the liquid expands more than the volume of the container.
Step 3: Calculate the change in volume for the liquid
- When the temperature increases by ΔT, the change in volume of the liquid can be expressed as:
ΔVL=γΔT
Step 4: Calculate the change in volume for the container
- The change in volume of the container, which has a linear expansion coefficient α, can be expressed as:
ΔVC=3αΔT
Step 5: Set up the inequality for overflow
- For the liquid to overflow, the change in volume of the liquid must be greater than the change in volume of the container:
ΔVL>ΔVC
Substituting the expressions for ΔVL and ΔVC:
γΔT>3αΔT
Step 6: Simplify the inequality
- Since ΔT is common on both sides and is positive, we can divide both sides by ΔT:
γ>3α
Conclusion
- This indicates that for the liquid to overflow, the coefficient of volume expansion of the liquid must be greater than three times the coefficient of linear expansion of the container material.
Final Result
- Therefore, the condition for the liquid to overflow when heated is:
γ>3α
- The coefficient of volume expansion of the liquid is denoted as γ.
- The coefficient of linear expansion of the container material is denoted as α.
- The relationship between the coefficient of volume expansion γ and the coefficient of linear expansion α is given by:
γ=3α
This means that for every unit increase in temperature, the volume of the liquid expands more than the volume of the container.
- When the temperature increases by ΔT, the change in volume of the liquid can be expressed as:
ΔVL=γΔT
- The change in volume of the container, which has a linear expansion coefficient α, can be expressed as:
ΔVC=3αΔT
- For the liquid to overflow, the change in volume of the liquid must be greater than the change in volume of the container:
ΔVL>ΔVC
Substituting the expressions for ΔVL and ΔVC:
γΔT>3αΔT
- Since ΔT is common on both sides and is positive, we can divide both sides by ΔT:
γ>3α
- This indicates that for the liquid to overflow, the coefficient of volume expansion of the liquid must be greater than three times the coefficient of linear expansion of the container material.
- Therefore, the condition for the liquid to overflow when heated is:
γ>3α
Topper's Solved these Questions
Similar Questions
Unit of coefficient of linear expansion
View SolutionDefine the coefficient of linear expansion.
View SolutionOn heating a liquid having coefficient of volume expension α in a container having coefficient of linear expansion α/2, the level of the liquid in the container would
View SolutionA fluid having a thermal coefficient of volume expansion γ is filled in a cylindrical vessel up to a height h0. The coefficient of linear thermal expansion of the material of the vessel is α . If the fluid is heated with the vessel , then find the level of liquid when temperature increases by Δθ.
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
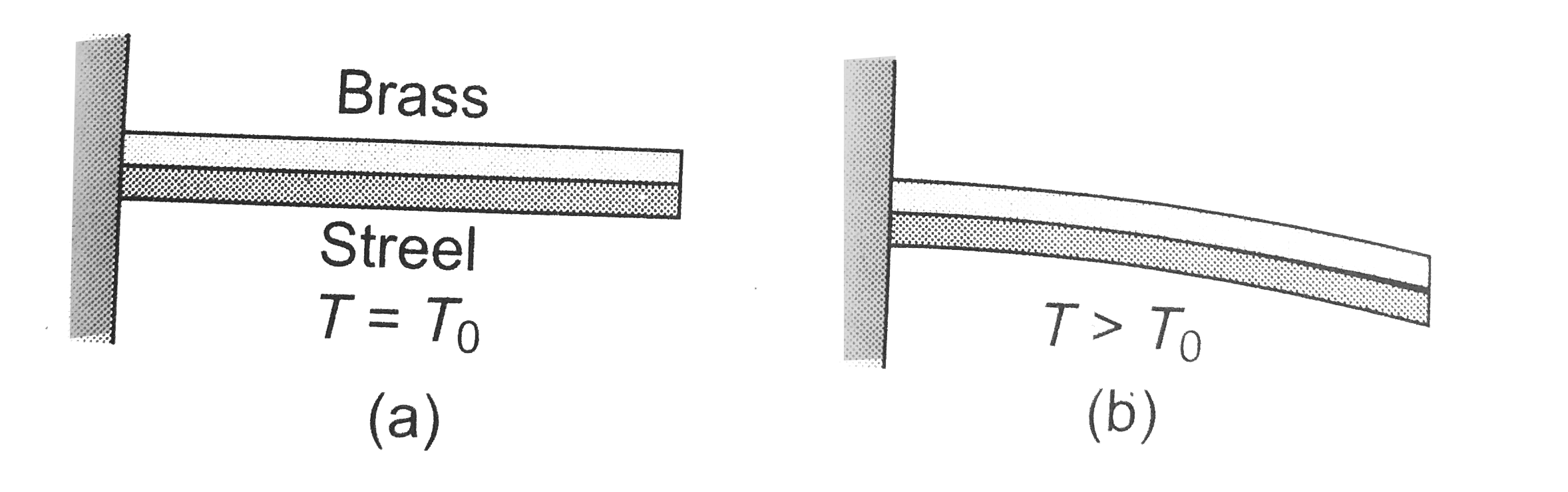 View Solution
View SolutionMercury has a small coefficient of volume expansion.
View SolutionLet γ : coefficient of volume expansion of the liquid and α : coefficient of linear expansion of the material of the tube
View SolutionCoefficient of linear and coefficient of superficial expansion
View SolutionCoefficient of areal expansion of a solid is 2×10−5.∘C−1. Calculate its coefficient of linear expansion.
View SolutionRelate the thermal coefficient of linear expansion, α, the the thermal coefficient of area expansion β.
View Solution
