The energy associated with each degree of freedom of a molecule
1/2RT
1/2 KT
3/2 RT
3/2KT
The correct Answer is:B
To solve the question regarding the energy associated with each degree of freedom of a molecule, we can follow these steps:
1. Understand the Concept of Degrees of Freedom:
- Degrees of freedom refer to the number of independent ways in which a molecule can move or store energy. For a gas molecule, these can include translational, rotational, and vibrational motions.
2. Recall the Equipartition Theorem:
- The equipartition theorem states that energy is equally distributed among all degrees of freedom. According to this theorem, each degree of freedom contributes an amount of energy to the total energy of the system.
3. Identify the Energy Contribution per Degree of Freedom:
- According to the equipartition theorem, the energy associated with each degree of freedom is given by the formula:
E=12kT
- Here, k is the Boltzmann constant, and T is the absolute temperature in Kelvin.
4. Evaluate the Given Options:
- The options provided are:
- (A) 12RT
- (B) 12kT
- (C) 32RT
- (D) 32kT
- From our understanding of the equipartition theorem, the correct expression for the energy associated with each degree of freedom is 12kT.
5. Select the Correct Answer:
- Based on the analysis, the correct answer is option (B) 12kT.
Final Answer:
The energy associated with each degree of freedom of a molecule is 12kT.
- Degrees of freedom refer to the number of independent ways in which a molecule can move or store energy. For a gas molecule, these can include translational, rotational, and vibrational motions.
- The equipartition theorem states that energy is equally distributed among all degrees of freedom. According to this theorem, each degree of freedom contributes an amount of energy to the total energy of the system.
- According to the equipartition theorem, the energy associated with each degree of freedom is given by the formula:
E=12kT
- Here, k is the Boltzmann constant, and T is the absolute temperature in Kelvin.
- The options provided are:
- (A) 12RT
- (B) 12kT
- (C) 32RT
- (D) 32kT
- From our understanding of the equipartition theorem, the correct expression for the energy associated with each degree of freedom is 12kT.
- Based on the analysis, the correct answer is option (B) 12kT.
The energy associated with each degree of freedom of a molecule is 12kT.
Topper's Solved these Questions
Similar Questions
Knowledge Check
According to the law of equipartition of energy, the energy associated with each degree of freedom is :
AE=kBTBE=12kBTCE=3kBTDE=32kBT
What are degrees of freedom?
View SolutionFind the molar mass and the number of degrees of freedom of molecules in a gas if its heat capacities are Cv=650Jkg−1K−1 and CP=910Jkg−1K−1
View SolutionIf γ be the ratio of specific heats (Cp&Cv) for a perfect gas. Find the number of degrees of freedom of a molecules of the gas?
View SolutionBond energy of a molecule :
View SolutionThe degrees of freedom of a triatomic gas is
View SolutionThe ratio (CpCv) for a gas is 1.29 . What is the degree of freedom of the molecules of this gas?
View SolutionThe total energy of molecules is divided equally amongst the various degrees of freedom of a molecule. The distribution of kinetic energy along x, y, z axis are EKx,EKy,EKz
Total K.e =EKx+EKy+EKz
Since the motion of molecule is equally probable in all the three directions, therefore
EKx=EKy=EKz=13EK=13×32kT=12kT, where k=RNA = Botzman constant.
K.E.=12kT per molecule or =12RT per mole.
In vibration motion, molecules possess both kinetic energy as well as potential energy. This means energy of vibration involves two degrees of fiuedom.
Vibration energy =2×12kT=2×12RT [∴ two degrees of freedom per mole]
If the gas molecules have n1 translational degrees of freedom, n2 rotational degrees of freedom and n3 vibrational degrees of freedom, that total energy = n1[kT2]+n2[kT2]+n3[kT2]×2
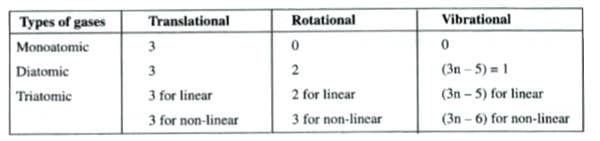
Where 'n' is atomicity of gas.
How many total degrees of freedom are present in H2 molecules in all types of motions ?
View Solution
