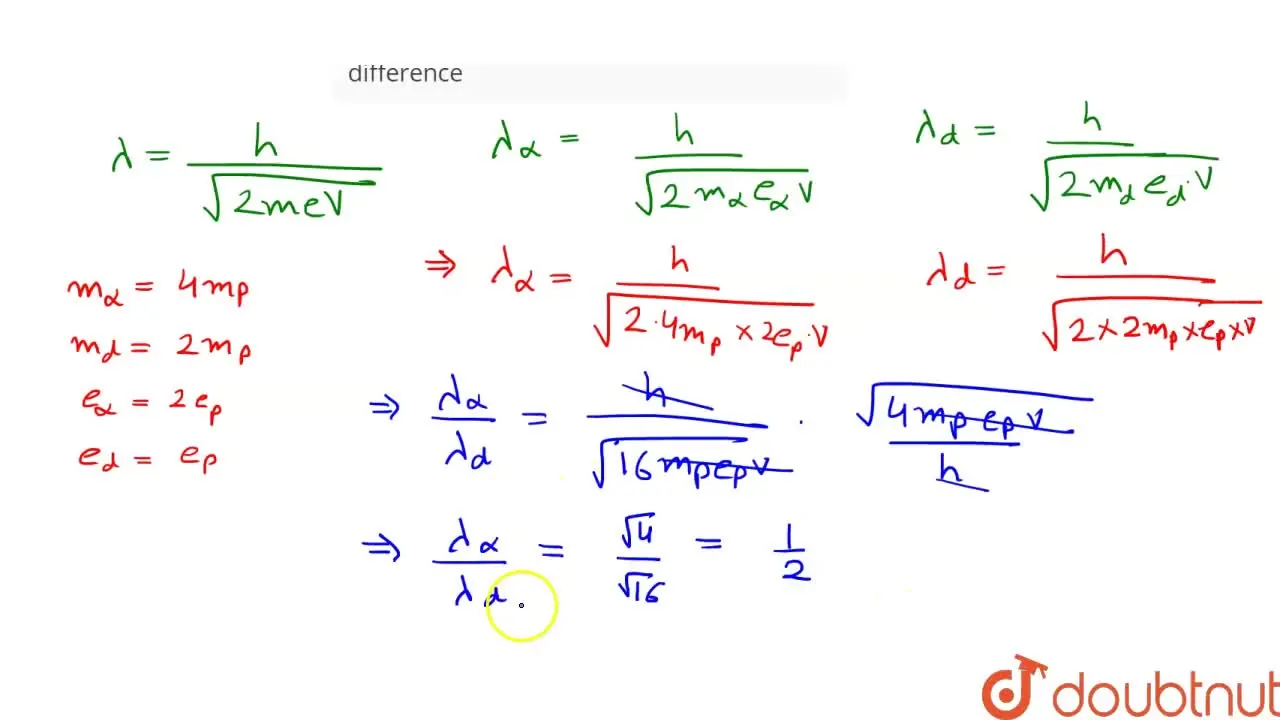
Find the ratio of de Broglie wavelengths of an α -perticle and a deutron it they are accelearating through the sam potenital difference .
1
√2
1/√2
none of the above
The correct Answer is:A
λPλN=(hmPVP)(hmNVN)=mNmP
(∵VP=VN)=1
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.209videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.2120videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMEXERCISE - DPP No.189videos
DAILY PRACTICE PROBLEM
- BOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - High Level Problems (HIP)19videos
CURRENT ELECTRICITY
- BOOK - RESONANCE ENGLISHCHAPTER - ELECTRO MAGNETIC WAVESEXERCISE - Exercise 327videos
ELECTRO MAGNETIC WAVES
Similar Questions
Find the ratio of de Broglie wavelength of an α -particle and a deutron if they are accelerating through the same potential difference
View SolutionFind the ratio of de Broglie wavelength of a proton and as α-particle which have been accelerated through same potential difference.
View SolutionThe ratio of de Broglie wavelengths of a proton and a neutron moving with the same velocity is nearly-
View SolutionThe de Broglie wavelength is given by
View Solutionde-Broglie wavelength applies only to
View SolutionThe ratio of the de Broglie wavelength of a proton and alpha particles will be 1:2 if their
View SolutionThe ratio of the de Broglie wavelength of a proton and alpha particles will be 1:2 if their
View SolutionWhat will be the ratio of de - Broglie wavelengths of proton and α - particle of same energy ?
View SolutionThe de Broglie wavelength associated with particle is
View SolutionMention the significance of Davisson-Germer experiment. An α-particle and a proton are accelerated from rest through the same potential difference V. Find the ratio of de-Broglie wavelengths associated with them.
View Solution