Particle 1 experiences a perfectly elastic collision with a stationary particle 2. Determine their mass ratio, if
(a) after a head-on collision the particles fly apart in the opposite directions with equal velocities,
(b) the particles fly apart symmetrically relative to the initial motion direction of particle I with the angle of divergence θ=60∘.
The correct Answer is:m2m1=3
To solve the problem, we will break it down into two parts as given in the question.
Part (a): Head-on Collision with Equal Velocities
1. Understanding the Scenario:
- Particle 1 (mass m1) is moving with an initial velocity u1.
- Particle 2 (mass m2) is stationary (velocity = 0).
- After the collision, both particles move apart with equal velocities v in opposite directions.
2. Applying Conservation of Momentum:
- Before the collision, the total momentum is:
Pinitial=m1u1+m2⋅0=m1u1
- After the collision, the total momentum is:
Pfinal=m1(−v)+m2v=−m1v+m2v
- According to the conservation of momentum:
m1u1=m2v−m1v
- Rearranging gives us:
m1u1=v(m2−m1)(Equation 1)
3. Applying Conservation of Kinetic Energy:
- The initial kinetic energy is:
KEinitial=12m1u21
- The final kinetic energy is:
KEfinal=12m1v2+12m2v2
- According to the conservation of kinetic energy:
12m1u21=12m1v2+12m2v2
- Simplifying gives us:
m1u21=m1v2+m2v2(Equation 2)
4. Substituting Equation 1 into Equation 2:
- From Equation 1, we can express u1 as:
u1=v(m2−m1)m1
- Substituting this into Equation 2:
m1(v(m2−m1)m1)2=m1v2+m2v2
- This leads to:
v2(m2−m1)2m1=m1v2+m2v2
- Dividing through by v2 (assuming v≠0):
(m2−m1)2m1=m1+m2
- Rearranging gives:
m22−2m1m2+m21=m21+m1m2
- Simplifying leads to:
m22−3m1m2=0
- Factoring out m2:
m2(m2−3m1)=0
- Thus, m2=3m1 or m2=0 (not physically meaningful). Therefore, the mass ratio is:
m2m1=3orm1m2=13
Part (b): Collision with Angle of Divergence θ=60∘
1. Understanding the Scenario:
- After the collision, particles fly apart symmetrically with an angle of 60∘.
- Each particle moves at an angle of 30∘ relative to the initial direction of particle 1.
2. Applying Conservation of Momentum:
- In the horizontal direction:
m1u1=m1v1cos(30∘)+m2v2cos(30∘)(Equation 1)
- In the vertical direction (initially zero):
0=m1v1sin(30∘)−m2v2sin(30∘)(Equation 2)
3. Using the Kinetic Energy Conservation:
- Initial kinetic energy:
12m1u21
- Final kinetic energy:
12m1v21+12m2v22(Equation 3)
4. Solving the Equations:
- From Equation 2:
m1v1sin(30∘)=m2v2sin(30∘
m1v1=m2v2
- From Equation 1:
m1u1=m1v1cos(30∘)+m2v2cos(30∘
- Substituting v2=m1v1m2 into the momentum equation:
m1u1=m1v1cos(30∘)+m2(m1v1m2)cos(30∘
- This simplifies to:
m1u1=m1v1cos(30∘)+m1v1cos(30∘
- Thus:
u1=2v1cos(30∘)(Equation 4)
5. Substituting into Kinetic Energy Equation:
- Substitute u1 from Equation 4 into Equation 3:
12m1(2v1cos(30∘))2=12m1v21+12m2v22
- Simplifying gives:
2m1v21cos2(30∘)=m1v21+m2(m1v1m2)2
- Rearranging leads to:
2cos2(30∘)=1+m1m2
- Knowing cos(30∘)=√32:
2(34)=1+m1m2
- Thus:
32=1+m1m2
- Rearranging gives:
m1m2=12
Final Results:
- For part (a): The mass ratio is m1m2=13.
- For part (b): The mass ratio is m1m2=2.
- Particle 1 (mass m1) is moving with an initial velocity u1.
- Particle 2 (mass m2) is stationary (velocity = 0).
- After the collision, both particles move apart with equal velocities v in opposite directions.
- Before the collision, the total momentum is:
Pinitial=m1u1+m2⋅0=m1u1
- After the collision, the total momentum is:
Pfinal=m1(−v)+m2v=−m1v+m2v
- According to the conservation of momentum:
m1u1=m2v−m1v
- Rearranging gives us:
m1u1=v(m2−m1)(Equation 1)
- The initial kinetic energy is:
KEinitial=12m1u21
- The final kinetic energy is:
KEfinal=12m1v2+12m2v2
- According to the conservation of kinetic energy:
12m1u21=12m1v2+12m2v2
- Simplifying gives us:
m1u21=m1v2+m2v2(Equation 2)
- From Equation 1, we can express u1 as:
u1=v(m2−m1)m1
- Substituting this into Equation 2:
m1(v(m2−m1)m1)2=m1v2+m2v2
- This leads to:
v2(m2−m1)2m1=m1v2+m2v2
- Dividing through by v2 (assuming v≠0):
(m2−m1)2m1=m1+m2
- Rearranging gives:
m22−2m1m2+m21=m21+m1m2
- Simplifying leads to:
m22−3m1m2=0
- Factoring out m2:
m2(m2−3m1)=0
- Thus, m2=3m1 or m2=0 (not physically meaningful). Therefore, the mass ratio is:
m2m1=3orm1m2=13
- After the collision, particles fly apart symmetrically with an angle of 60∘.
- Each particle moves at an angle of 30∘ relative to the initial direction of particle 1.
- In the horizontal direction:
m1u1=m1v1cos(30∘)+m2v2cos(30∘)(Equation 1)
- In the vertical direction (initially zero):
0=m1v1sin(30∘)−m2v2sin(30∘)(Equation 2)
- Initial kinetic energy:
12m1u21
- Final kinetic energy:
12m1v21+12m2v22(Equation 3)
- From Equation 2:
m1v1sin(30∘)=m2v2sin(30∘
m1v1=m2v2
- From Equation 1:
m1u1=m1v1cos(30∘)+m2v2cos(30∘
- Substituting v2=m1v1m2 into the momentum equation:
m1u1=m1v1cos(30∘)+m2(m1v1m2)cos(30∘
- This simplifies to:
m1u1=m1v1cos(30∘)+m1v1cos(30∘
- Thus:
u1=2v1cos(30∘)(Equation 4)
- Substitute u1 from Equation 4 into Equation 3:
12m1(2v1cos(30∘))2=12m1v21+12m2v22
- Simplifying gives:
2m1v21cos2(30∘)=m1v21+m2(m1v1m2)2
- Rearranging leads to:
2cos2(30∘)=1+m1m2
- Knowing cos(30∘)=√32:
2(34)=1+m1m2
- Thus:
32=1+m1m2
- Rearranging gives:
m1m2=12
- For part (a): The mass ratio is m1m2=13.
- For part (b): The mass ratio is m1m2=2.
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 78
3 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 79
4 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 76
5 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
53 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
A particle of mass m1 experiences a perfectlly elastic collision with a stationary particle of mass m2. Match the entries in column I to all the entries in column II.
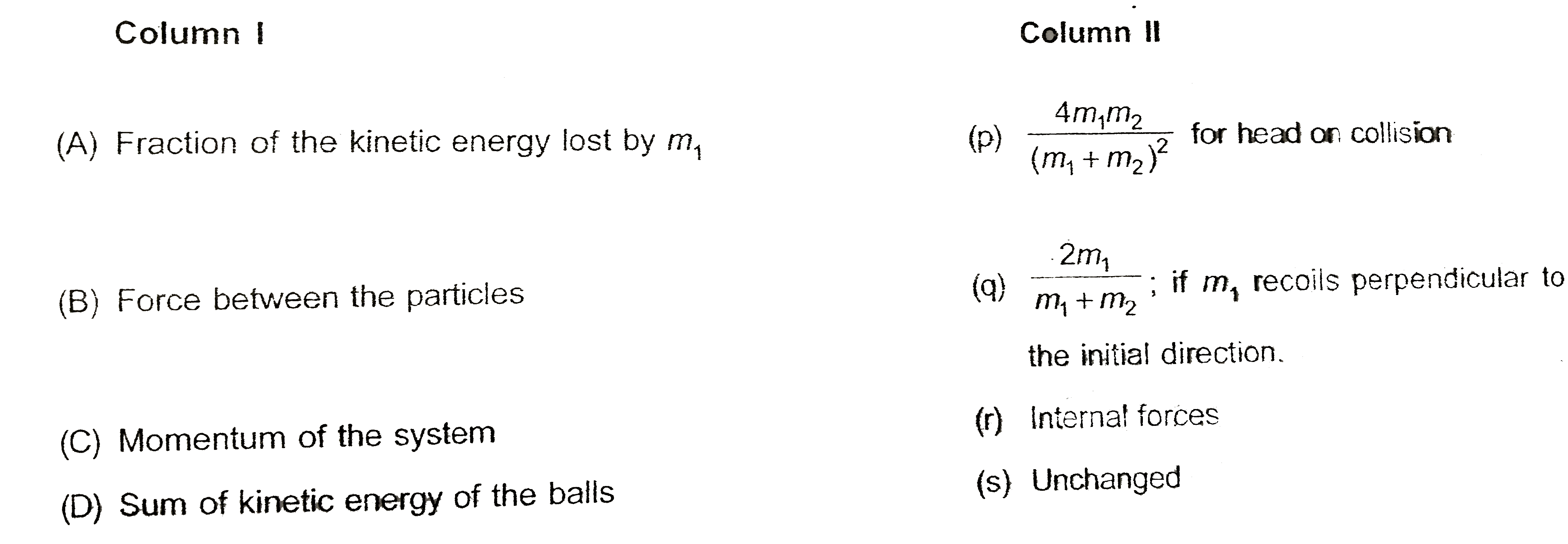
View SolutionParticle A makes a head on elastic collision with another stationary particle B. They fly apart in opposite directions with equal speeds. The mass ratio will be
View SolutionParticle A makes a perfectly elastic collision with anther particle B at rest. They fly apart in opposite direction with equal speeds. If the masses are mA&mB respectively, then
View SolutionParticle A makes a perfectly elastic collision with anther particle B at rest. They fly apart in opposite direction with equal speeds. If the masses are mA&mB respectively, then
View SolutionA particle A suffers an oblique elastic collision particle B that is at rest initially. If their masses with a are the same, then after the collision
View SolutionA particle of mass m moving with speed V collides elastically with another particle of mass 2m. Find speed of smaller mass after head on collision
View SolutionA particle of mass m collides with another stationary particle of mass M such that the second particle starts moving and the first particle stops just after the collision. Then which of the following conditions must always be valid ?
View SolutionA particle strikes elastically with another particle with velocity V after collision its move with half the velocity in the same direction find the velocity of the second particle if it is initially at rest
View SolutionA particle of mass m moving with velocity v makes head-on elastic collision with a sationary particle of mass 2m. Kinetic energy lost by the lighter particle during period of deformation is
View SolutionA particle moving with kinetic energy =3J makes an elastic head-on collision with a stationary particle which has twice its mass. During the impact :-
View Solution
