A chain is held on a frictionless talbe with L/4 hanging over. Knowing total mass of the chain is M and total length is L, the work required to slowly pull hanging part back to the table is :
MgL16
MgL8
MgL32
MgL24
The correct Answer is:C
To solve the problem of calculating the work required to slowly pull the hanging part of the chain back to the table, we can follow these steps:
Step 1: Identify the mass of the hanging part of the chain
The total mass of the chain is M and the total length of the chain is L. Since L4 of the chain is hanging, the mass of the hanging part can be calculated as follows:
Mass of hanging part=ML×L4=M4
Step 2: Calculate the gravitational force on the hanging part
The gravitational force acting on the hanging part of the chain is given by the product of its mass and the acceleration due to gravity g:
Gravitational force=Mass×g=(M4)g=Mg4
Step 3: Determine the center of mass of the hanging part
The center of mass of the hanging part, which is L4 long, is located at its midpoint. Therefore, the distance from the table to the center of mass is:
Distance to center of mass=L4÷2=L8
Step 4: Calculate the work done to lift the hanging part
The work done W against gravity to lift the hanging part back to the table can be calculated using the formula:
W=Force×Distance
Since the force is acting in the opposite direction of the displacement (lifting the chain), we take the angle between force and displacement as 180 degrees, thus:
W=(Mg4)×(L8)×cos(180∘)
Since cos(180∘)=−1:
W=−(Mg4)×(L8)=−MgL32
However, we are interested in the magnitude of work done, so we take the positive value:
W=MgL32
Final Answer
The work required to slowly pull the hanging part back to the table is:
MgL32
The total mass of the chain is M and the total length of the chain is L. Since L4 of the chain is hanging, the mass of the hanging part can be calculated as follows:
The gravitational force acting on the hanging part of the chain is given by the product of its mass and the acceleration due to gravity g:
The center of mass of the hanging part, which is L4 long, is located at its midpoint. Therefore, the distance from the table to the center of mass is:
The work done W against gravity to lift the hanging part back to the table can be calculated using the formula:
The work required to slowly pull the hanging part back to the table is:
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - Dpp 37
8 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - dpp 38
5 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - DPP 35
5 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
53 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
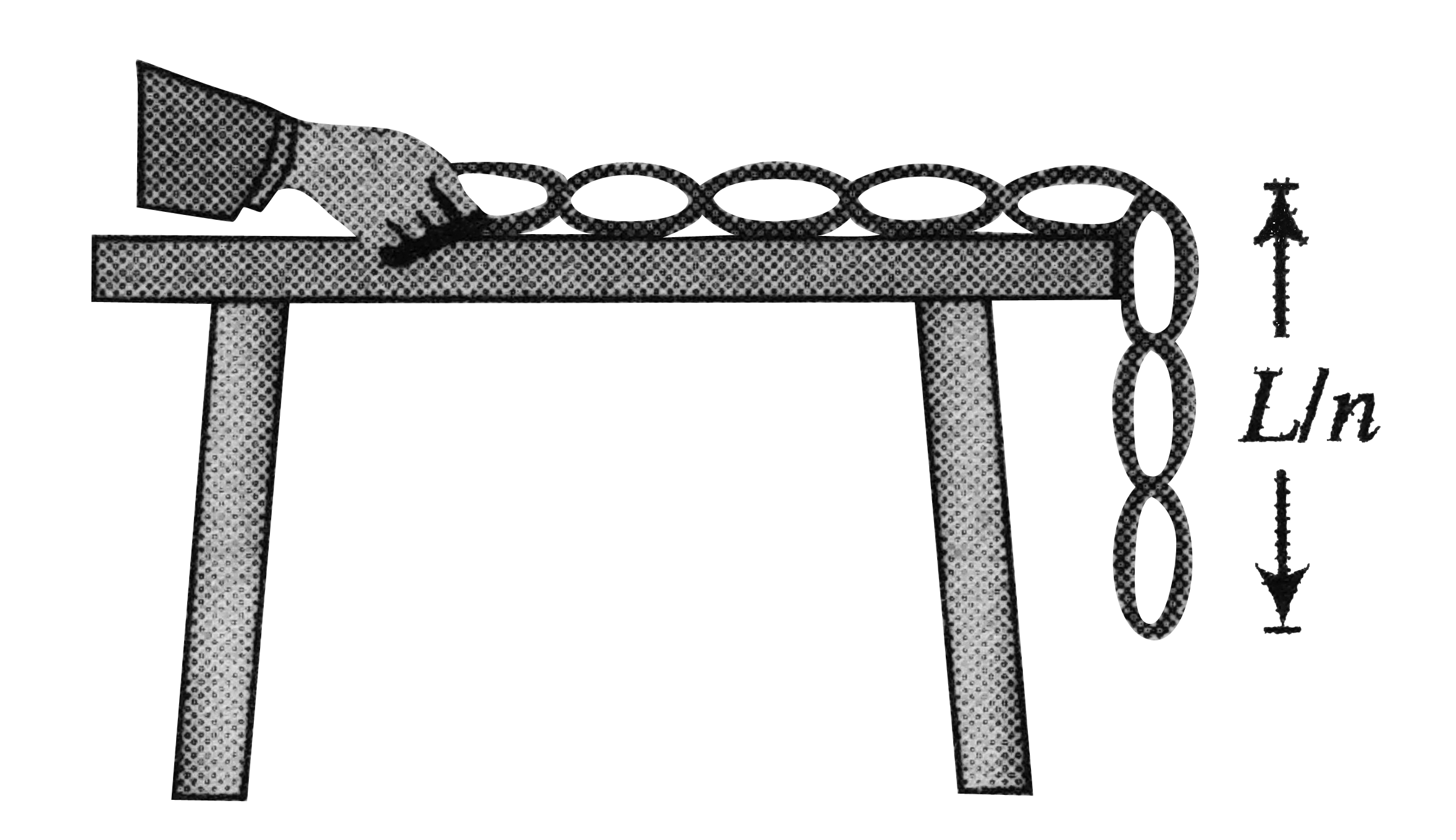
A chain is held on a frictionless table with 1/n th of its length hanging over the edge. If the chain has a length L and a mass M, how much work is required to pull the hanging part back on the table?
View SolutionA chain of length L and mass M is held on a frictionless table with (1/n)th of its length hanging over the edge. When the chain is released, find the velocity of chain while leaving the table.
View Solution
Knowledge Check
A uniform chain of length L and mass M is lying on a smooth table and one-third of its length is hanging vertically down over the edge of the table. If g is the acceleration due to gravity, the work required to pull the hanging part on to the table is
AMgLBMgL3CMgL9DMgL18A chain of uniform mass m and length L is held on a frictionless table in such a way that its 1nth part is hanging below the edge of table. The work done to pull the hanging part of chain is :−
A√nBnCn−3Dn−2A uniform chain of length 2m is kept on a table such that a length of 60cm hangs freely from the edge of the table . The total mass of the chain is 4kg What is the work done in pulling the entire the chain the on the table ?
A12.9JB6.3 JC3.6 JD2.0 J
A uniform chain of mass m & length L is kept on a smooth horizontal table such that Lnportion of the chaing hangs from the table. The work dione required to slowly bringsthe chain completely on the table is
View SolutionA uniform chain of length 4m is kept on a table such that a length of 120 cm hangs freely from the edge of the table. The total mass of the chain is 4 kg What is the work done in pulling the entire chain on the table ?
View SolutionA uniform chain has a mass M and length L. It is placed on a frictionless table with length l0 hanging over the edge. The chain begins to slide down. Ten, the speed v with which the end slides down from the edge is given by
View SolutionOne fourth chain is hanging down from a table work done to bring the hanging part of the chain on the table is ( mass of chain =M and length = L)
View SolutionA uniform chain of mass m and length l overhangs a table with its two third part on the table. Fine the work to be done by a person to put the hanging part back on the table.
View Solution
