Find the magnitude of the unknown forces X and Y if sum of all forces is zero.

The correct Answer is:(5,10)
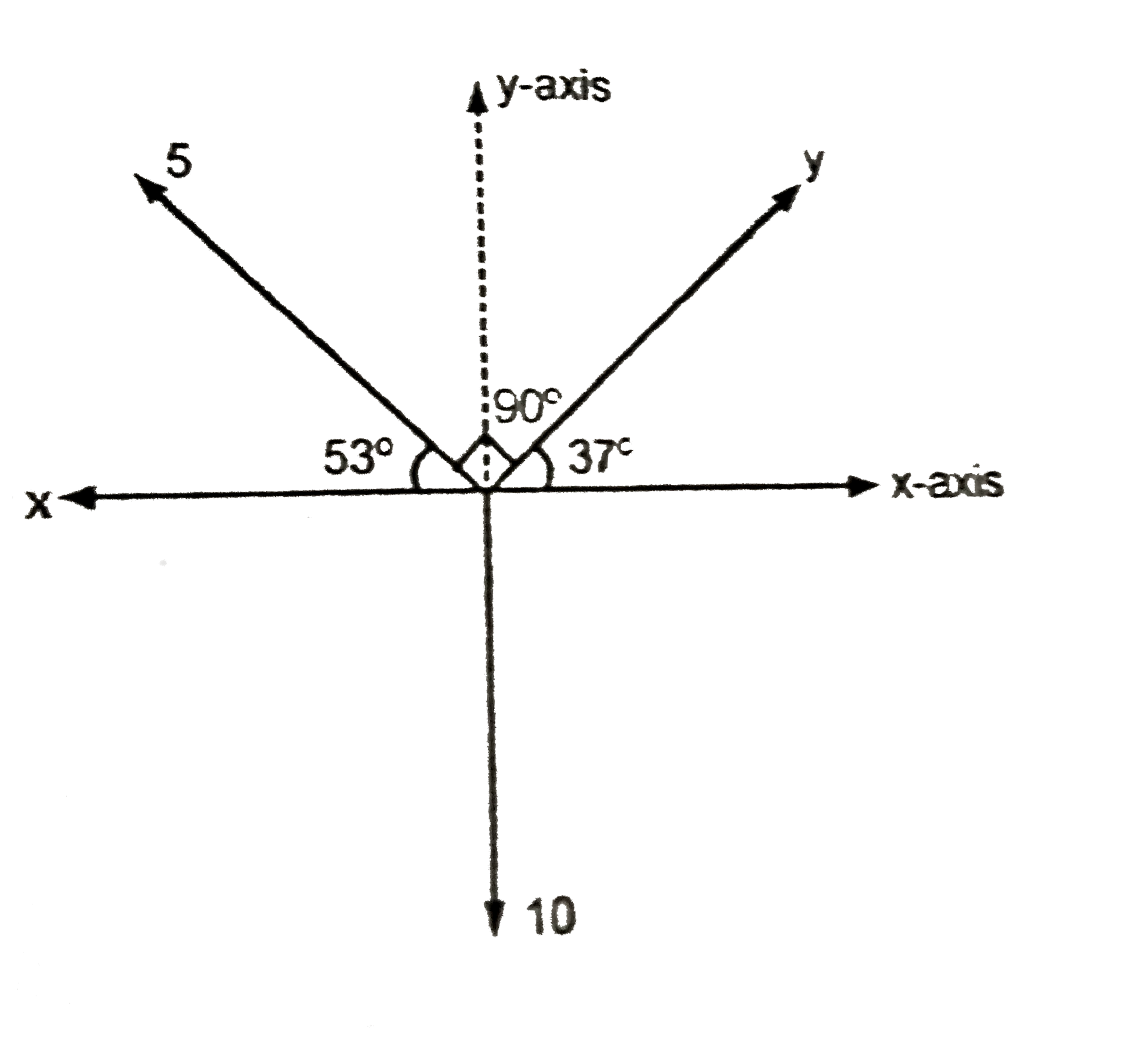
Σ→F=0
⇒(ycos37∘ˆi+ysin37∘ˆj)+(5cos53∘(−ˆi)+5sin53∘ˆj)+(x(−ˆi))+10(−ˆj))=0
⇒(4y5−3−x)ˆi+(3y5+4−10)ˆj=0ˆi+0ˆj
Comparting coefficients of ˆi&ˆj both sided - 4y5−x=3 .......(i)
3y5=6⇒y=10
Putting 8−x=3⇒x=5
Topper's Solved these Questions
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - Dpp no 10 physics
8 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - Dpp no 11 physics
6 Videos
- DAILY PRACTICE PROBLEMSBOOK - RESONANCE ENGLISHCHAPTER - DAILY PRACTICE PROBLEMSEXERCISE - DPP 8 PHYSICS
7 Videos
- CURRENT ELECTRICITYBOOK - RESONANCE ENGLISHCHAPTER - CURRENT ELECTRICITYEXERCISE - Exercise
53 Videos
- ELASTICITY AND VISCOCITYBOOK - RESONANCE ENGLISHCHAPTER - ELASTICITY AND VISCOCITYEXERCISE - Advanced Level Problems
9 Videos
Similar Questions
Find the magnitudes of the unknown forces if the sum of all forces is zero (figure)

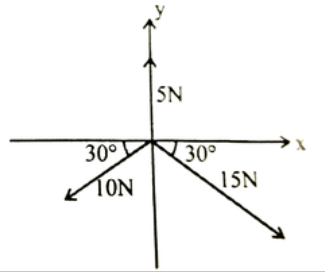
View SolutionFind the magnitude of the resultant of shown forces :-
View SolutionFind the values of the unknowns x and y in the following diagrams

View SolutionTwo forces with equal magnitudes F act on a body and the magnitude of the resultant force is F /3. The angle between the two forces is
View SolutionTwo forces of magnitudes P and Q are inclined at an angle (θ). The magnitude of their resultant is 3Q. When the inclination is changed to (180∘−θ), the magnitude of the resultant force becomes Q. Find the ratio of the forces.
View SolutionLet F,FNandf denote the magnitudes of the contact force, normal force and the friction exerted by one surface on the other kept in contact. If none of these is zero,
View SolutionAssertion: Magnitude of the contact force is always greater than the magnitude of frictional force.
Reason: Contact force is the resultant of the friction force and normal reaction.
View SolutionThe sum of the magnitudes of two forces acting at a point is 18 and the magnitude of their resultant is 12. If the resultant is at 90∘ with the force of smaller magnitude, What are the magnitudes of forces?
View SolutionThe sum of the magnitudes of two forces acting at a point is 18 and the magnitude of their resultant is 12. If the resultant is at 90∘ with the force of smaller magnitude, What are the magnitudes of forces?
View SolutionThe sum of magnitudes of two forces acting at a point is 16N. If the resultant force is 8N and its direction is perpendicular to smaller force, then the forces are :-
View Solution
