A polytropic process for an ideal gas is represented by equation PVn=constant. If g is ratio of specific heats (CpCv), then value of n for which molar heat capacity of the process is negative is given as
γ>n
γ>n>1
n>γ
none, as it is not possible
The correct Answer is:B
To solve the problem, we need to find the value of n for which the molar heat capacity of a polytropic process becomes negative. The polytropic process is defined by the equation:
PVn=constant
1. Understanding the Polytropic Process:
The equation PVn=K (where K is a constant) describes a polytropic process. The value of n characterizes the type of process (isothermal, adiabatic, etc.).
Hint: Recall the definitions of different thermodynamic processes and how they relate to the value of n.
2. Applying the First Law of Thermodynamics:
According to the first law of thermodynamics, the heat added to the system Q is equal to the change in internal energy ΔU plus the work done W:
Q=ΔU+W
3. Expressing Heat and Work:
The heat Q can be expressed as:
Q=mCΔT
where C is the molar heat capacity. The change in internal energy for an ideal gas is:
ΔU=mCVΔT
The work done during a polytropic process can be expressed as:
W=PΔV
4. Substituting for Work:
From the equation PVn=K, we can express P as:
P=KVn
Substituting this into the work equation gives:
W=KVnΔV
5. Combining Equations:
Substituting Q, ΔU, and W into the first law gives:
mCΔT=mCVΔT+KVnΔV
6. Rearranging the Equation:
Rearranging the equation leads to:
C=CV+KmVnΔTΔV
7. Finding Molar Heat Capacity:
Using the ideal gas law PV=nRT, we can express K in terms of R and γ:
C=CV+R1−n
8. Setting Condition for Negative Heat Capacity:
For the molar heat capacity C to be negative, we need:
C<0⟹CV+R1−n<0
Rearranging gives:
R1−n<−CV
9. Using the Relation of Specific Heats:
We know that:
CV=Rγ−1
Substituting this into the inequality gives:
R1−n<−Rγ−1
10. Solving for n:
Simplifying leads to:
1−n<−(γ−1)
Which simplifies to:
n<γ
Thus, for molar heat capacity to be negative, we need:
n<γ
Final Answer: The value of n for which the molar heat capacity of the process is negative is given by n<γ.
---
The equation PVn=K (where K is a constant) describes a polytropic process. The value of n characterizes the type of process (isothermal, adiabatic, etc.).
According to the first law of thermodynamics, the heat added to the system Q is equal to the change in internal energy ΔU plus the work done W:
The heat Q can be expressed as:
From the equation PVn=K, we can express P as:
Substituting Q, ΔU, and W into the first law gives:
Rearranging the equation leads to:
Using the ideal gas law PV=nRT, we can express K in terms of R and γ:
For the molar heat capacity C to be negative, we need:
We know that:
Simplifying leads to:
Topper's Solved these Questions
- KTG & THERMODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - Exercise-2
1 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - PART -I
15 Videos
- KTG & THERMODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - PART -II
17 Videos
- KINETIC THEORY OF GASES AND THERMODYNAMICSBOOK - RESONANCE ENGLISHCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Exercise
64 Videos
- MAGNETIC FIELD AND FORCESBOOK - RESONANCE ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Exercise
64 Videos
Similar Questions
If molar heat capacity of the given process (as shown in figure) is C, then

View SolutionFind the value of molar heat capacity for an ideal gas in an adiabatic process.
View SolutionThe molar heat capacity for the process shown in fig. is

View SolutionAn ideal has undergoes a polytropic given by equation PVn = constant. If molar heat capacity of gas during this process is arithmetic mean of its molar heat capacity at constant pressure and constant volume then value of n is
View SolutionOne mole of an ideal gas undergoes a process such that P∝1T. The molar heat capacity of this process is 4R.
View SolutionP-V graph for an ideal gas undergoing polytropic process PVm=constant is shown here. Find the value of m.
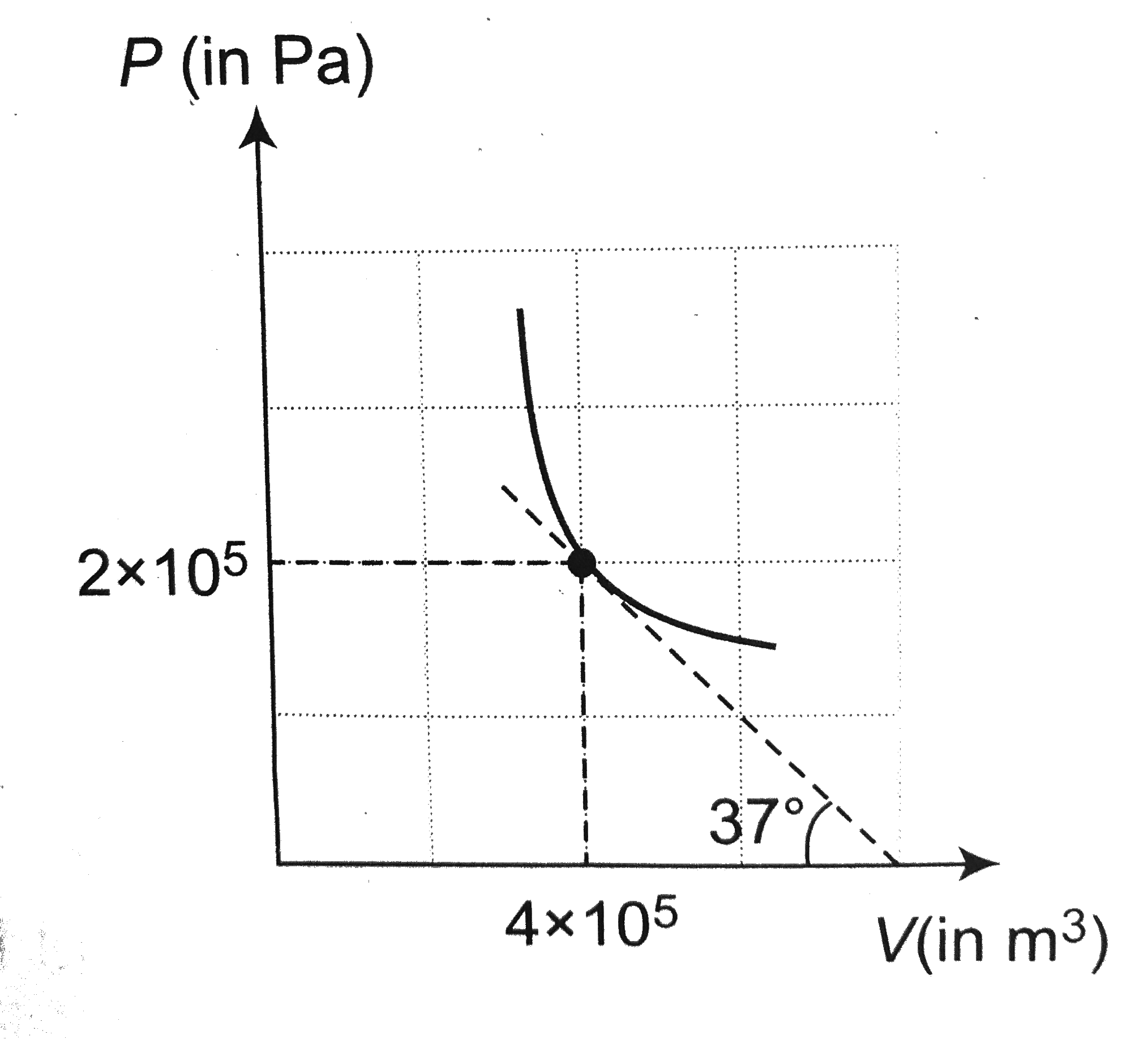
View SolutionOne mole of an ideal monatomic gas undergoes a process described by the equation PV3= constant. The heat capacity of the gas during this process is
View SolutionA diatomic gas obeys the law pVx= constant. For what value of x, it has negative molar specific heat?
View SolutionA diatomic ideal gas is expanded according to PV3 = constant, under very high temperature (Assume vibration mode active). Calculate the molar heat capacity of gas (in cal / mol K) in this process.
View SolutionA cyclic process ABCD is shown in the P−V diagram. Which of the following curves represents the same process?
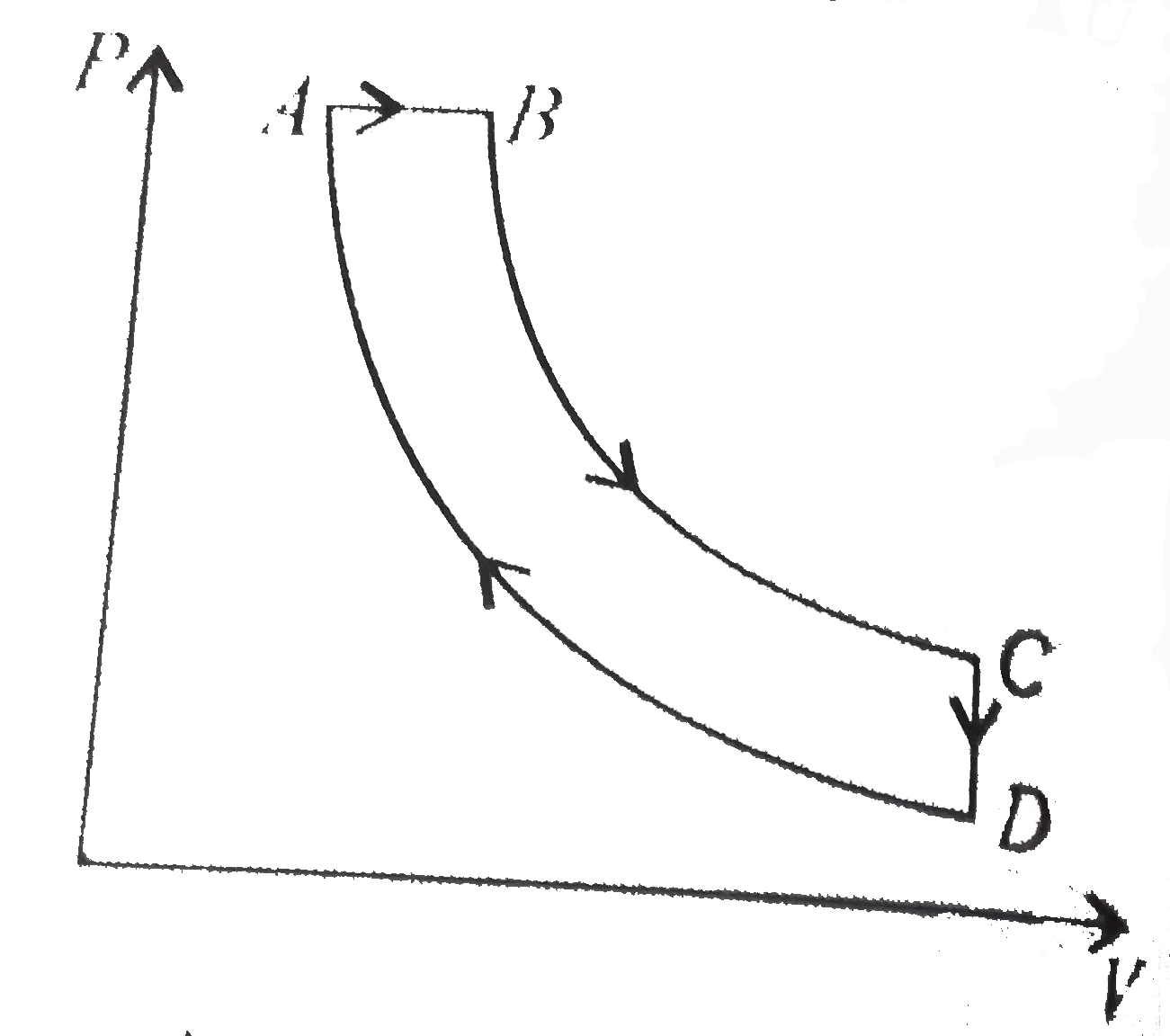
View Solution
