Find the average momentum of molecules of hydrogen gas in a container at temperature 300K.
The correct Answer is:Zero
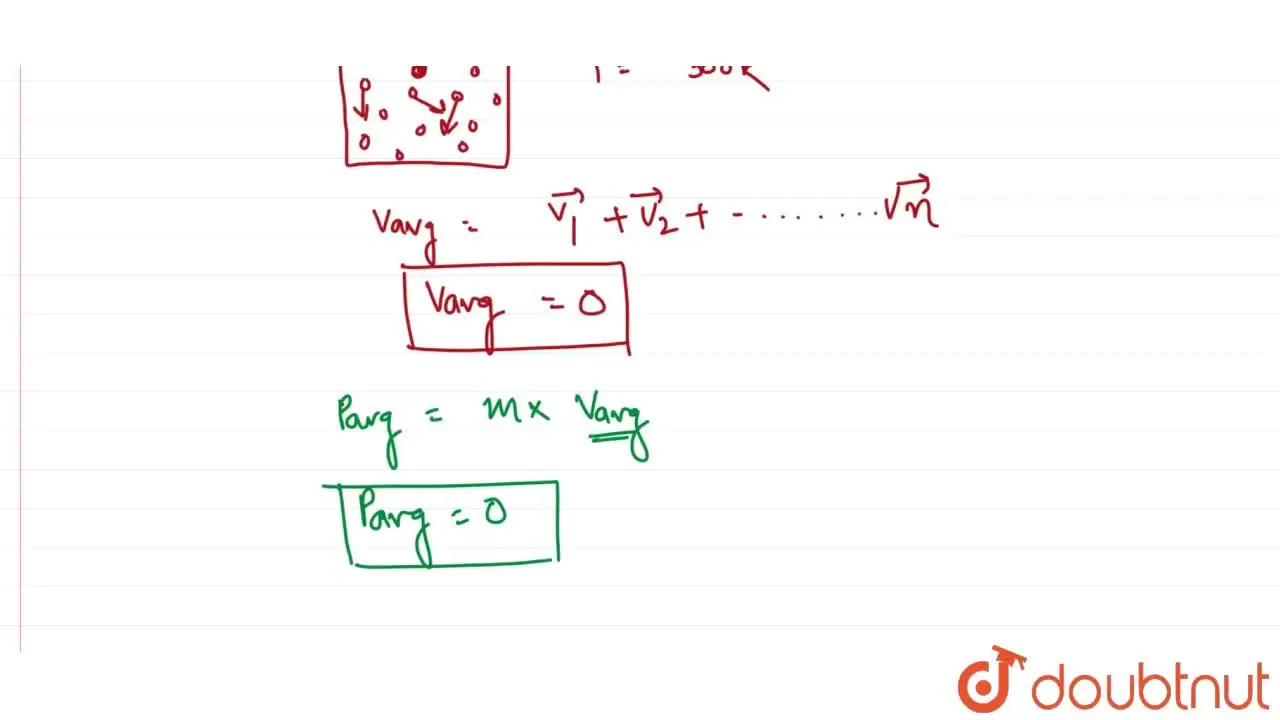
Average momentum of molecule of gas
→Pav=M→Vav
[→Vav=0 Average velocity of gas molecules at any temperature is always zero]
Hence, →Pav=0
Related Playlists
- BOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (D)2videos
KTG & THERMODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (I)2videos
KTG & THERMODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - KTG & THERMODYNAMICSEXERCISE - SECTION (H)3videos
KTG & THERMODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Exercise64videos
KINETIC THEORY OF GASES AND THERMODYNAMICS
- BOOK - RESONANCE ENGLISHCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Exercise64videos
MAGNETIC FIELD AND FORCES
Similar Questions
If the average kinetic energy of a molecule of hydrogen gas at 300 K is E , then the average kinetic energy of a molecule of nitrogen gas at the same temperature is
View SolutionSolve
A vessel contains X number of molecules of hydrogen gas at a certain temperature and pressure. How many molecules of nitrogen gas would be present in the same vessel under the same conditions of temperature and pressure ?View SolutionThe average velocity of molecules of a gas of molecular weight (M) at temperature (T) is
View SolutionA vessel contains X number of molecule of hydrogen gas at a certain temperature and pressure. How many molecules of oxygen will be present in a vessel of same volume under the same conditions of temperature and pressure? Name the law to justify your answer.
View SolutionFind the average of magnitude of linear momentum of helium molecules in a sample of helium gas at temperature of 150πK. Mass of a helium molecules =(166/3)×10−27kg and R=25/3J−mol−1K−1
View SolutionThe average energy per molecule of a triatomic gas at room temperature T is
View SolutionConsider a sample fo oxygen at 300K. Find the average time taken by a molecule to travel a distance equal to the diameter of the earth.
View SolutionThe avrage kinetic energy of molecules in a gas at temperature T is 1.5KTfind the temperature at which the average kinetic energy of the molecules of hydrogen equals the binding energy of its atoms will hydrogen remain in molecles form at this temperature ? Take k=8.62×10−5eVK−1
View SolutionThe temperature at which the average speed of the gas molecules is double to that at a temperature of 27∘C is
View SolutionFind the rms speed of hydrogen molecules at room temperature (=300K).
View Solution