A body is executing SHM under the action of force whose maximum magnitude is 5√2N. The magnitude of force acting on the particle at the instant when its kintentic energy and potential are euqal is 10x. Find x. (Assume potenital energy to be zero at mean position)
The correct Answer is:5
To solve the problem step by step, we need to analyze the given information about a body executing Simple Harmonic Motion (SHM).
Step 1: Understand the Maximum Force in SHM
The maximum force Fmax in SHM is given by:
Fmax=mω2A
where A is the amplitude of the motion. We are given that Fmax=5√2N.
Step 2: Relate Kinetic Energy and Potential Energy
In SHM, when the kinetic energy (KE) is equal to the potential energy (PE), we can express them as:
KE=12mv2
PE=12kx2
where k=mω2 and x is the displacement from the mean position.
Since KE=PE, we can set the equations equal:
12mv2=12kx2
This simplifies to:
mv2=kx2
Step 3: Express Velocity in Terms of Amplitude
In SHM, the velocity v can be expressed as:
v=ω√A2−x2
Substituting this into the kinetic energy equation gives:
m(ω√A2−x2)2=kx2
Substituting k=mω2:
mω2(A2−x2)=mω2x2
Cancelling mω2 (assuming m≠0 and ω≠0):
A2−x2=x2
This simplifies to:
A2=2x2
Thus, we can express x in terms of A:
x=A√2
Step 4: Calculate the Force at x
The force F at displacement x is given by:
F=−kx=−mω2x
Substituting x=A√2:
F=−mω2(A√2)=−mω2A√2
Step 5: Relate to Given Force
We know that the maximum force Fmax=mω2A=5√2N. Therefore:
F=−5√2√2=−5N
Step 6: Set Up the Equation
According to the problem, the magnitude of the force at this instant is given as 10x:
10x=5
Step 7: Solve for x
From the equation 10x=5:
x=510=0.5
Thus, the value of x is:
0.5
The maximum force Fmax in SHM is given by:
Fmax=mω2A
where A is the amplitude of the motion. We are given that Fmax=5√2N.
In SHM, when the kinetic energy (KE) is equal to the potential energy (PE), we can express them as:
KE=12mv2
PE=12kx2
where k=mω2 and x is the displacement from the mean position.
12mv2=12kx2
This simplifies to:
mv2=kx2
In SHM, the velocity v can be expressed as:
v=ω√A2−x2
Substituting this into the kinetic energy equation gives:
m(ω√A2−x2)2=kx2
Substituting k=mω2:
mω2(A2−x2)=mω2x2
Cancelling mω2 (assuming m≠0 and ω≠0):
A2−x2=x2
This simplifies to:
A2=2x2
Thus, we can express x in terms of A:
x=A√2
The force F at displacement x is given by:
F=−kx=−mω2x
Substituting x=A√2:
F=−mω2(A√2)=−mω2A√2
We know that the maximum force Fmax=mω2A=5√2N. Therefore:
F=−5√2√2=−5N
According to the problem, the magnitude of the force at this instant is given as 10x:
10x=5
From the equation 10x=5:
x=510=0.5
0.5
Topper's Solved these Questions
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PHYSICS
784 Videos
- TEST PAPERSBOOK - RESONANCE ENGLISHCHAPTER - TEST PAPERSEXERCISE - PART - II PHYSICS SEC - 2
20 Videos
- SIMPLE HARMONIC MOTION BOOK - RESONANCE ENGLISHCHAPTER - SIMPLE HARMONIC MOTION EXERCISE - Advanced Level Problems
13 Videos
- TEST SERIESBOOK - RESONANCE ENGLISHCHAPTER - TEST SERIESEXERCISE - PHYSICS
130 Videos
Similar Questions
A body is executing SHM under action of the a force whose maximum magnitude is 50N. The magnitude of force acting on the particle at the time when its energy is half kinetic and half potential energy is (Assume potential energy to be zero at mean position).
View SolutionA particle is executing SHM with amplitude A. At displacement x=(−A4), force acting on the particle is F, potential energy of the particle is U, velocity of particle is v and kinetic energy is K. Assuming potential energyh to be zero at mean position. At displacement x=A/2
View SolutionThe potential energy of a body is given by U=A−Bx2 (where x is the displacement). The magnitude of force acting on the partical is
View SolutionForce acting on a particle is F=−8x in S.H.M. The amplitude of oscillation is 2 (in m) and mass of the particle is 0.5 kg. The total mechanical energy of the particle is 20 J. Find the potential energy of the particle in mean position (in J).
View SolutionThe kinetic energy K of a particle moving along x - axis varies with its position (x) as shown in figure
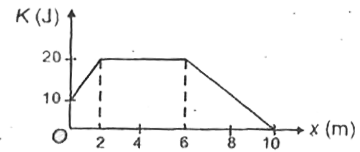
The magnitude of force acting on particle at x = 9 mis
View SolutionIf a body is executing simple harmonic motion and its current displacement is √3/2 times the amplitude from its mean position , then the ratio between potential energy and kinetic energy is
View SolutionA particle executes linear SHM with amplitude A and mean position is x=0. Determine position of the particle where potential energy of the particle is equal to its kinetic energy.
View SolutionThe force on a body executing SHM is 4 N when the displacement from mean position is 2 cm. If amplitude of oscillation is 10 cm, then the maximum kinetic energy associated with the SHM will be
View SolutionA particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View SolutionA particle of mass m and charge −q is released from rest in a uniform electric field. If there is no other force on the particle, the dependence of its potential energy on the distance x travelled by it is correctly given by (assume initial potential energy to be zero ,graphs are schematic and not drawn to scale)
View Solution
