The work done by (x−3) men in (2x+1) days and the work done by (2x+1) men in (x+4) days are in the ratio 3:10. Find the value of x.
The correct Answer is:∴X=6
To solve the problem, we need to find the value of x given that the work done by (x−3) men in (2x+1) days and the work done by (2x+1) men in (x+4) days are in the ratio 3:10.
1. Define the Work Done:
- Let the work done by (x−3) men in (2x+1) days be denoted as W1.
- The work done can be expressed as:
W1=(x−3)×(2x+1)
2. Define the Second Work Done:
- Let the work done by (2x+1) men in (x+4) days be denoted as W2.
- The work done can be expressed as:
W2=(2x+1)×(x+4)
3. Set Up the Ratio:
- According to the problem, the ratio of W1 to W2 is given as:
W1W2=310
- Substituting the expressions for W1 and W2:
(x−3)(2x+1)(2x+1)(x+4)=310
4. Simplify the Equation:
- We can cancel (2x+1) from both sides (assuming 2x+1≠0):
x−3x+4=310
5. Cross Multiply:
- Cross multiplying gives us:
10(x−3)=3(x+4)
6. Expand Both Sides:
- Expanding both sides:
10x−30=3x+12
7. Rearrange the Equation:
- Bringing all terms involving x to one side and constant terms to the other:
10x−3x=12+30
7x=42
8. Solve for x:
- Dividing both sides by 7:
x=427=6
Final Answer:
The value of x is 6.
- Let the work done by (x−3) men in (2x+1) days be denoted as W1.
- The work done can be expressed as:
W1=(x−3)×(2x+1)
- Let the work done by (2x+1) men in (x+4) days be denoted as W2.
- The work done can be expressed as:
W2=(2x+1)×(x+4)
- According to the problem, the ratio of W1 to W2 is given as:
W1W2=310
- Substituting the expressions for W1 and W2:
(x−3)(2x+1)(2x+1)(x+4)=310
- We can cancel (2x+1) from both sides (assuming 2x+1≠0):
x−3x+4=310
- Cross multiplying gives us:
10(x−3)=3(x+4)
- Expanding both sides:
10x−30=3x+12
- Bringing all terms involving x to one side and constant terms to the other:
10x−3x=12+30
7x=42
- Dividing both sides by 7:
x=427=6
The value of x is 6.
Topper's Solved these Questions
- RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)Book:ICSEChapter:RATIO AND PROPORTION (INCLUDING PROPERTIES AND USES)Exercise:Exercise 7(D)
Explore 27 Videos
- RATIO AND PROPORTIONBook:ICSEChapter:RATIO AND PROPORTIONExercise:MULTIPLE CHOICE QUESTION
Explore 61 Videos
- REFLECTION Book:ICSEChapter:REFLECTION Exercise:QUESTION
Explore 1 Video
Similar Questions
The work done by (x−2) men in (4x+1) days and the work done by (4x+1) men in (2x−3) days are in the ratio 3 : 8. Find the value of x.
View SolutionIf (x+3) : (4x+1) is the duplicate ratio of 3:5, find the value of x.
View SolutionIf x=2−√3, find the value of (x−1x)3
View SolutionIf ratio of the work done by n men in (n+2) days is to the work done by n+3 men in n days is (n2+2n+9):(n2+3n+10), then n is equal to
View SolutionThe work done in extending a spring by x0 is W0. Find the work done in further extension x0.
View SolutionA particular work can be completed by 6 men and 6 women in 24 days, whereas the same work can be completed by 8 men and 12 women in 15 days. Find :
The time taken by 4 men and 6 women to complete the same work.
View SolutionIf 32x+1⋅4x−1=36 then find the value of x
View SolutionA particular work can be completed by 6 men and 6 women in 24 days, whereas the same work can be completed by 8 men and 12 women in 15 days. Find :
According to the amount of work done, one man is equivalent to how many women.
View SolutionIf x=√3+12, find the value of 4x3+2x2−8x+7.
View SolutionA force (F) acting on a particle varies work done by a particle varies with the with the position x as shown in figure
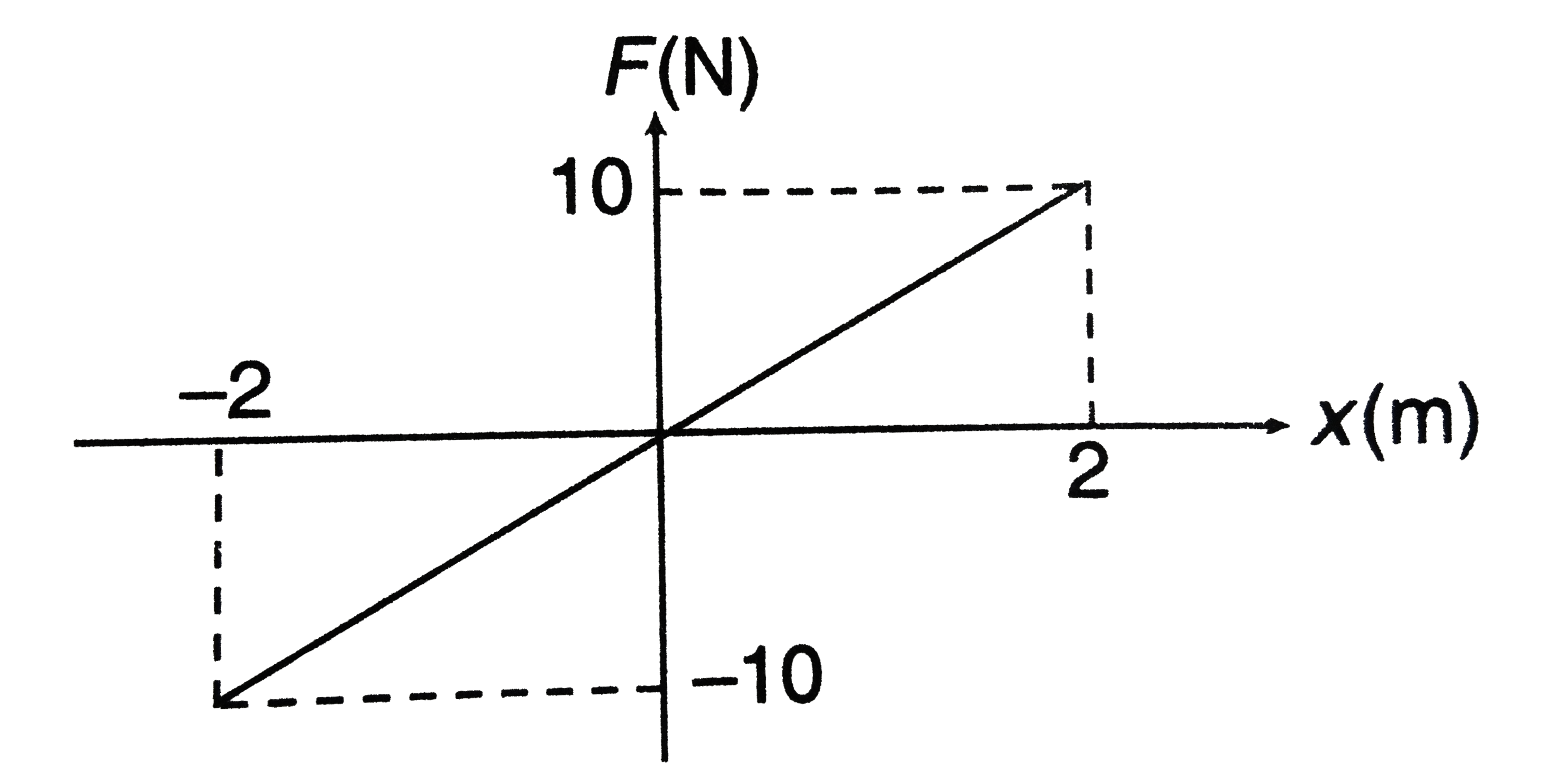 .
.
Find the work done by by force in displacing the particle from .
(a) x=−2m to x=0
(b) x=0 to x=2m..
View Solution