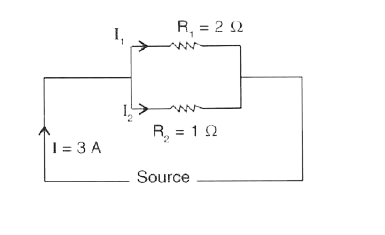
Two resistors of resistance R1=2ΩandR2=1Ω are connected in parallel with a current source of 3 A. Draw the arrangement and deduce the current I1 in R1andI2 in R2.
The diagram alongside shows the arrangement
In parallel,
p.d.across R1= p.d acrosss R2
∴ I1R1=I1R2 (i)
and I=I1+I2 (ii)
On solving equations (i) and (ii)
I1=IR1R1+R2=3×12+1=1A
I2=IR1R1+R2=3×22+1=2A
Topper's Solved these Questions
Similar Questions
Knowledge Check
Two resistors of resistances R1=(300±3)Ω and R2=(500±4)Ω are connected in series. The equivalent resistance of the series combination is
A(800±1)ΩB(800±7)ΩC(200±7)ΩD(200±1)ΩTwo resistances R1=100±3ΩandR2=200±4Ω are connected in series . Find the equivalent resistance of the series combination.
A(66.7±1.8)ΩB(66.7±4.0)ΩC(66.7±3.0)ΩD(66.7±7.0)Ω
Two resistors of resistance R1 and R2 having R1>R2 are connected in parallel. For equivalent resistance R, the correct statement is
View SolutionThree resistors of 1Ω,2Ωand3Ω are connected in parallel. The combined resistance of 3 resistors should be
View SolutionTwo resistors R1=60ΩandR2=90Ω are connected in parallel. If electric power consumed by the resistor R1 is 15 W, calculate the power consumed by the resistor R2
View SolutionTwo resistances R1=100±3ΩandR2=200±4Ω are connected in series . Find the equivalent resistance of the series combination.
View SolutionTwo resistor R1=(24±0.5)ΩandR2=(8±0.3)Ωare joined in series, The equivalent resistence is
View SolutionTwo resistors R1=400ΩandR2=20Ω are connected in parallel to a battery. If heating power developed in R1 is 25. W. find the heating power developed in R2.
View Solution
