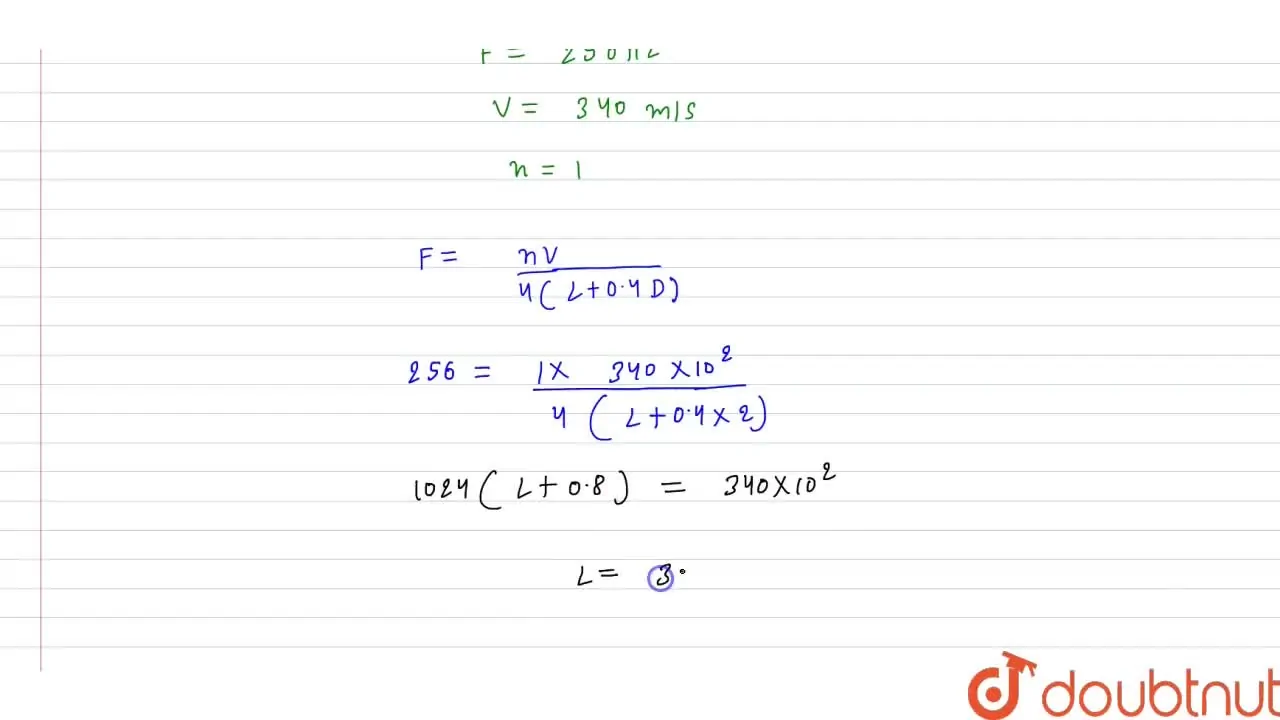
In determination of velocity of sound in air using resonance column tube, radius of cylindrical tube is 1 cm and frequency of tuning fork is 256 Hz. If velocity of sound is 340 m/s then length of air column in the tube when first resonance occurs, is
33.2 cm
32.0 cm
32.6 cm
33.8 cm
Step by step video & image solution for In determination of velocity of sound in air using resonance column tube, radius of cylindrical tube is 1 cm and frequency of tuning fork is 256 Hz. If velocity of sound is 340 m/s then length of air column in the tube when first resonance occurs, is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
In a resonance tube the first resonance with a tuning fork occurs at 16 cm and second at 49 cm . If the velocity of sound is 330 m/s , the frequency of tuning fork is
View SolutionIn an experiment to determine the velocity of sound in air using resonance column apparature, the first and resonating length measured to be 25.4 and 78.5cm respectively. Calculate the velocity of sound in air and the end correction of frequency of the turning fork is 320Hz.
View SolutionIn resonance tube tube successive positions of resonance are obtained at 15 cm and 48 cm . If the frequency of the fork is 500 cps , the velocity of sound is
View SolutionIn the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is 0.1m. When this length is changed to 0.35m, the same tuning fork resonates with the first overtone. Calculate the end correction.
View SolutionIn the experiment for the determination of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fundamental mode, with a tuning fork is 0.1m. When this length is changed to 0.35m, the same tuning fork resonates with the first overtone. Calculate the end correction.
View SolutionThe air column in a pipe closed at one end is made to vibrate in its second overtone by a tuning fork of frequency 440 Hz. The speed of sound in air is 330 m/s. find the length of air column.
View SolutionIn the experinment for the determinnation of the speed of sound in air using the resonance column method, the length of the air column that resonates in the fu ndamental mode , with a tuning fork is 0.1m . When this length is changed to 0.35m , the same tuning fork resonates with the first overtone. Calculate the end correction.
(a) 0.012m
(b) 0.0025m
(c) 0.05m
(d) 0.024mView SolutionWhat is the fundamental frequency of an open organ pipe of length 42.5 cm, if the velocity of sound in air is 340 m/s?
View SolutionIf a resonance tube is sounded with a tuning fork of frequency 256 Hz, resonance occurs at 35 cm and 105 cm. The velocity of sound is about
View SolutionA standing tuning fork of frequency f is used to find the velocity of sound in air by resonance column appartus. The difference two resonating lengths is 1.0m. Then the velocity of sound in air is
View Solution