Calculate the threshold freqency of the metalfrom the photoelectronsare emitted with zero velocity when exposed to radiation of wavelength 6800 Å
Hint :KE=12mv2
hv=hv0+KE,KE=0
v=cλ
To calculate the threshold frequency of the metal from which photoelectrons are emitted with zero velocity when exposed to radiation of wavelength 6800 Å, we can follow these steps:
1. Understand the Concept of Threshold Frequency:
- The threshold frequency (ν₀) is the minimum frequency required to emit a photoelectron from a metal surface. When the energy of the incoming radiation is equal to the work function of the metal, the photoelectron is emitted with zero kinetic energy.
2. Use the Photoelectric Equation:
- The photoelectric equation is given by:
hν = hν₀ + KE
- Since the photoelectrons are emitted with zero velocity, the kinetic energy (KE) is zero. Therefore, the equation simplifies to:
hν=hν₀
- This implies that:
ν=ν₀
3. Calculate the Frequency from Wavelength:
- We are given the wavelength (λ) of the radiation, which is 6800 Å. First, convert this to meters:
λ=6800Å=6800×10−10m
- The frequency (ν) can be calculated using the formula:
ν=cλ
- Where c is the speed of light, approximately 3×108m/s.
4. Substituting Values:
- Substitute the values into the frequency formula:
ν=3×108m/s6800×10−10m
5. Perform the Calculation:
- Calculate the frequency:
ν=3×1086800×10−10=3×1086.8×10−7≈4.41×1014s−1
6. Conclusion:
- Since we established that ν=ν₀, the threshold frequency of the metal is:
ν₀=4.41×1014Hz
Final Answer:
The threshold frequency of the metal is 4.41×1014Hz.
- The threshold frequency (ν₀) is the minimum frequency required to emit a photoelectron from a metal surface. When the energy of the incoming radiation is equal to the work function of the metal, the photoelectron is emitted with zero kinetic energy.
- The photoelectric equation is given by:
hν = hν₀ + KE
- Since the photoelectrons are emitted with zero velocity, the kinetic energy (KE) is zero. Therefore, the equation simplifies to:
hν=hν₀
- This implies that:
ν=ν₀
- We are given the wavelength (λ) of the radiation, which is 6800 Å. First, convert this to meters:
λ=6800Å=6800×10−10m
- The frequency (ν) can be calculated using the formula:
ν=cλ
- Where c is the speed of light, approximately 3×108m/s.
- Substitute the values into the frequency formula:
ν=3×108m/s6800×10−10m
- Calculate the frequency:
ν=3×1086800×10−10=3×1086.8×10−7≈4.41×1014s−1
- Since we established that ν=ν₀, the threshold frequency of the metal is:
ν₀=4.41×1014Hz
The threshold frequency of the metal is 4.41×1014Hz.
Topper's Solved these Questions
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - EXERCISE40videos
STRUCTURE OF ATOM
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - ASSIGNMENT (SECTION -A) Objective type questions50videos
STRUCTURE OF ATOM
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOMEXERCISE - EXAMPLE29videos
STRUCTURE OF ATOM
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STATES OF MATTEREXERCISE - ASSIGNMENT (SECTION-D)14videos
STATES OF MATTER
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRUCTURE OF ATOM EXERCISE - ASSIGNMENT ( SECTION -J) Aakash Challengers Questions12videos
STRUCTURE OF ATOM
Similar Questions
Calculate the threshold freqency of the metal from the photoelectrons are emitted with zero velocity when exposed to radiation of wavelength 6800 Å
Hint :KE=12mv2
hv=hv0+KE,KE=0
v=cλView SolutionElectrons are emitted with zero velocity from a metal surface when it is exposed to radiation of wavelength 6800 Å . Calculate threshold frequency (v0) and work function (W0) of the metal.
View SolutionCalculate the frequency of paritcle wave, when the kinetic energy of a sub-atomic particle is3.79×10−27J.
Hint :KE=12mv2
lambda = (h)/(mv) ,View Solutionthe threashold frequency v0 for a metal is 7×1014s−1 . Calculate the kinetic energy of an electron emitted when radiation of fequency v=1.0×1015s−1 hits the metal .
View Solutionthe threashold frequency v0 for a metal is 7×1014s−1 . Calculate the kinetic energy of an electron emitted when radiation of fequency v=1.0×1015s−1 hits the metal .
View SolutionIf a surface has a work function 4.0 eV, what is the maximum velocity of electrons liberated from the surface when it is irradiated with ultraviolet radiation of wavelength 0.2μm?
View SolutionCalculate the frequency of paritcle wave, when the kinetic energy of a sub-atomic particle is3.79×10−27J.
Hint :KE=12mv2
λ=hmv,λ=vv
∴vv=hmv,v=mv2h=2×12mv2hView SolutionThe threshold frequency v0 for a metal is 6×1014s−1. Calculate the kinetic energy of an electron emitted when radiation of frequency v=1.1×1015s−1 hits the metal.
View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectroic effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejercted electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Aborbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−01λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
Whaich of the following is the graph between the frequency (V) of the incident radiation and the stopping potential (v) ?View SolutionThe emission of electrons from a metal surface exposed rto light radaition of appropriate wavelength is called photoelectroic effect .The emmited electron are called photo=-weklectron work function of threshold energy may be defined as the minimum amount of energy required to ejercted electron from a most surface .According to Einstein
Maximum kinetic energy of ejected electron = Aborbed energy - Work function
12mv2max=h(v)−h(vn)=hv[1λ−01λn]
Where vn and λ0 are thereshold frequency and threshold wavelength respectively
Sopping potential : it is the miximum potential at which the photoelectric current becomes zero if V0 is the stopping potential eV0=h(v−v0)
The stopping potential as a function on electron frtequency is plotted for two photoelectric surface A abd B The graph show that the work function of A is
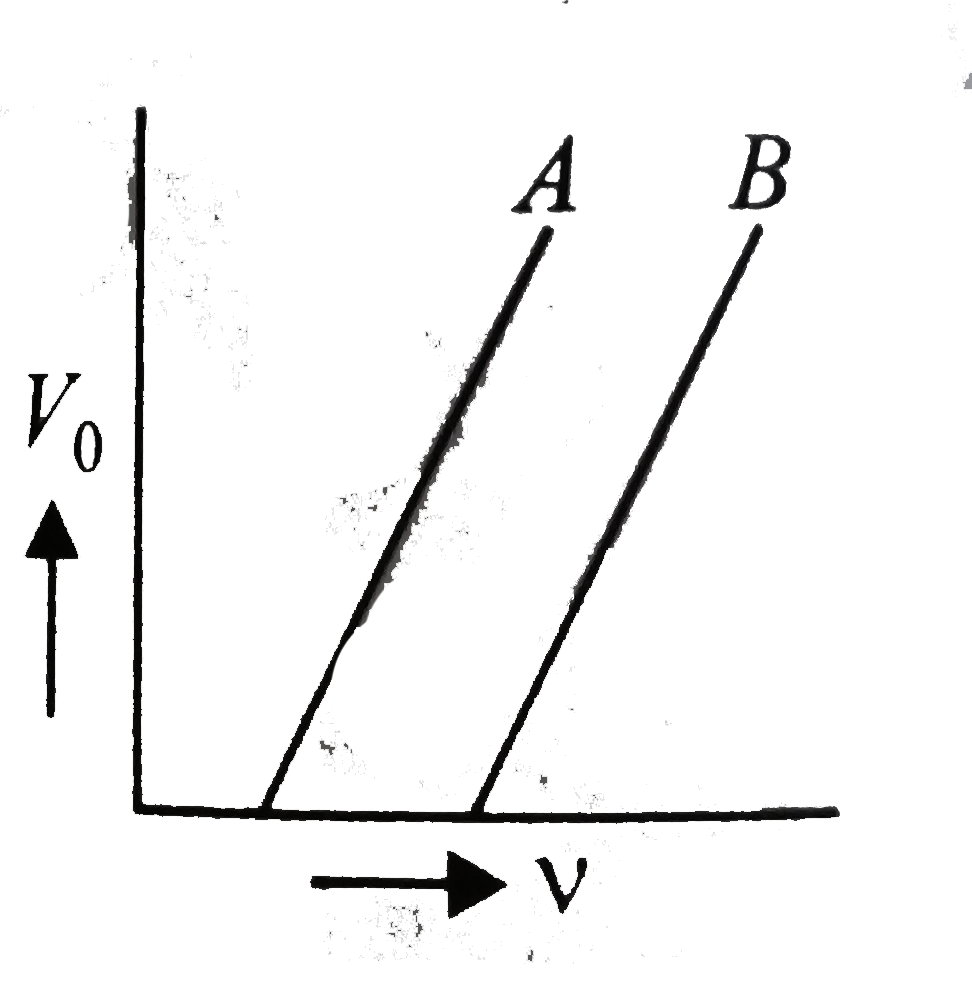 View Solution
View Solution
