Calculate the kinetic energy of β-particles and the radiation frequencies corresponding to the γ-decays shown in figure.
Given, mass of .12Mg27 atom =26.991425 amu and mass of .13Al27 atom = 26.990080 amu
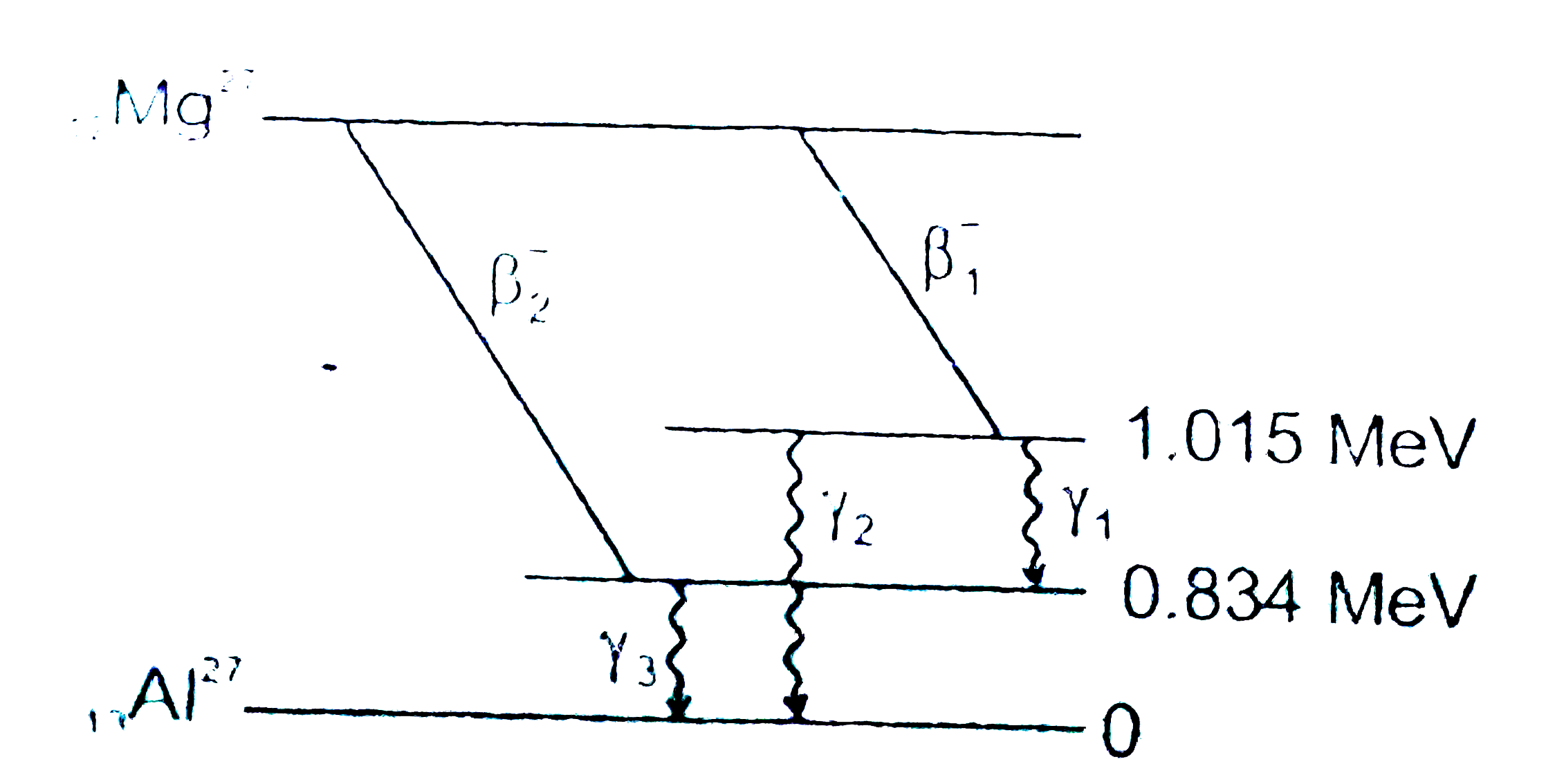
Energy of photon v1,hv1=E3−E2h=(1.015−0.834)MeV6.62×10−34Js
0.181×1.6×10−13J(6.62×10−34Js)=4.37×1019s−1
Energy of photon v2,v2=E3−E1h=(1.015−0)MeV6.62×10−34Js=2.45×1020s−1
Energy of photon v3,v3=E3−E1h=(0.834−0)MeV6.62×10−34=2.0×1020s−1
Now emission of β−1 particle is given by 12Mg27→13AI27+β−+v2+Q
Q=[m(12Mg27)−m(13AI27)−E(v2)
=[26.991425−26.990080]u−(E3−E1)MeV
=0.001345×931−1.015MeV
∴ K.E of β−2 particle is given by 12Mg27→13AI27+β−2+v3+Q
={[26.991425−26.990080]931−0.34}MeV
=0.418 MeV
Topper's Solved these Questions
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - Try Yourself
36 Videos
- NUCLEIBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - NUCLEIEXERCISE - Assignment Section A Objective (One option is correct )
52 Videos
- MOVING CHARGES AND MAGNETISMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGES AND MAGNETISMEXERCISE - Assignment Section J (Aakash Challengers Questions)
5 Videos
- OSCILLATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - OSCILLATIONSEXERCISE - Assignment (Section D) (ASSERTION-REASON TYPE QUESTIONS)
13 Videos
Similar Questions
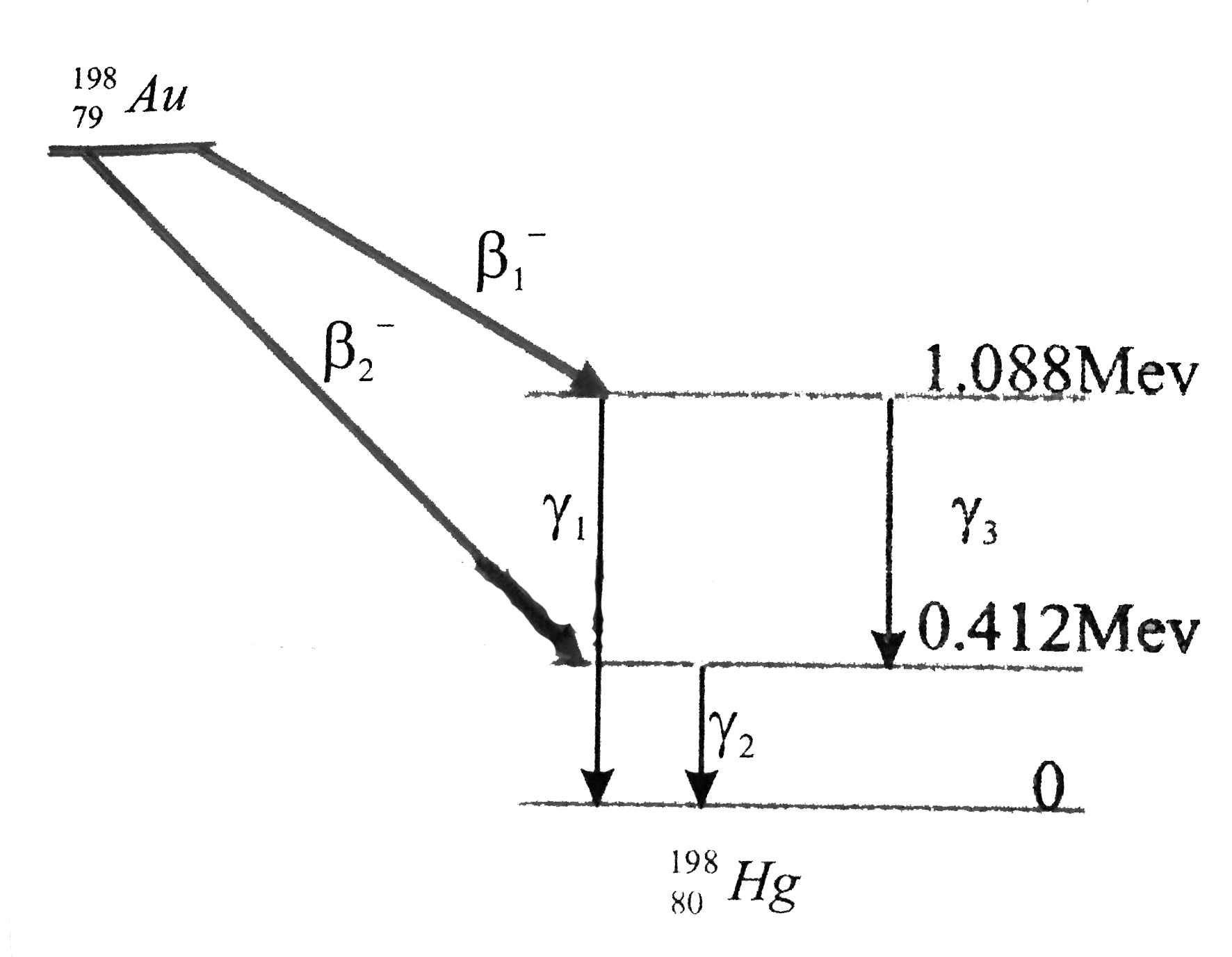
Obtain the maximum kinetic energy of β-particles, and the radiation frequencies of γ decays in the decay scheme shown in Fig. 14.6. You are given that m(.198Au)=197.968233u,m(.198Hg)=197.966760u
View Solution.^(239)Pu.94 is undergoing α−decay according to the equation .23994Pu→(.23597U)+.42He . The energy released in the process is mostly kinetic energy of the α-particle. However, a part of the energy is released as γ rays. What is the speed of the emiited α-particle if the γ rays radiated out have energy of 0.90MeV? Given: Mass of .23994Pu=239.05122u, mass of (.23597U)=235.04299u and mass of .41He=4.002602u(1u=931MeV).
View SolutionCalculate the energy released when three alpha particles combine to form a _12C nucleus. The atomic mass of _4He is 4.002603u.
View SolutionCalculate the number of gram atoms present in the following masses.
(i) 12.69 g of hydrogen, (ii) 40.089 g of calcium (Given, atomic mass of H = 1.008 amu and atomic mass of Ca = 40.08 amu)
View SolutionCalculate the binding energy of an α-particle in MeV Given : mp (mass of proton) = 1.007825 amu, mn (mass of neutron) = 1.008665 amu Mass of the nucleus =4.002800 amu, 1 amu = 931 MeV.
View SolutionThe mass of one atom of hydrogen is 1.008 amu. Calculate the mass of 26 atoms of hydrogen.
View SolutionCalculate de Broglie wavelength for an average helium atom in a furnace at 400 K. Given mass of helium=4.002amu.
View SolutionThere is a stream of neutrons with a kinetic energy of 0.0327eV. If the half-life of neutrons is 700 seconds, the fraction of neutrons that will decay before they travel a distance of 10 Km . (Given mass of neutron m=1.6758×10−27 kg )
View SolutionIn a nuclear reaction, α+7N14→X+p
When nitrogen atoms are bombarded by 7.7MeVα− particles, protons are ejected with a kinetic energy of 5.5MeV. The Q− value of the reaction is −1.26MeV
(a) Find the atomic mass of X in amu, identify the element X.
(b) Find the angle ϕ between the direction of motion of proton and α− particle.
Given the atomic mass of 1H1=1.00814a.m.u.,
atomic mass of 7N14=14.00752aμ, and atomic mass of 2He4=4.0038a.m.u.
View SolutionThe distance between the carbon atom and the oxygen atom in a carbon monoxide molecule is 1.1Å. Given, mass of carbon atom is 12 a.m.u. and mass of oxygen atom is 16 a.m.u., calculate the position of the centre of mass of the carbon monoxide molecule
View Solution
