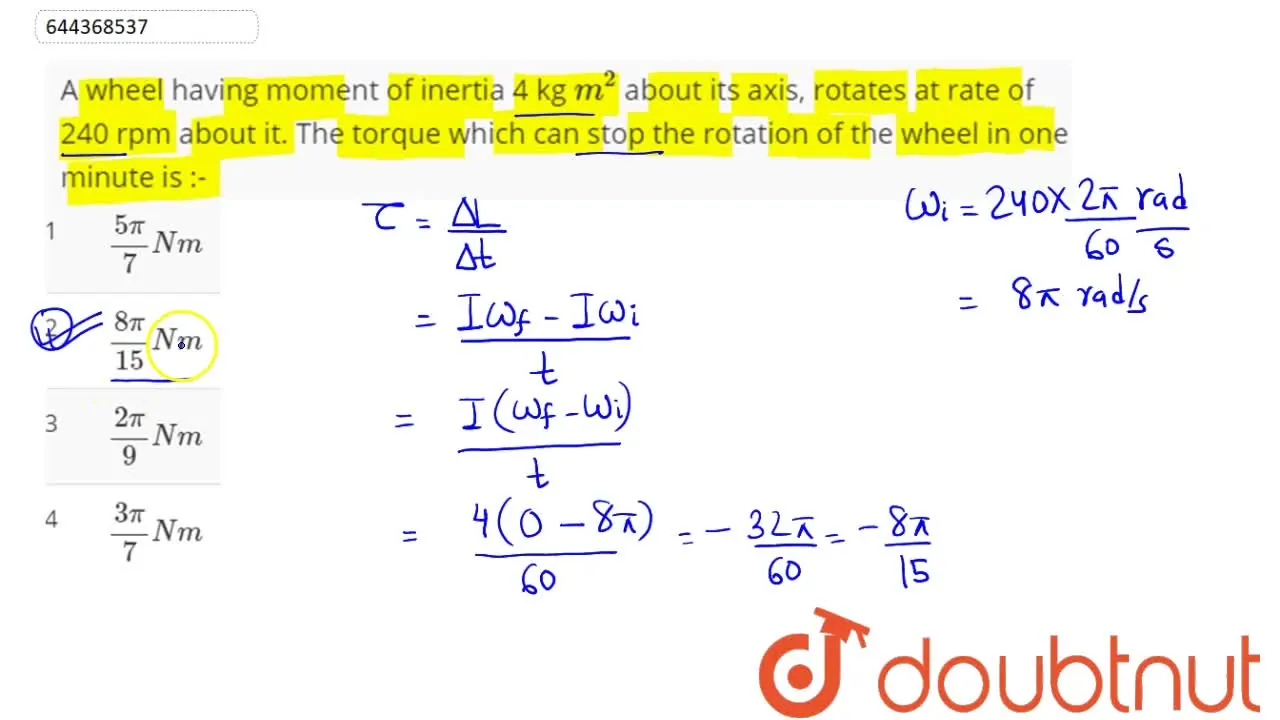
A wheel having moment of inertia 4 kg m2 about its axis, rotates at rate of 240 rpm about it. The torque which can stop the rotation of the wheel in one minute is :-
5π7Nm
8π15Nm
2π9Nm
3π7Nm
The correct Answer is:B
Step by step video & image solution for A wheel having moment of inertia 4 kg m^(2) about its axis, rotates at rate of 240 rpm about it. The torque which can stop the rotation of the wheel in one minute is :- by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Similar Questions
A wheel having moment of inertia 2kgm2 about its vertical axis, rotates at the rate of 60r± about this axis. The torque which can stop the wheel's rotation in one minute would be
View SolutionA wheel having moment of inertia 2kg-m2 about its vertical axis, rotates at the rate of 60 rpm about this axis. The torque which can stop the wheel's rotation in one minute would be
View SolutionA wheel having moment of inertia 2 kg m^2 about its axis, rotates at 50 rpm about this axis. Find the torque that can stop the wheel in one minute.
View SolutionA wheel having moment of interia 2kgm−2 about its axis, rotates at 50 rpm about this axis. The angular retardation that can stop the wheel in one minute is
View SolutionA wheel having moment of inertia 2π2 kgm2 is at rest. It is rotated by a 60 W ideal motor for one minute. The angular displacement traversed by the wheel in one minute is
View SolutionA flywheel having moment of inertia 20kgm2 rotates about its axis with an angular velocity of 100rads−1. If the moment of inertia of the flywheel is reduced to 10kgm2 without applying external torque, calculate the new angular velocity of the Flywheel.
View SolutionA flywheel having moment of inertia 20kgm2 rotates about its axis with an angular velocity of 100rads−1. If the moment of inertia of the flywheel is reduced to 10kgm2 without applying external torque, calculate the new angular velocity of the Flywheel.
View SolutionA uniform disc of moment of inertia 1kgm2 rotates with a speed of 120 rpm, Calculate the torque required to stop the disc in 10 revolutions ?
View SolutionA flywheel of moment of inertia 2kg-m2 is rotated at a speed of 30rad/s. A tangential force at the rim stops the wheel in 15 second. Average torque of the force is
View SolutionThe moment of inertia of a body about a given axis of rotation depends upon :-
View Solution