Calculate the instantenous power of a wheel rotating with an angular velocity of 20rad/s, when a torque of 10Nm is applied to it.
To calculate the instantaneous power of a wheel rotating with a given angular velocity and torque, we can follow these steps:
1. Identify the Given Values:
- Angular velocity (ω) = 20 rad/s
- Torque (τ) = 10 Nm
2. Recall the Formula for Instantaneous Power:
The formula for instantaneous power (P) in terms of torque (τ) and angular velocity (ω) is:
P=τ⋅ω
3. Substitute the Given Values into the Formula:
Substitute τ = 10 Nm and ω = 20 rad/s into the power formula:
P=10Nm⋅20rad/s
4. Perform the Calculation:
P=200W
5. Conclusion:
The instantaneous power of the wheel is 200 watts.
- Angular velocity (ω) = 20 rad/s
- Torque (τ) = 10 Nm
The formula for instantaneous power (P) in terms of torque (τ) and angular velocity (ω) is:
P=τ⋅ω
Substitute τ = 10 Nm and ω = 20 rad/s into the power formula:
P=10Nm⋅20rad/s
P=200W
The instantaneous power of the wheel is 200 watts.
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration
4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)
62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
Calculate the instantaneous power of a body rotating with angular velocity of 20rad/s−2, when an external torque of 5Nm is applied to it.
Hint : P=τω.
View SolutionCalculate the instantaneous power of a body rotating with angular velocity of 20rad/s−1, when an external torque of 5Nm is applied to it.
Hint : P=τω.
View SolutionCompute the angular acceleration of a wheel having moment of inertia 20kgm2, when a torque of 10Nm is applied on the wheel.
View SolutionCompute the angular acceleration of a wheel having moment of inertia 20kgm2, when a torque of 10Nm is applied on the wheel.
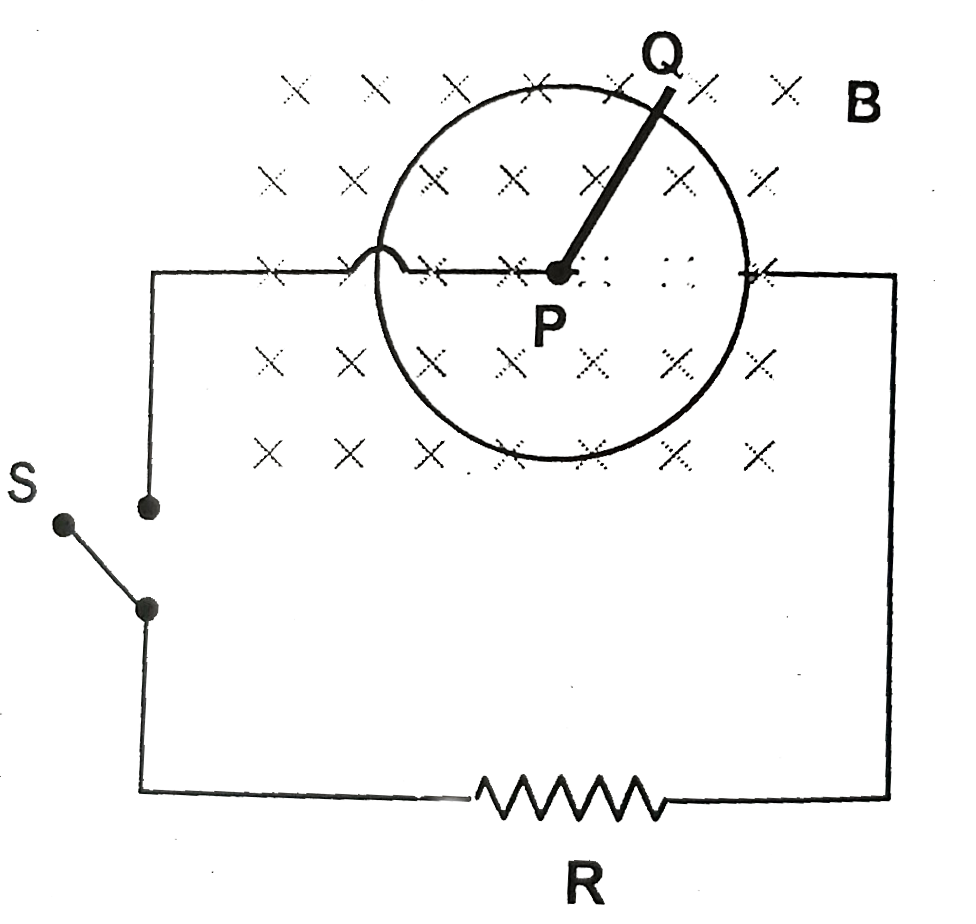
View SolutionA resitance R=1kΩ connected to a conducting rod PQ that can slide on a conducting circular ring of radius 2m with center at P. A constant uniform magnetic field 10 Tesla exist as shown in figure. At t=0, switch 'S' is closed and simulaneously an external torque is applied on the rod so that it rotates with constant angular velocity 20rad/sec. The magnitude of power delivered by external is 20K Watt. Find the value of K. (Neglect the gravity, friction and self inductance of circular loop)
View SolutionCalculate the angular velocity with which a wheel is rotating. If its instanteous power is 500W, and an external torque of 50Nm is applied to it.
Hint : P=τω
View SolutionCalculate the angular velocity with which a wheel is rotating. If its instanteous power is 500W, and an external torque of 50Nm is applied to it.
Hint : P=τω
View SolutionA wheel whose moment of inertia is 10kgm2 has an initial angular velocity of20rad/s. A constant torque of 20Nm acts on the wheel. The time in which wheel is acceleration to 100rad/s is
View SolutionWhen a constant torque is applied to a flywheel at rest it angular velocity increases by 10 rad/s in 2 s: Calculate the torque applied if the moment of inertia of the flywheel about the axis of rotation is 200kgm2.
View SolutionA wheel rotates with a constant angular velocity of 300 rpm. The angle through which the wheel rotates in 1 s is.
View Solution
