A rectangular surface of sides 10cmand15cm is palaced inside a uniform electric field fo 25Vm−1, such that normal to the surface makes an angle of 60∘ with the direction of electric field. Find the flux of electric field through the rectangular surface.
To find the electric flux through the rectangular surface, we can use the formula for electric flux, which is given by:
Φ=E⋅A⋅cos(θ)
where:
- Φ is the electric flux,
- E is the magnitude of the electric field,
- A is the area of the surface,
- θ is the angle between the electric field and the normal to the surface.
Step 1: Calculate the Area of the Rectangular Surface
The area A of the rectangle can be calculated using the formula:
A=length×width
Given the dimensions of the rectangle are 10cm and 15cm, we first convert these dimensions to meters:
10cm=0.1m,15cm=0.15m
Now, calculate the area:
A=0.1m×0.15m=0.015m2
Step 2: Identify the Electric Field and Angle
The electric field E is given as 25V/m, and the angle θ between the electric field and the normal to the surface is 60∘.
Step 3: Calculate the Cosine of the Angle
Next, we need to find cos(60∘):
cos(60∘)=12
Step 4: Substitute Values into the Flux Formula
Now we can substitute the values into the flux formula:
Φ=E⋅A⋅cos(θ)
Substituting the known values:
Φ=25V/m⋅0.015m2⋅12
Step 5: Calculate the Electric Flux
Now, perform the calculation:
Φ=25⋅0.015⋅0.5=0.1875Nm2/C
Final Answer
Thus, the electric flux through the rectangular surface is:
Φ=0.1875Nm2/C
---
- Φ is the electric flux,
- E is the magnitude of the electric field,
- A is the area of the surface,
- θ is the angle between the electric field and the normal to the surface.
The area A of the rectangle can be calculated using the formula:
The electric field E is given as 25V/m, and the angle θ between the electric field and the normal to the surface is 60∘.
Next, we need to find cos(60∘):
Now we can substitute the values into the flux formula:
Now, perform the calculation:
Thus, the electric flux through the rectangular surface is:
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - TRY YOURSELF
33 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - ASSIGNMENT(SECTION-A) Objective Type Question
45 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - ASSIGNMENT (SECTION-D)
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ASSIGNMENT(SECTION -D) Assertion-Reason type Question)
15 Videos
Similar Questions
If the flux of the electric field through a closed surface is zero,
View SolutionA coil of area 5cm2 and having 20 turns is placed in a uniform magnetic field of 103 gauss. The normal to the plane of the coil makes an angle of 60∘ with the magnetic field. The flux in Maxwell through the coil is:
View Solution
Knowledge Check
A circular plane sheet of radius 10 cm is placed in a uniform electric field of 5×105NC−1, making an angle of 60∘ with the field. Calculate electric flux through the sheet.
A1.36×102Nm2C−1B1.36×104Nm2C−1C0.515×102Nm2C−1D0.515×104Nm2C−1
A coil of area 5cm2 and having 20 turns is placed in a uniform magnetic field of 103 gauss. The normal to the plane of the coil makes an angle of 60∘ with the magnetic field. The flux in Maxwell through the coil is:
View SolutionIn a uniform electric field find the total flux associated with the given surfaces
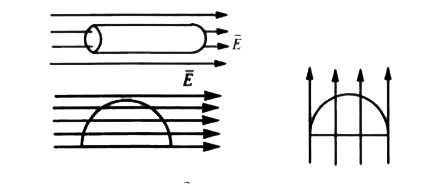
View SolutionA charge Q is placed at the centre of a cube. Find the flux of the electric field through the six surfaced of the cube.
View SolutionAn electric dipole is placed in an uniform electric field with the dipole axis making an angle θ with the direction of the electric field. The orientation of the dipole for stable equilibrium is
View SolutionA square frame of edge 10 cm is placed with its positive normal making on angle of 600 with a uniform electric field of 20Vm−1. Find the flux of the electric field through the surface bounded by the frame.
View SolutionFigure shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is
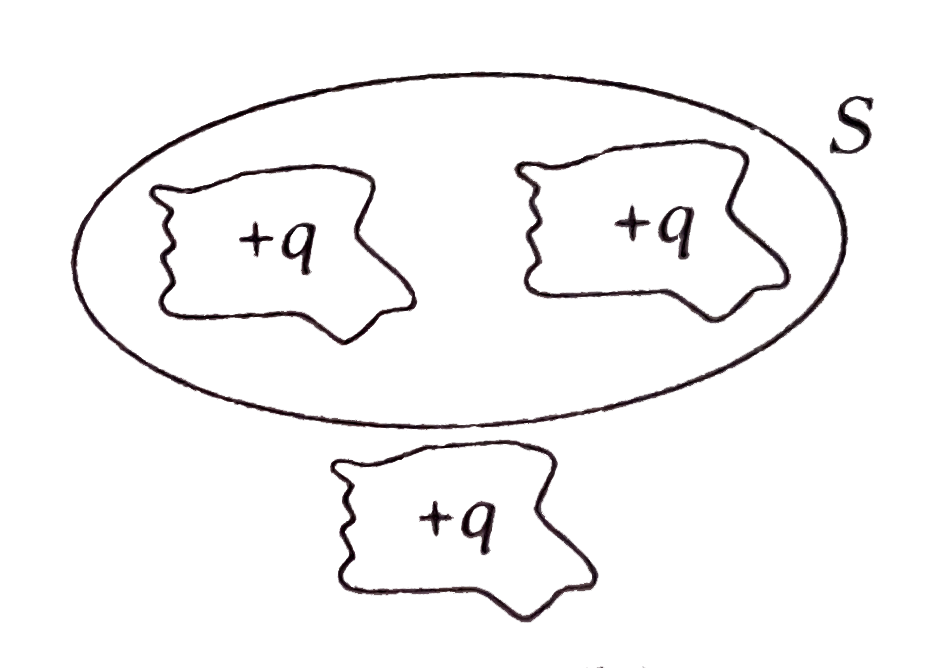
View SolutionFigure shown below is a distribution of charges. The flux of electric field due to these charges through the surface S is

View Solution
