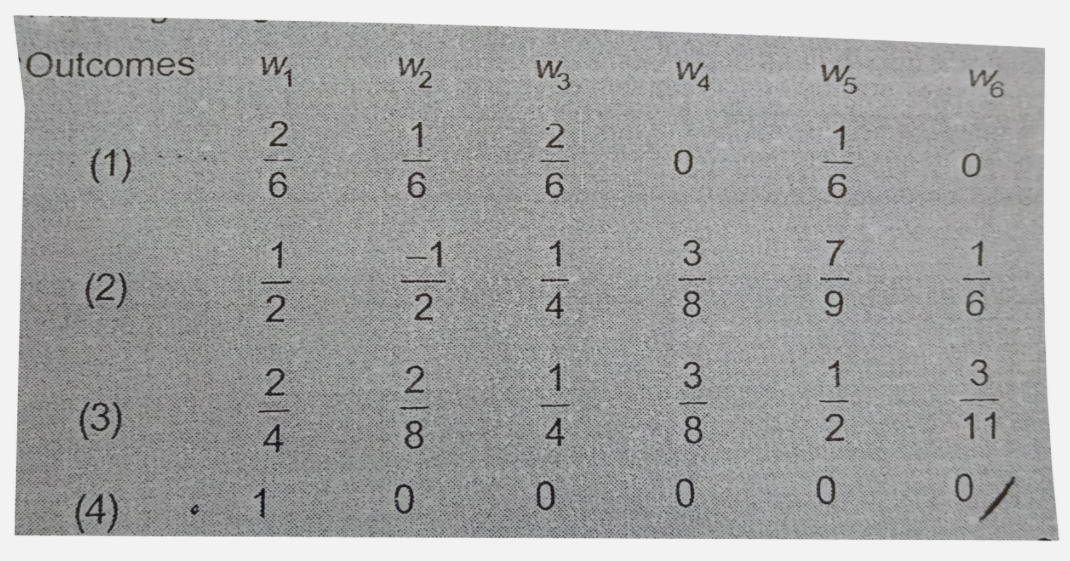
Consider a sample space S={W1,W2,W3,……W6}. Where Wi∩Wj=ϕ,∀i≠j. Which of the following assignments of probabilities to each outcome are valid? {Where Wi∩Wj=ϕ,∀I≠j} outcomes
Video Solution
Text Solution
(1) and (4)
|
Topper's Solved these Questions
- PROBABILITYBook:AAKASH INSTITUTE ENGLISHChapter:PROBABILITYExercise:ASSIGNMENT (SECTION - A Competition Level Questions)
Explore 114 Videos
- PROBABILITYBook:AAKASH INSTITUTE ENGLISHChapter:PROBABILITYExercise:ASSIGNMENT (SECTION - B Objective Type Questions (One option is correct))
Explore 64 Videos
- PROBABILITYBook:AAKASH INSTITUTE ENGLISHChapter:PROBABILITYExercise:ASSIGNMENT SECTION-J (aakash challengers questions)
Explore 11 Videos
- PRINCIPLE OF MATHEMATICAL Book:AAKASH INSTITUTE ENGLISHChapter:PRINCIPLE OF MATHEMATICAL Exercise:Section-D:(Assertion-Reason Type Questions)
Explore 11 Videos
- RELATIONS AND FUNCTIONS Book:AAKASH INSTITUTE ENGLISHChapter:RELATIONS AND FUNCTIONS Exercise:Assignment (Section - J) Aakash Challengers Questions
Explore 8 Videos
Similar Questions
Knowledge Check
If the sample space of a random experiment is S={w1,w2,...,w6}, then which of the following arrangements of probability are valid?
 A(i) and (iii) onlyB(i) , (ii) and (iii) onlyC(i) and (ii) onlyD(i) , (ii) , (iii) and (iv)
A(i) and (iii) onlyB(i) , (ii) and (iii) onlyC(i) and (ii) onlyD(i) , (ii) , (iii) and (iv)18−3ww+6=w2w+6
Which of the following represents the sum of all possible solutions to the equation above?A−9B−3C3D9If w and z represents two complex numbers such that w=3−2iandz=−2+4i, which of he following gives w2 written in the form a+bi? (Note: i2=−1)
A−32−12iB−710−25iC1320−12iD76+23i
Which of the following are not state functions?
(I) q+w
(II)q
(III) w
(IV) H−TS
View SolutionConsider the two complex numbers z and w, such that
w=z−1z+2=a+ib, where a,b∈R and i=√−1.
If z=CiSθ, which of the following does hold good?
View SolutionObserve the following reaction sequence
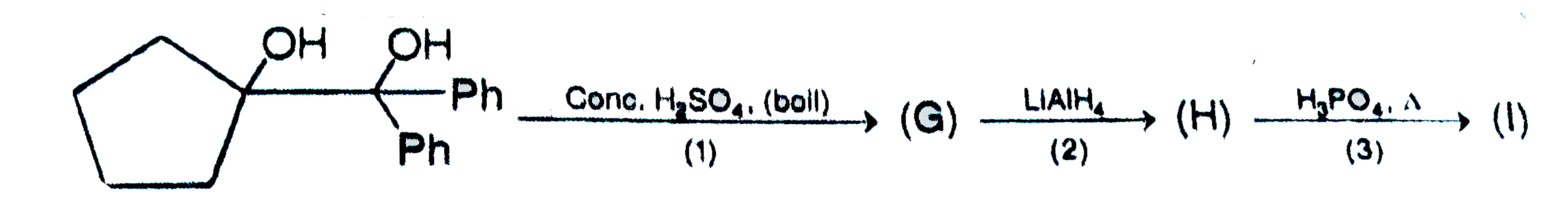
Calculate molecular mass [W] of product I and report your answer as N, where N=W÷3.
View SolutionIf two fair dices are thrown and digits on dices are a and b, then find the probability for which ωab=1, (where ω is a cube root of unity).
View SolutionIf z=x+yi,ω=2−iz2z−iand|ω|=1, find the locus of z in the complex plane
View Solution

