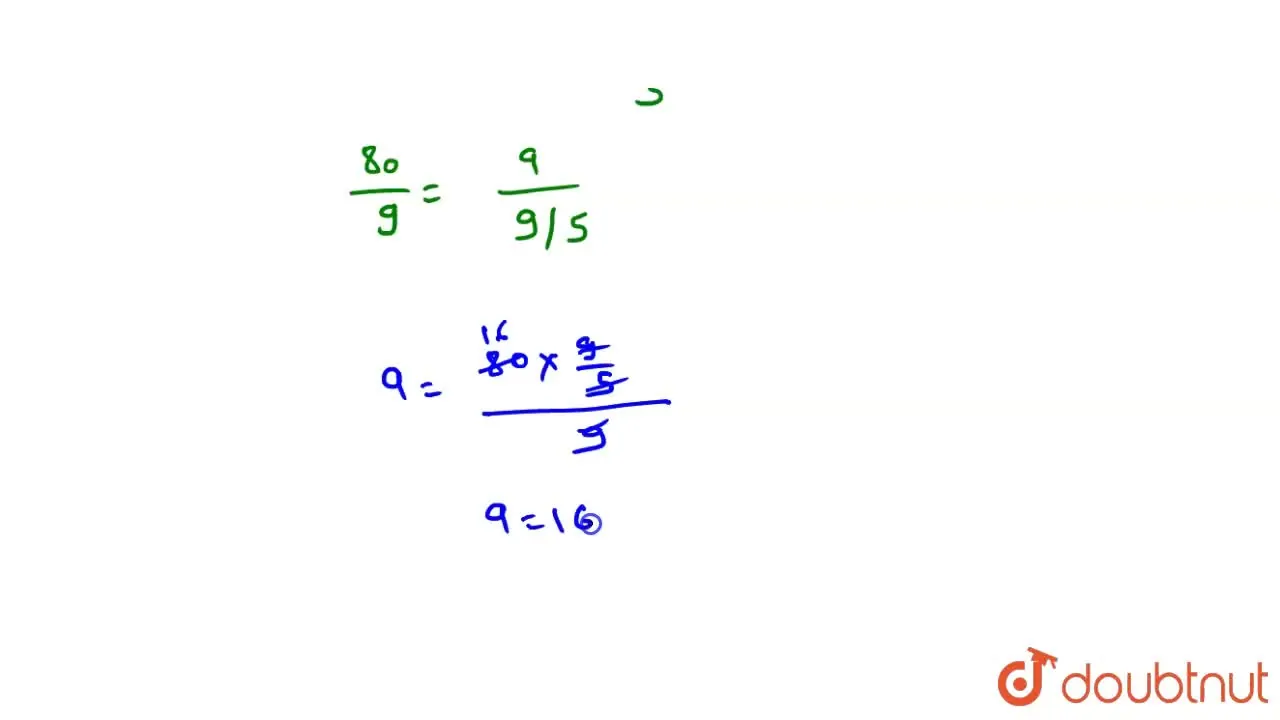If the common ratio is −45 and the sum of infinite terms in a G.P is 809 then find the first term.
r=−45andS∞=a1−r=809
∴a1+45=809
⇒5a=80
⇒a=16
Related Playlists
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - A) One option is correct60videos
SEQUENCES AND SERIES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - B) One option is correct49videos
SEQUENCES AND SERIES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers11videos
SEQUENCES AND SERIES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions8videos
RELATIONS AND FUNCTIONS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)4videos
SETS
Similar Questions
The common ratio of a GP is −45 and the sum to infinity is 809 . The first term of GP isView SolutionThe second term of a G.P. is 2 and the sum of infinite terms is 8. Find the first term.
View SolutionIf S is the sum to infinite terms of a G.P whose first term is 'a', then the sum of the first n terms is
View SolutionIf the sum of n terms of a G.P. is 33n+142n , then find the common ratio.
View SolutionThe sum oif the first ten terms of an A.P. is equal to 155, and the sum of the first two terms of a G.P. is 9. Find these progressionsif the first term of the A.P. equals the common ratio of the G.P. and the 1st term of G.P. equals the common difference of A.P.
View SolutionThe first and last term of a geometrical Pregression (G.P.) are 3 and 96 respectively. If the common ratio is 2, find :
(i) 'n' the number of terms of the G.P.
(ii) Sum of the n terms.View SolutionIf the sum of n terms of a G.P. is 3−3n+142n , then find the common ratio.
View SolutionThe sum of first three terms of a G.P is 16 and the sum of the next three terms is 128. Determine the first term, the common ratio and the sum to n terms of the G.P.
View SolutionThe sum of first three terms of a G.P. is 16 and the sum of the next three terms is 128. Determine the first term, the common ratio and the sum to n terms of the GP.
View SolutionFind the common difference of an A.P. whose first term is 5 and the sum of its first four terms is half the sum of the next four terms.
View Solution