If A1,A2,A3,A4andA5 are the five A.M.'s between 2 nd 8, then find the value of A1+A2+A3+A4+A5
To find the sum of the five arithmetic means (A.M.s) between the numbers 2 and 8, we can follow these steps:
Step 1: Understand the Concept of Arithmetic Means
The arithmetic means between two numbers are equally spaced values that lie between those two numbers. If we denote the two numbers as a and b, and we want to find n arithmetic means between them, we can use the formula for the arithmetic mean.
Step 2: Identify the Given Values
In this case, we have:
- The first number a=2
- The second number b=8
- The number of arithmetic means n=5
Step 3: Calculate the Arithmetic Mean Between the Two Numbers
The arithmetic mean (A.M.) between two numbers a and b is given by:
A.M.=a+b2
Substituting the values:
A.M.=2+82=102=5
Step 4: Use the Formula for the Sum of Arithmetic Means
The sum of n arithmetic means between two numbers can be calculated using the formula:
A1+A2+A3+A4+A5=n×A.M.
Substituting the values we have:
A1+A2+A3+A4+A5=5×5=25
Step 5: Conclusion
Thus, the sum of the five arithmetic means between 2 and 8 is:
25
---
The arithmetic means between two numbers are equally spaced values that lie between those two numbers. If we denote the two numbers as a and b, and we want to find n arithmetic means between them, we can use the formula for the arithmetic mean.
In this case, we have:
- The first number a=2
- The second number b=8
- The number of arithmetic means n=5
The arithmetic mean (A.M.) between two numbers a and b is given by:
A.M.=a+b2
Substituting the values:
A.M.=2+82=102=5
The sum of n arithmetic means between two numbers can be calculated using the formula:
A1+A2+A3+A4+A5=n×A.M.
Substituting the values we have:
A1+A2+A3+A4+A5=5×5=25
Thus, the sum of the five arithmetic means between 2 and 8 is:
25
Topper's Solved these Questions
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - A) One option is correct
60 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - B) One option is correct
49 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
11 Videos
- RELATIONS AND FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - RELATIONS AND FUNCTIONS EXERCISE - Assignment (Section - J) Aakash Challengers Questions
8 Videos
- SETS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)
4 Videos
Similar Questions
Knowledge Check
IfA1andA2 are two AMs between a and b then (2A1−A2)(2A2−A1)=
AabBbaCbaDa2bLet A1,A2…..,A3 be n arithmetic means between a and b. Then the common difference of the AP is
A(a) b−aB(b) a−bC(c) a−bn+1D(d) b−an+1
If a1,a2,a3,....an are positive real numbers whose product is a fixed number c, then the minimum value of a1+a2+....+an−1+3an is
View SolutionIf a1,a2,a3,....an are positive real numbers whose product is a fixed number c, then the minimum value of a1+a2+.......an−1+2an is
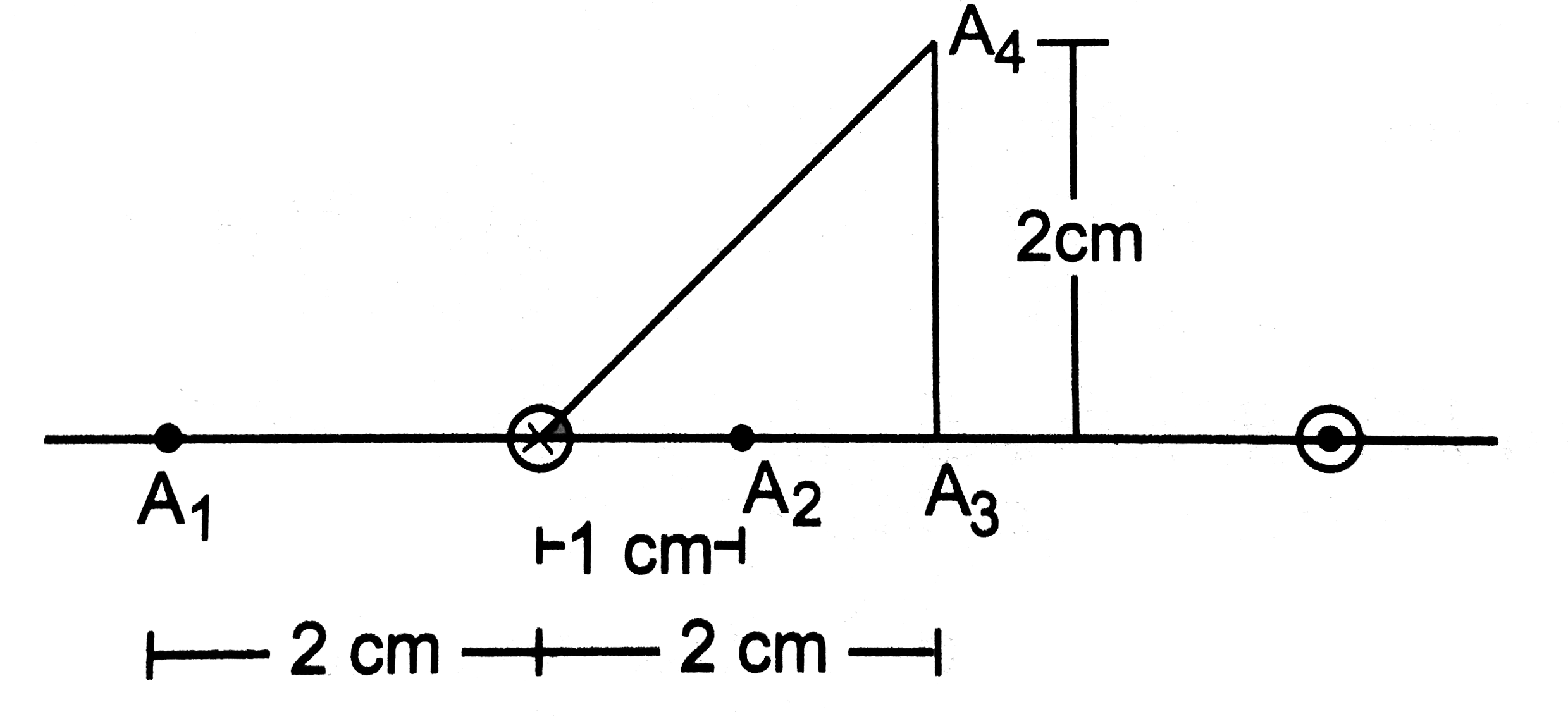
View SolutionFigure shows two parallel wires separated by a distance of 4.0 cm and carrying equal currents of 10A along opposite directions. Find the magnitude of the magnetic field B at the points A1,A2,A3andA4.
View SolutionIf a1,a2,a3,a4 be the coefficient of four consecutive terms in the expansion of (1+x)n, then prove that: a1a1+a2+a3a3+a4=2a2a2+a3.
View SolutionIf a1,a2,a3,a4 be the coefficient of four consecutive terms in the expansion of (1+x)n, then prove that: a1a1+a2+a3a3+a4=2a2a2+a3.
View SolutionFive different objects A1,A2,A3,A4,A5 are distributed randomly in 5 places marked 1, 2, 3, 4, 5. One arrangement is picked at random. The probability that in the selected arrangement, none of the object occupies the place corresponding to its number, is
View Solution
