A vector lying in x-y plane has a magnitude 3, and makes an angle 30∘ with the x-axis. Find its components along the two axes.
To find the components of a vector lying in the x-y plane with a given magnitude and angle, we can follow these steps:
Step 1: Understand the problem
We have a vector →A with a magnitude of 3 that makes an angle of 30∘ with the x-axis. We need to find its components along the x-axis and y-axis.
Step 2: Identify the components
The components of the vector can be found using trigonometric functions:
- The x-component (Ax) can be calculated using the cosine of the angle.
- The y-component (Ay) can be calculated using the sine of the angle.
Step 3: Write the formulas
The formulas for the components are:
Ax=A⋅cos(θ)
Ay=A⋅sin(θ)
where:
- A is the magnitude of the vector (3 in this case),
- θ is the angle with the x-axis (30 degrees).
Step 4: Substitute the values
Substituting the known values into the formulas:
Ax=3⋅cos(30∘)
Ay=3⋅sin(30∘)
Step 5: Calculate the components
Now, we need to calculate the cosine and sine of 30∘:
- cos(30∘)=√32
- sin(30∘)=12
Now substituting these values:
Ax=3⋅√32=3√32
Ay=3⋅12=32
Step 6: Final result
Thus, the components of the vector are:
- Ax=3√32
- Ay=32
Summary
The components of the vector along the x-axis and y-axis are:
- Ax=3√32
- Ay=32
We have a vector →A with a magnitude of 3 that makes an angle of 30∘ with the x-axis. We need to find its components along the x-axis and y-axis.
The components of the vector can be found using trigonometric functions:
- The x-component (Ax) can be calculated using the cosine of the angle.
- The y-component (Ay) can be calculated using the sine of the angle.
The formulas for the components are:
Ax=A⋅cos(θ)
Ay=A⋅sin(θ)
where:
- A is the magnitude of the vector (3 in this case),
- θ is the angle with the x-axis (30 degrees).
Substituting the known values into the formulas:
Ax=3⋅cos(30∘)
Ay=3⋅sin(30∘)
Now, we need to calculate the cosine and sine of 30∘:
- cos(30∘)=√32
- sin(30∘)=12
Ax=3⋅√32=3√32
Ay=3⋅12=32
Thus, the components of the vector are:
- Ax=3√32
- Ay=32
The components of the vector along the x-axis and y-axis are:
- Ax=3√32
- Ay=32
Topper's Solved these Questions
- MOTION IN A PLANEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A PLANEExercise:llustration 1 :
Explore 1 Video
- MOTION IN A PLANEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A PLANEExercise:Try yourself
Explore 48 Videos
- MOCK_TEST_17Book:AAKASH INSTITUTE ENGLISHChapter:MOCK_TEST_17Exercise:Example
Explore 15 Videos
- MOTION IN A STRAIGHT LINEBook:AAKASH INSTITUTE ENGLISHChapter:MOTION IN A STRAIGHT LINEExercise:ASSIGNMENT (SECTION - D)
Explore 15 Videos
Similar Questions
A vector of magnitude 13 makes an angle 65.37∘ with the x-axis . What is its component along positive y-axis ?
View SolutionA displacement vector of magnitude 4 makes an angle 30∘ with the x-axis. Its rectangular components in x-y plane are
View SolutionA displacment vector fo magnitude 4 makes an angle 30∘ with the x-axis. Its rectangular components in x-y plane are
View SolutionLet AB be a vector in two dimensional plane with the magnitude 4 units and making an angle of 30∘ with X-axis and lying in the first quadrant. Find the components of AB along the two axes off coordinates. Hence, represent AB in terms of unit vectors ˆiandˆj.
View SolutionA tangent to the parabola y2=16x makes an angle of 60∘ with the x-axis. Find its point of contact.
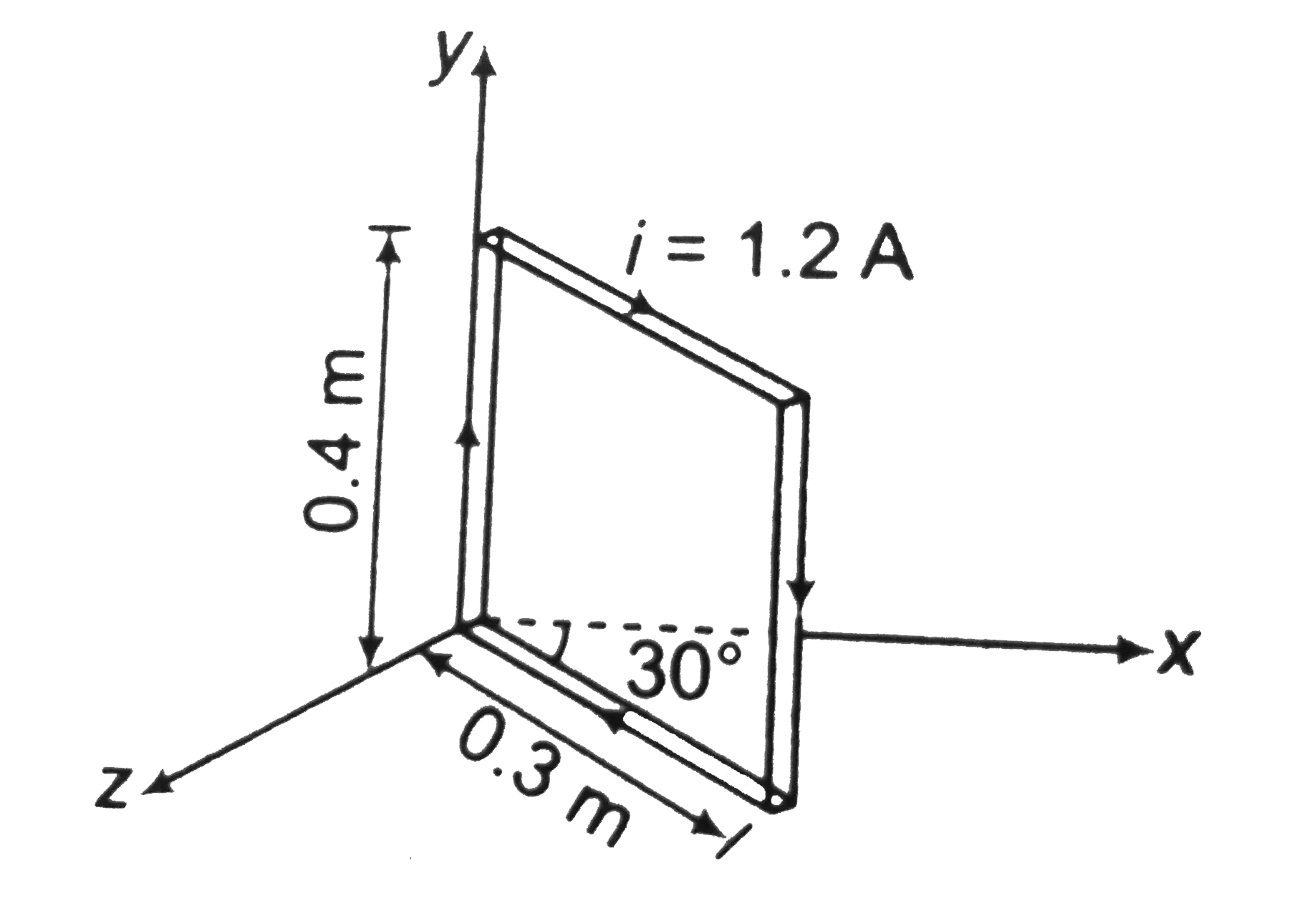
View SolutionA rectangular loop consists of N=100 closed wrapped turns and has dimensions (0.4m×0.3m). The loop is hinged along the y-axis and its plane makes an angle θ=30∘ with the x-axis. What is the magnitude of the torque exerted on the loop by a uniform magnetic field B=0.8T directed along the x-axis when current is i=1.2A in the direction shown. What is the expected direction of rotation of the loop?
View SolutionA vector having magnitude 30 unit makes equal angles with each of X,Y, and Z -axes The components of vector along each of X,Y,and Z -axes are
View SolutionX- component of →a is twice of its Y- component. If the magnitude of the vector is 5√2 and it makes an angle of 135∘ with z-axis then the components of vector is:
View SolutionLet −−→AB be a vector in two dimensional plane with magnitude 4 units. And making an anle of 600 with x-axis, and lying in first quadrant. Find the components of −−→AB in terms of unit vectors ˆiandˆj. so verify that calculation of components is correct.
View SolutionA vector →A makes an angle of 20∘and→B makes an angle of −−→110∘ with the X-axis. The magnitude of these vectors are 3 m and 4 m respectively.Find the resultant
View Solution