Prove that when the sides of a triagle are taken in order, it leads to zero resultant.
To prove that when the sides of a triangle are taken in order, it leads to a zero resultant, we can follow these steps:
Step 1: Draw a Triangle
Let's consider a triangle ABC. We will represent the sides of the triangle as vectors.
Step 2: Define the Vectors
Let:
- →AB be represented by vector →A
- →BC be represented by vector →B
- →CA be represented by vector →C
Step 3: Apply the Triangle Law of Vector Addition
According to the triangle law of vector addition, if two sides of a triangle are represented by vectors, then the resultant vector in direction and magnitude is given by the third side of the triangle. Mathematically, we can express this as:
→AB+→BC=→AC
However, since we are considering the sides in order, we will express this as:
→AB+→BC−→AC=0
Step 4: Substitute the Vector Representations
Substituting the vectors we defined earlier:
→A+→B−→C=0
Step 5: Rearrange the Equation
Rearranging the equation gives us:
→A+→B+→C=0
Conclusion
This shows that the sum of the vectors representing the sides of the triangle taken in order results in zero. Therefore, we have proved that when the sides of a triangle are taken in order, it leads to a zero resultant.
Let's consider a triangle ABC. We will represent the sides of the triangle as vectors.
Let:
- →AB be represented by vector →A
- →BC be represented by vector →B
- →CA be represented by vector →C
According to the triangle law of vector addition, if two sides of a triangle are represented by vectors, then the resultant vector in direction and magnitude is given by the third side of the triangle. Mathematically, we can express this as:
→AB+→BC=→AC
However, since we are considering the sides in order, we will express this as:
→AB+→BC−→AC=0
Substituting the vectors we defined earlier:
→A+→B−→C=0
Rearranging the equation gives us:
→A+→B+→C=0
This shows that the sum of the vectors representing the sides of the triangle taken in order results in zero. Therefore, we have proved that when the sides of a triangle are taken in order, it leads to a zero resultant.
Topper's Solved these Questions
- VECTOR ALGEBRABOOK - AAKASH INSTITUTE ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - ILLUSTRATION
1 Video
- VECTOR ALGEBRABOOK - AAKASH INSTITUTE ENGLISHCHAPTER - VECTOR ALGEBRAEXERCISE - TRY YOURSELF
20 Videos
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section - J (Akash Challengers Question)
16 Videos
Similar Questions
Assertion: The minimum number of vectors of unequal magnitude required to produce zero resultant is three.
Reason: Three vectors of unequal magnitude which can be represented by the three sides of a triangle taken in order, produce zero resultant.
View SolutionIf →a, →b, →c be the vectors represented by the sides of a triangle, taken in order, then prove that →a+→b+→c=→0.
View SolutionIf →a, →b, →c represent the sides of a triangle taken in order, then write the value of →a+→b+→c
View SolutionIf →a,→band→c represents three sides of a triangle taken in order prove that
→a×→b=→b×→c=→c×→a
View SolutionThe quadrilateral formed by joining the mid-points of the sides of a quadrilateral PQRS, taken in order, is a rectangle, if
View SolutionThe figure formed by joining the mid-points of the sides of a quadrilateral ABCD, taken in order, is a square only, if
View SolutionIf A,B,C represents the three sides of an equilateral triangle taken in the same order then find the angle between i) A and B ii) B and C iii) A and C.
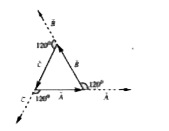
View SolutionUsing vector method, prove that the line segments joining the mid-points of the adjacent sides of a quadrilateral taken in order form a parallelogram.
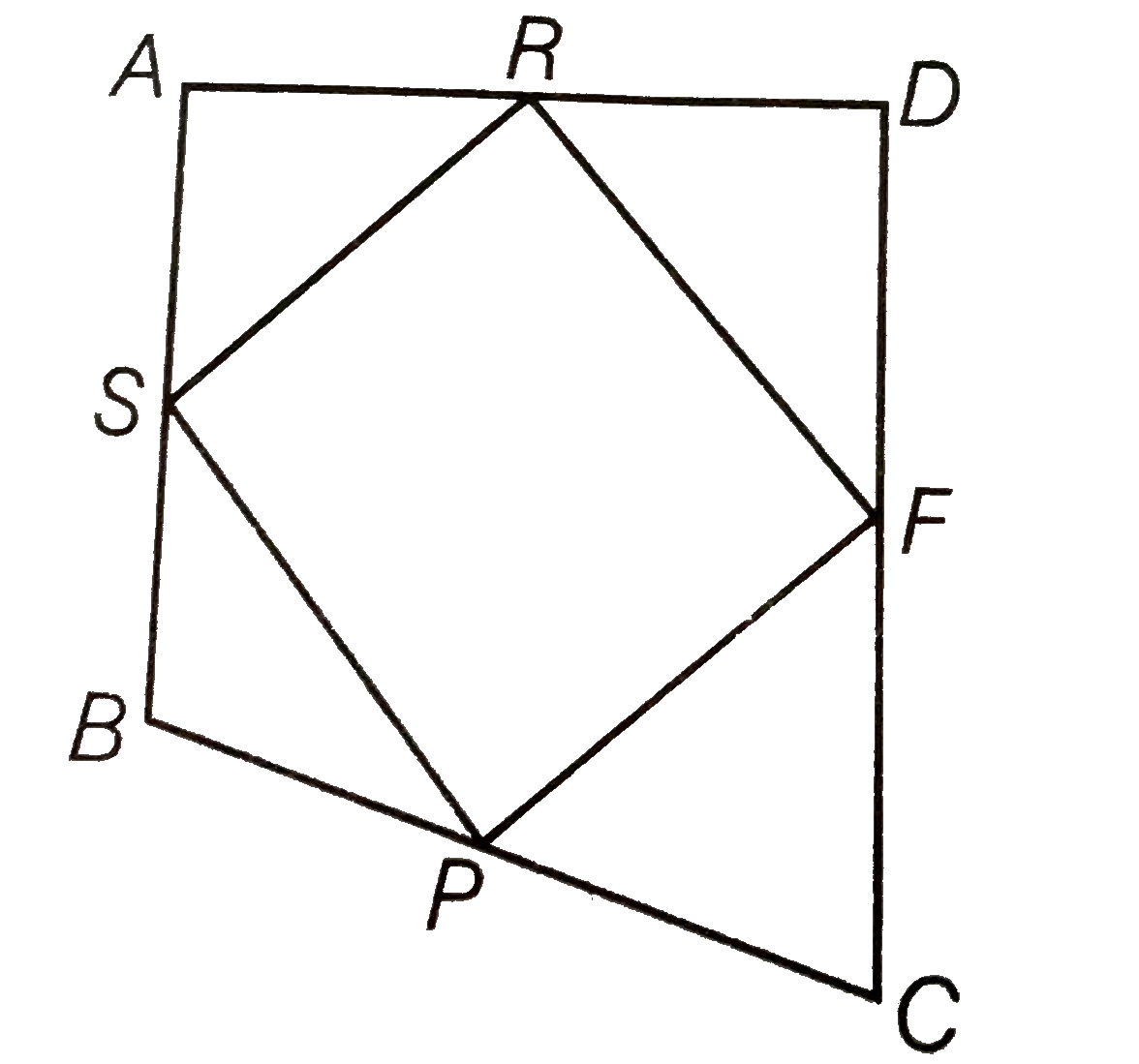
View SolutionIf the mid-points of the sides of a quadrilateral are joined in order, prove that the area of the parallelogram, so formed will be half of the area of the given quadrilateral (figure).
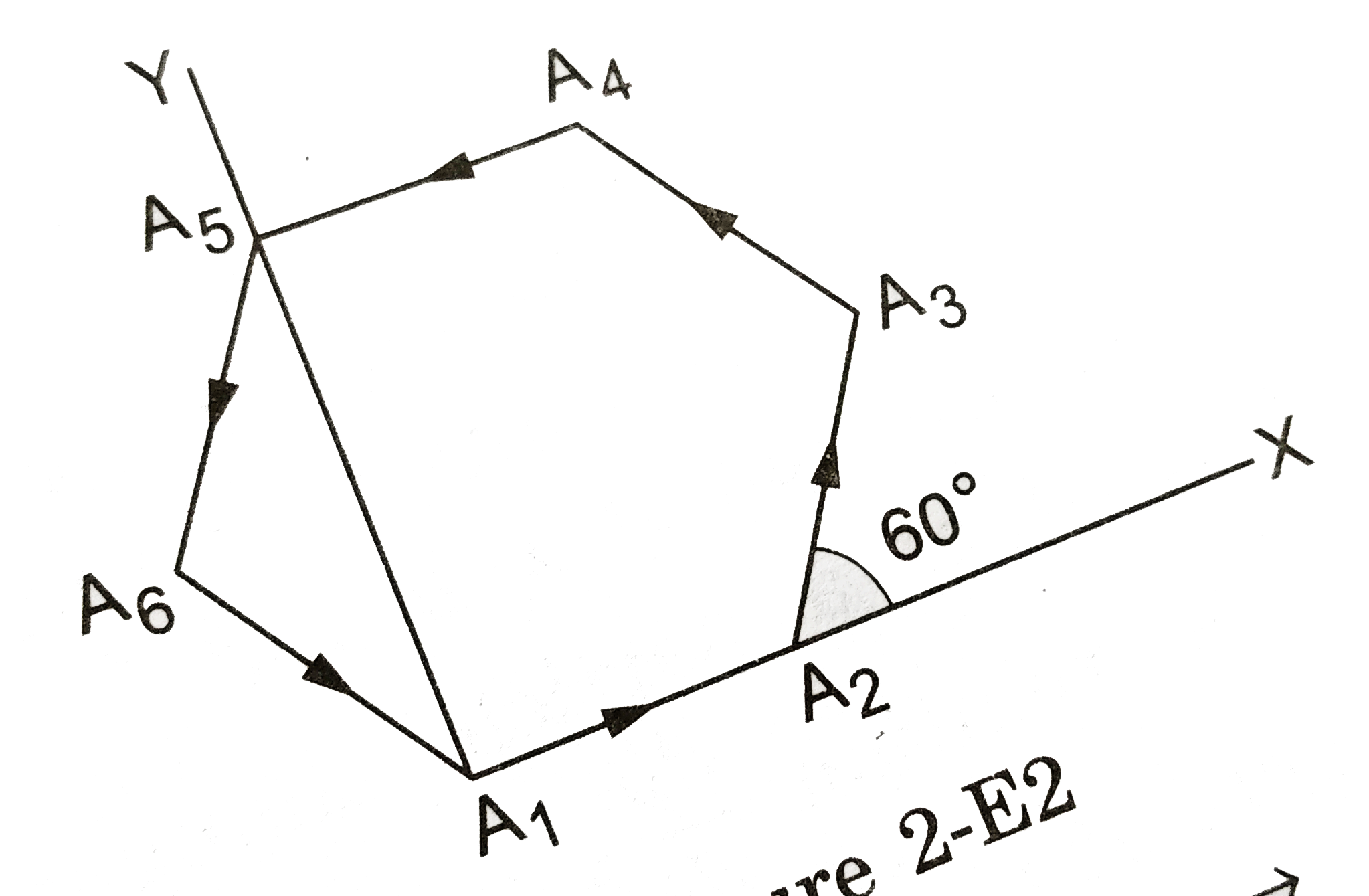
View SolutionLet A1A2A3A4A5A6A1 be a regular hexagon. Write the x-components of the vectors representeed by the six sides taken in order. Use the fact that the resultant of these six vectors is zero, to prove that cos0+cosπ3+cos(2π)3+cos(4π)3+cos(5π)3=0 Use the known cosine values of verify the result.
 .
.
View Solution
