The plane xa+yb+zc=1 meets the co-ordinate axes in the point A, B, C respectively. Find the
area △ABC.
To find the area of triangle ABC formed by the intersection of the plane xa+yb+zc=1 with the coordinate axes, we can follow these steps:
Step 1: Find the coordinates of points A, B, and C
The plane intersects the coordinate axes at the following points:
- Point A (where the plane intersects the x-axis): Set y=0 and z=0:
xa=1⟹x=a⟹A(a,0,0)
- Point B (where the plane intersects the y-axis): Set x=0 and z=0:
yb=1⟹y=b⟹B(0,b,0)
- Point C (where the plane intersects the z-axis): Set x=0 and y=0:
zc=1⟹z=c⟹C(0,0,c)
Step 2: Use the formula for the area of a triangle in 3D
The area A of triangle ABC can be calculated using the formula:
Area=12∥→AB×→AC∥
where →AB and →AC are the vectors from A to B and from A to C, respectively.
Step 3: Calculate the vectors →AB and →AC
- Vector →AB=B−A=(0,b,0)−(a,0,0)=(−a,b,0)
- Vector →AC=C−A=(0,0,c)−(a,0,0)=(−a,0,c)
Step 4: Compute the cross product →AB×→AC
Using the determinant method for the cross product:
→AB×→AC=∣∣
∣
∣∣^i^j^k−ab0−a0c∣∣
∣
∣∣
Calculating the determinant:
=^i∣∣∣b00c∣∣∣−^j∣∣∣−a0−ac∣∣∣+^k∣∣∣−ab−a0∣∣∣
=^i(bc)−^j(−ac)+^k(ab)
=bc^i+ac^j+ab^k
Step 5: Find the magnitude of the cross product
The magnitude is given by:
∥→AB×→AC∥=√(bc)2+(ac)2+(ab)2
Step 6: Calculate the area of triangle ABC
Now substituting back into the area formula:
Area=12∥→AB×→AC∥=12√(bc)2+(ac)2+(ab)2
Final Answer
Thus, the area of triangle ABC is:
Area=12√(bc)2+(ac)2+(ab)2
xa=1⟹x=a⟹A(a,0,0)
yb=1⟹y=b⟹B(0,b,0)
zc=1⟹z=c⟹C(0,0,c)
Area=12∥→AB×→AC∥
where →AB and →AC are the vectors from A to B and from A to C, respectively.
- Vector →AC=C−A=(0,0,c)−(a,0,0)=(−a,0,c)
→AB×→AC=∣∣
∣
∣∣^i^j^k−ab0−a0c∣∣
∣
∣∣
=^i∣∣∣b00c∣∣∣−^j∣∣∣−a0−ac∣∣∣+^k∣∣∣−ab−a0∣∣∣
=^i(bc)−^j(−ac)+^k(ab)
=bc^i+ac^j+ab^k
∥→AB×→AC∥=√(bc)2+(ac)2+(ab)2
Area=12∥→AB×→AC∥=12√(bc)2+(ac)2+(ab)2
Thus, the area of triangle ABC is:
Area=12√(bc)2+(ac)2+(ab)2
Topper's Solved these Questions
- THREE DIMENSIONAL GEOMETRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - Illustration
4 Videos
- THREE DIMENSIONAL GEOMETRY BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRY EXERCISE - TRY YOURSELF
97 Videos
- STRAIGHT LINESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - SECTION-J (AAKASH CHALLENGERS QUESTIONS)
5 Videos
- TRIGNOMETRIC FUNCTIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TRIGNOMETRIC FUNCTIONS EXERCISE - Section - J (Akash Challengers Question)
16 Videos
Similar Questions
Knowledge Check
A plane meets the coordinate axes at A, B and C respectively such that the centroid of triangle ABC is (p, q, r). The equation of the plane is
Axp+yq+zr=1Bxp+yp+zr=13Cpz+qy+rz=3Dxp+yq+zr=3
If the plane x2+y3+z6=1 cuts the axes of coordinates at points, A,B, and C, then find the area of the triangle ABC. a. 18 sq unit b. 36 sq unit c. 3√14 sq unit d. 2√14 sq unit
View SolutionIf the plane x2+y3+z4=1 cuts the coordinate axes in A,B,C, then the area of triangle ABC is
View SolutionThe plane x2+y3+z4=1 cuts the co-ordinate axes in A,B,C: then the area of the ΔABC is
View SolutionA plane meets the coordinate axes at A,BandC respectively such that the centroid of triangle ABC is (1,−2,3). Find the equation of the plane.
View SolutionA variable plane at a constant distance p from the origin meets the coordinate axes in points A,B and C respectively.Through these points , planes are drawn parallel to the coordinate planes, show that locus of the point of intersection is 1x2+1y2+1z2=1p2
View SolutionThe given figure shows a triangle ABC with ∠BAC=56∘and∠ABC=64∘, Bisectors of angles A,B and C meet the circumcircle of the ΔABC at points P,Q and R respectively . Find the measure of ∠QPR.
View SolutionThe line xa+yb=1 meets the axis of and y at A and B respectively and C is middle point of AB then
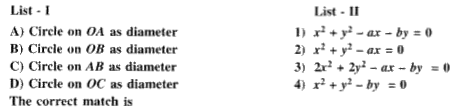
View Solution
