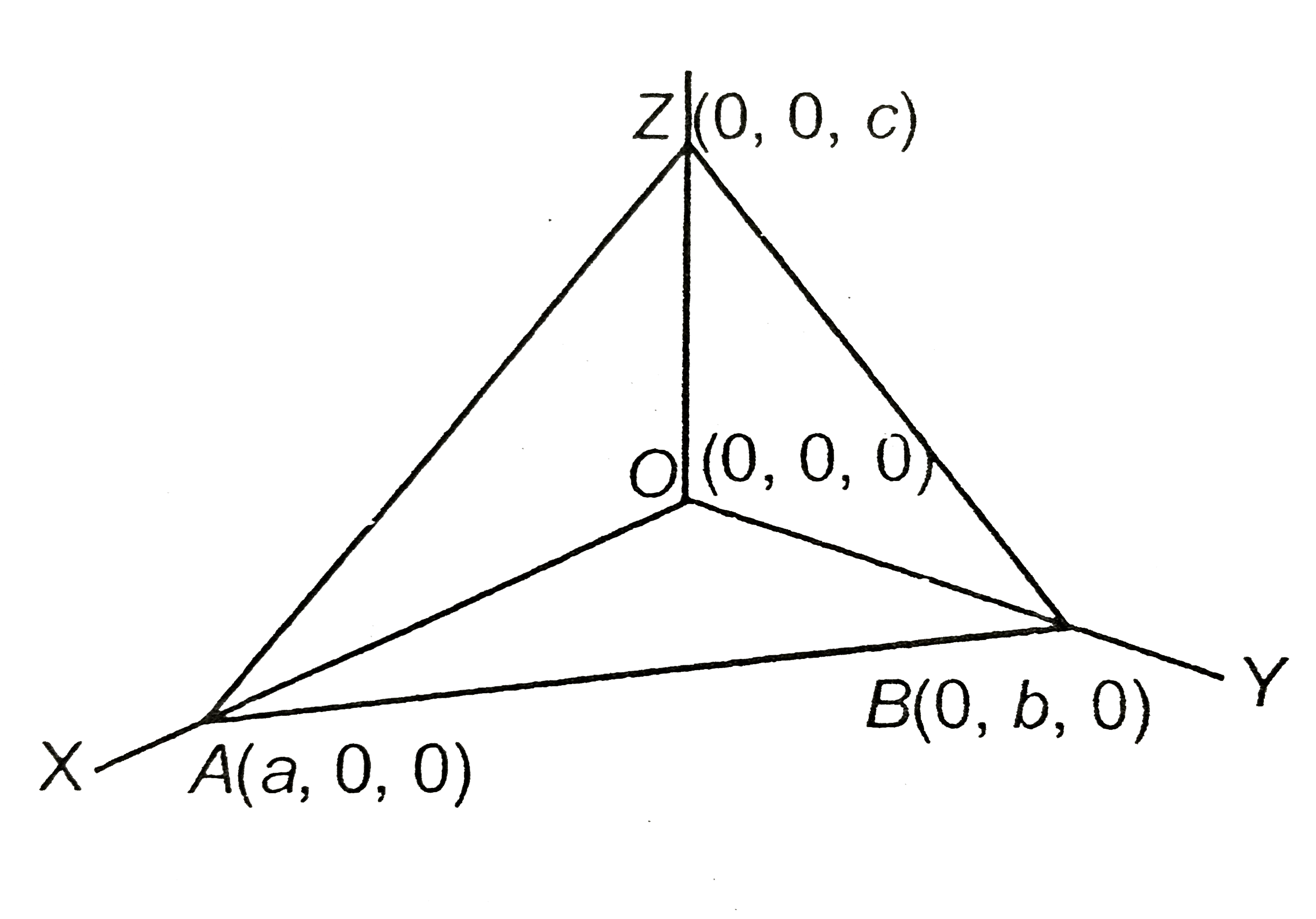
A variable plane which remains at q constant distance $3 p$ from the origin cut the coordinate axes at $A, B, C .$ Show that the locus o the centroid of triangle $A B C$ is $x^{- 2} + y^{- 2} + z^{- 2} = p^{- 2} .$

Video Solution

Text Solution

Verified by Experts

Let a variable plane cuts the coordinate axes in A(a, 0, 0), B(0, b, ) and C (0, 0, c) and
$(α, β, γ)$ be the coordinates of centroid of the terahedron OABC.
$∴ α = \frac{a}{4}, β = \frac{b}{4}, γ = \frac{c}{4}$
$\Rightarrow a = 4 α, b = 4 β, c = 4 γ$
Since the plane $\frac{x}{a} + \frac{y}{b} + \frac{z}{c} = 1$ is at a constant
distance p from origin, hence
$p = \frac{0 + 0 + 0 - 1}{\sqrt{\frac{1}{a^{2}} + \frac{1}{b^{2}} + \frac{1}{c^{2}}}}$
$\Rightarrow \frac{1}{p^{2}} = \frac{1}{a^{2}} + \frac{1}{b^{2}} + \frac{1}{c^{2}}$
$\Rightarrow \frac{1}{p^{2}} = \frac{1}{{(4 α)}^{2}} + \frac{1}{{(4 β)}^{2}} + \frac{1}{{(4 γ)}^{2}} = \frac{1}{16 α^{2}} + \frac{1}{16 β^{2}} + \frac{1}{16 γ^{2}}$
Locus of $(α, β, γ)$ is
$\frac{16}{p^{2}} = \frac{1}{x^{2}} + \frac{1}{y^{2}} + \frac{1}{z^{2}}$
$\Rightarrow x^{- 2} + y^{- 2} + z^{- 2} = 16 p^{_2}$

Updated on:21/07/2023

|

Class 12 MATHS THREE DIMENSIONAL GEOMETRY

Topper's Solved these Questions

Similar Questions

AAKASH INSTITUTE ENGLISH-THREE DIMENSIONAL GEOMETRY -ASSIGNMENT SECTION - J

Home
Ask Doubt
Profile