Question

Prove that the points $A(4,0),B(6,1),C(4,3)$ and D(3, 2) are concyclic.

Explanation

Our strategy would be to show that `D(3, 2)` lies on the circumcircle of `triangleABC`.
Note that the points A(4, 0) and C(4, 3) have the same x-coordinates, viz x = 4.
Thus AC is parallel to y-axis.
`therefore` The perpendicular bisector of AC through `M_(1)(4, (3)/(2))` is perpendicular to y-axis.
hence y-coordinates S of triangle ABC is `(3)/(2)`.

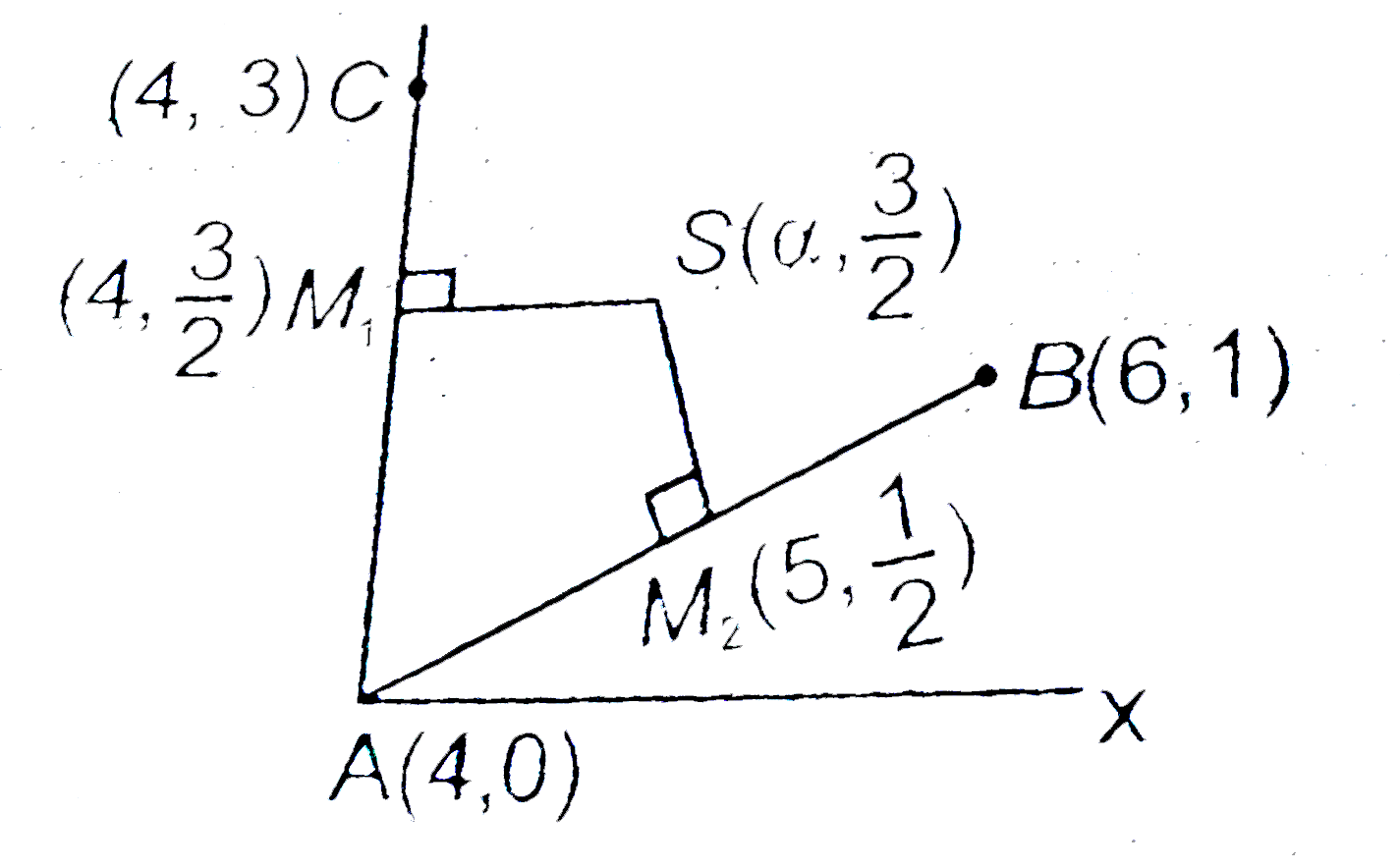
Let S be `(alpha, (3)/(2))` now `M_(2)` is mid-point of AB and `M_(2)S` perpendicular ot AB. (The centre of a circle is the point of intersection of two perpendicular bisectors of the non-parallel chords).
`rArr((3)/(2)-(1)/(2))/(alpha-5)*(1-0)/(6-4) = -1" " rArr(1)/(alpha-5)*(1)/(2)=-1`
`rArr alpha - 5 = -(1)/(2)`
`rArr alpha = 5-(1)/(2) " " therefore alpha = (9)/(2)`
Thus S is `((9)/(2),(3)/(2))`.
`SA = sqrt((alpha-4)^(2)+((3)/(2))^(2))=sqrt(((9)/(2)-4)^(2)+((3)/(2))^(2))=sqrt(((1)/(2))^(2)+((3)/(2))^(2))`
`=sqrt((10)/(4))=sqrt((5)/(2))`
`SA = sqrt((5)/(2))`, therefore the circumcircle of `triangleABC` has the centre `((9)/(3),(3)/(2))` and radius `sqrt((5)/(2))`. Its equation then is
`(x-(9)/(2))^(2)+(y-(3)/(2))^(2)=(5)/(2)......(A)`
The point D( 3, 2) lies on (A) - one can check it easily. Thus points A, B, C, D are concyclic