mn squares of the equal size are arranged to form a rectangle of dmensin mbyn,wheremandn are natural number. Two square will be called neighbours if they have exactly one common side. A number is written in each square such that the number written in any square is the arithmetic men of the numbers written in its neighboring squares. Show that this is possible only if all the numbers used are equal.
Let mn squares of equal size are arranged to form a rectangle of dimensions m and n shown in the figure. The neighbiur of x1 are x2,x3,x4,x5 .
Similarly neighbours of x5 are x6,x1 and x7 and that of x7 are x5,x4 .
From the guven condition ,
x1=x2+x3+x4+x54
⇒4x2=x2+x3+x4+x5
Also X5=x1+x6+x73
⇒3x5=x1+x6+x7andx7=x4+x52
⇒2x7=x4+x5
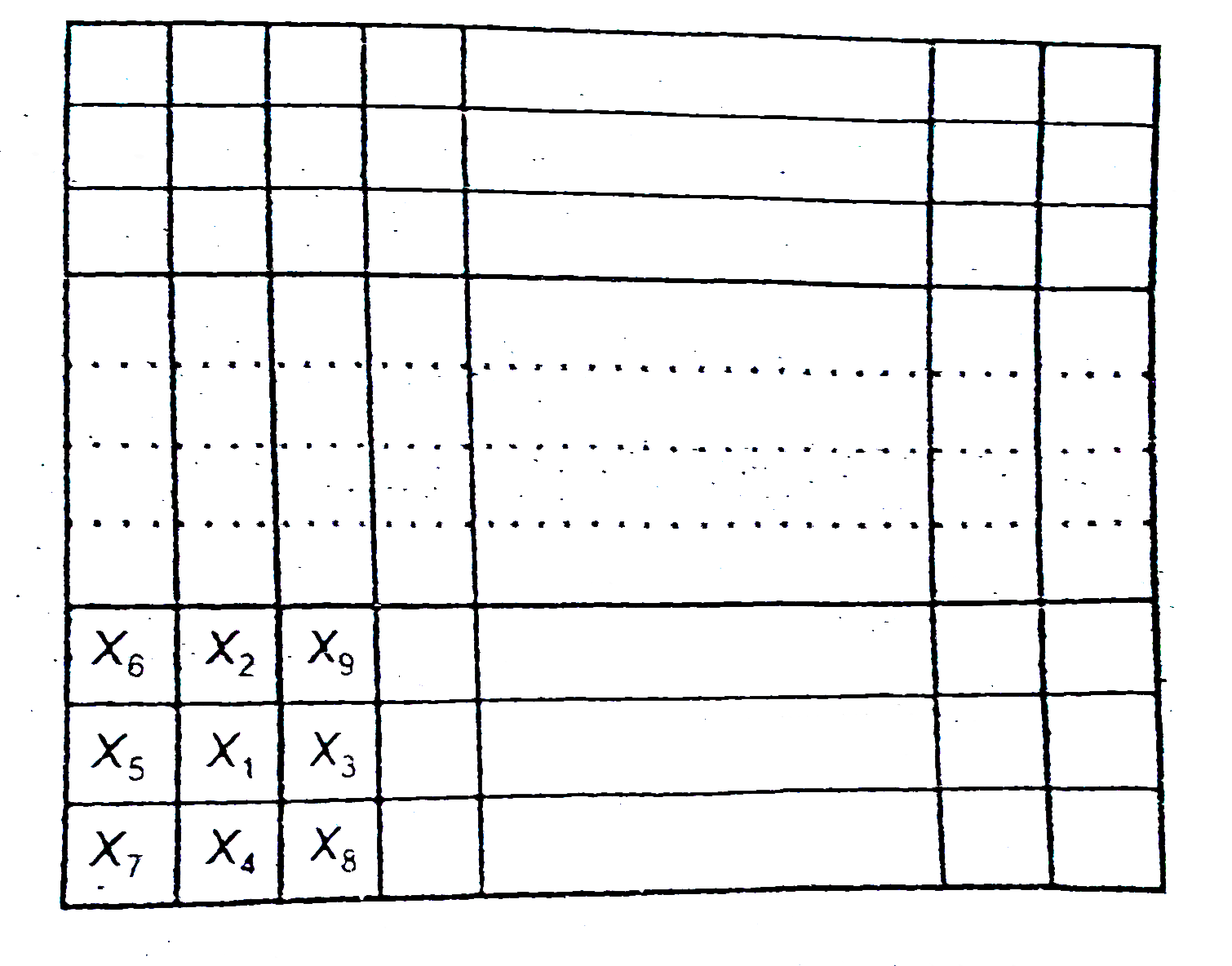
∴4x1=x2+x3+x4+x1+x6+x73
⇒12x1+3x2=3x3+3x4=x1=x6+x7
=3x2+3x3+3x4+x1+x6+x4+x52
⇒24x1=6x2+6x3+7x4+2x1+2x6+x5
⇒22x1=6x2+6x3+7x4+x5+2x6
Where x1,x2,x3,x4,x5,x6 are all natural numbers and x1 is linearly expressed as the sum of x2,x3,x4,x5andx6 where the sum of coefficients is equal to the coefficient of x1.
i.e., 22=6+6+7+1+2
Which will happen only when
x1=x2=x3=x4=x5=x6
⇒ all the numbers used are equal .
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Try Yourself
65 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section A Objective type questions (One option is correct )
51 Videos
- MATRICESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MATRICESEXERCISE - Assignment (Section - J) Aakash Challengers Questions
3 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
Similar Questions
mn squares of equal size are arranged to form a rectangle of dimension m by n, where m and n are natural numbers. Two square will be called neighbors if they have exactly one common side. A number is written in each square such that the number written in any square is the arithmetic mean of the numbers written in its neighboring squares. Show that this is possible only if all the numbers used are equal.
View SolutionThe arithmetic mean of the squares of first n natural numbers is
View SolutionFind the squares of the number127
View SolutionThe arithmetic mean of two numbers is 1834 and the positive square root of their product is 15. The larger of the two numbers is
View SolutionThe sum of a number and its positive square root is 6/25.Find the number.
View SolutionFind the number of ways of selecting pair of black squares in chessboard such that they have exactly one common corner
View SolutionFind the smallest square number that is divisible by each of the numbers 8, 15 and 20.
View SolutionFind the smallest square number that is divisible by each of the numbers 4, 9 and 10.
View SolutionFind the square roots of the numbers by expressing each number as the product of primes :
196
View SolutionFind the square roots of the numbers by expressing each number as the product of primes :
676
View Solution
