Find the highest power of 5 in (100)!
To find the highest power of 5 in 100!, we can use the formula for determining the highest power of a prime p in n!:
Highest power of p in n!=⌊np⌋+⌊np2⌋+⌊np3⌋+…
where ⌊x⌋ denotes the greatest integer less than or equal to x.
1. Identify n and p:
- Here, n=100 and p=5.
2. Calculate ⌊1005⌋:
⌊1005⌋=⌊20⌋=20
3. Calculate ⌊10052⌋:
⌊10025⌋=⌊4⌋=4
4. Calculate ⌊10053⌋:
⌊100125⌋=⌊0.8⌋=0
5. Sum the results:
Highest power of 5 in 100!=20+4+0=24
Thus, the highest power of 5 in 100! is 24.
- Here, n=100 and p=5.
⌊1005⌋=⌊20⌋=20
⌊10025⌋=⌊4⌋=4
⌊100125⌋=⌊0.8⌋=0
Highest power of 5 in 100!=20+4+0=24
Topper's Solved these Questions
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Try Yourself
65 Videos
- PERMUTATIONS AND COMBINATIONS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PERMUTATIONS AND COMBINATIONS EXERCISE - Assignment Section A Objective type questions (One option is correct )
51 Videos
- MATRICESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MATRICESEXERCISE - Assignment (Section - J) Aakash Challengers Questions
3 Videos
- PRINCIPLE OF MATHEMATICAL BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - PRINCIPLE OF MATHEMATICAL EXERCISE - Section-D:(Assertion-Reason Type Questions)
11 Videos
Similar Questions
Knowledge Check
An equation with one variable in which the highest power of the variable is two is known as ______
ALinear equationBQuadratic equationCCubic equationDNone of theseTwo real numbers or two algebraic expressions related by the symbols >,<,≤or≥ form an inequation. If the highest power of the variables used in the inequation is 1, then the inequation is called linear inequation. For the linear inequation 3x−5≤8, answer teh following questions.
If x∈R, then the highest value of x is:A3B413C4D5Two real numbers or two algebraic expressions related by the symbols >,<,≤or≥ form an inequation. If the highest power of the variables used in the inequation is 1, then the inequation is called linear inequation. For the linear inequation 3x−5≤8, answer teh following questions.
If x∈I, then the highest value of x isA3B4C5D413
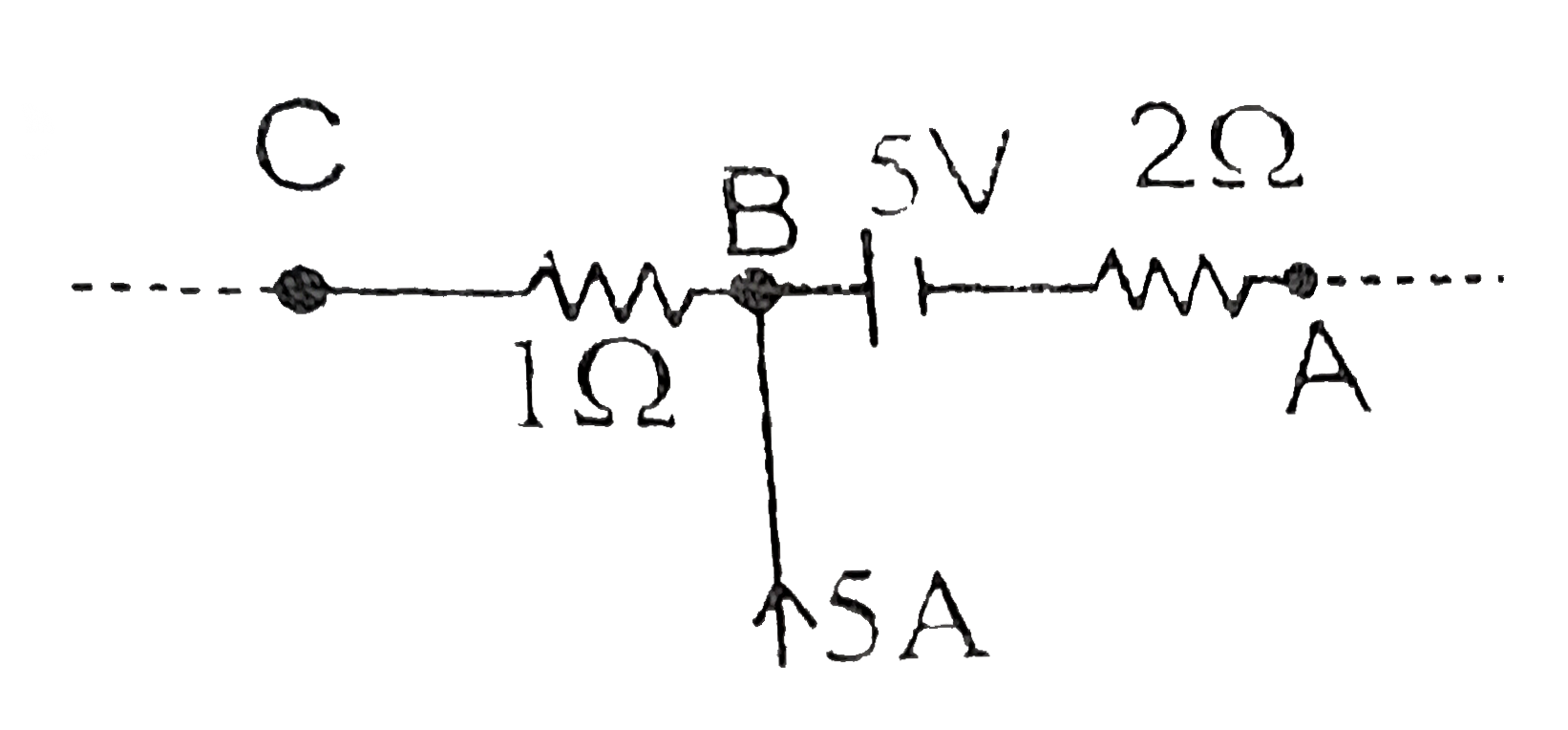
If the cell of emf 5 volt shown in the figure gives a power of 10W. Q, find the powers consumed by the resistors 2Ω and 1Ω
View SolutionThe focal lengths of a convex lens for red, yellow and violet rays are 100 cm, 98 cm and 96 cm respectively. Find the dispersive power of the material of the lens.
View SolutionTransform each of the following equations into ones in which of the coefficients of the second highest power of x is zero and also find their transformed equations x4+4x3+2x2−4x−2=0
View SolutionFind the greater number is 100100 and (300)!.
View SolutionIn LC oscillation resistance is 100 Ω and inductance and capacitance is 1 H and 10μF . Find the half power of frequency .
View Solution
