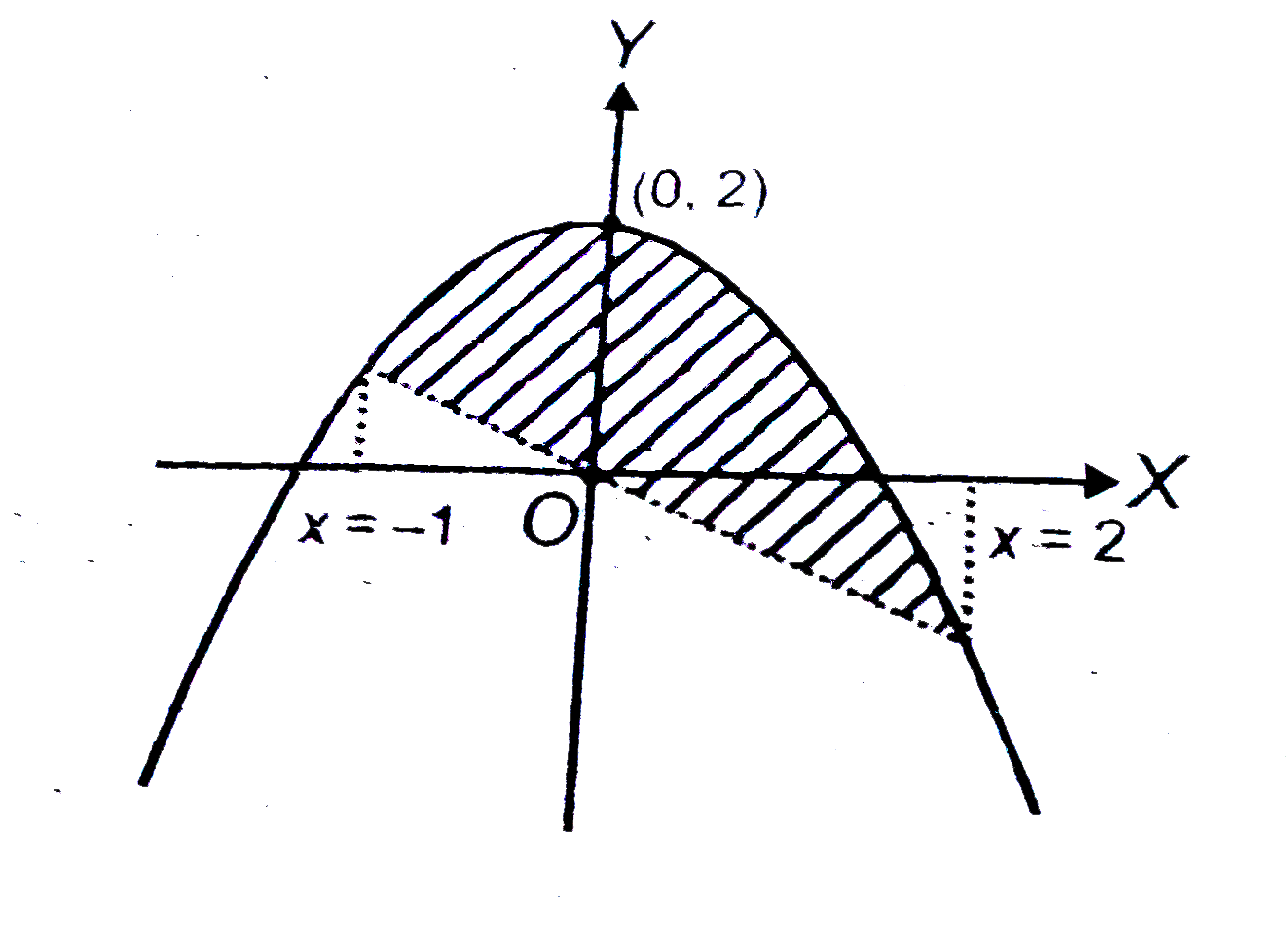
Find the area bounded by the parabola y=2−x2 and the straight line y+x=0.
By solving y=2−x2 and y + x = 0 we get x=−1,2
A=∫2−1[f(x)−g(x)] dx
=∫2−1[2−x2+x] dx
=[2x]2−1−[x23]2−1+[x22]2−1
=132 sq. units
Topper's Solved these Questions
- APPLICATION OF INTEGRALS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF INTEGRALS EXERCISE - Try Yourself
4 Videos
- APPLICATION OF INTEGRALS BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF INTEGRALS EXERCISE - Assignment Section - A Competition Level Questions
24 Videos
- APPLICATION OF DERIVATIVESBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - Assignment SECTION-J (Aakash Challengers Questions )
8 Videos
- BINOMIAL THEOREMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Assignment (section-J) Objective type question (Aakash Challengers Questions)
4 Videos
Similar Questions
Find the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y=x2+1 and the straight line x+y=3.
View SolutionFind the area bounded by the parabola y2=4x and the straight line x+y=3.
View SolutionFind the area bounded by the curves y=2x−x2 and the straight line y=−x.
View SolutionFind the area bounded by the curve x2=4y and the straight line x=4y−2.
View SolutionFind the area bounded by the curve x2=4y and the straight line x=4y−2.
View SolutionFind the area of the region bounded by the parabola y2=2x and straight line x−y=4.
View SolutionFind the area enclosed between the parabola 4y=3x2 and the straight line 3x−2y+12=0
View SolutionFind the area enclosed between the parabola 4y=3x2 and the straight line 3x−2y+12=0
View Solution
