Let $f (x) = {sin}^{- 1} 2 x + {cos}^{- 1} 2 x + {sec}^{- 1} 2 x$ . Then the sum of the maximum and minimum values of f(x) is

A

$π$

B

$2 π$

C

$3 π$

D

$\frac{π}{2}$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:B

$f (x) = {sin}^{- 1} 2 x + {cos}^{- 1} 2 x + {sec}^{- 1} 2 x$
$f (x) = \frac{π}{2} + {sec}^{- 1} 2 x$
$f (x) = \frac{π}{2} + {sec}^{- 1} 2 x$
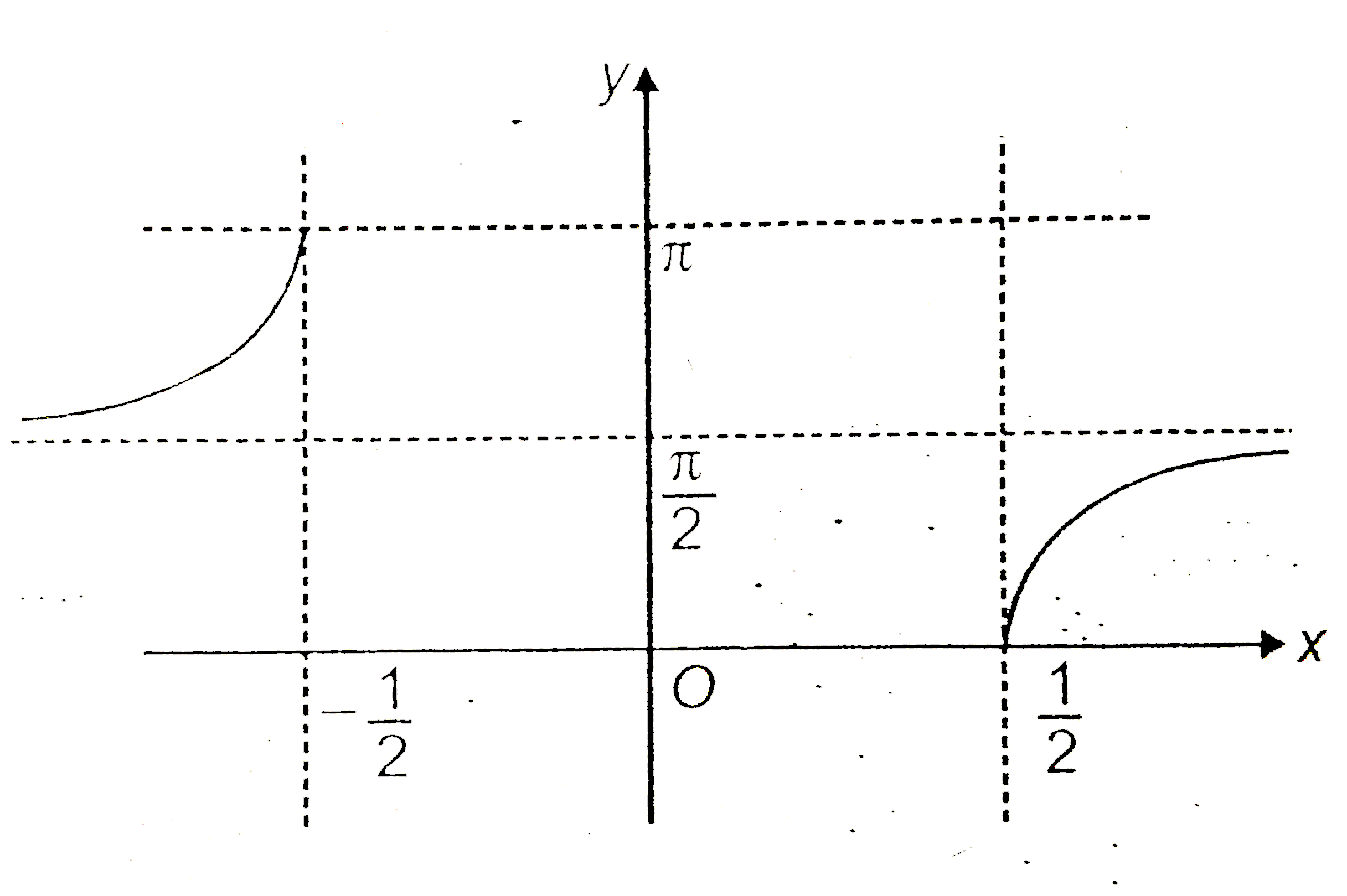
Graph of ${sec}^{- 1} 2 x$ is as following
${(f (x))}_{minimum} = \frac{π}{2} + 0 = \frac{π}{2}$
${(f (x))}_{minimum} = \frac{π}{2} + π = \frac{3 π}{2}$
Sum $= \frac{π}{2} + \frac{3 π}{2}$
$2 π$

Updated on:21/07/2023

|

Class 12 MATHS INVERSE TRIGONOMETRIC FUNCTIONS

Topper's Solved these Questions

Similar Questions

AAKASH INSTITUTE ENGLISH-INVERSE TRIGONOMETRIC FUNCTIONS-ASSIGNMENT (SECTION - J)(ANKASH CHALLENGERS QUESTIONS)

Home
Ask Doubt
Profile