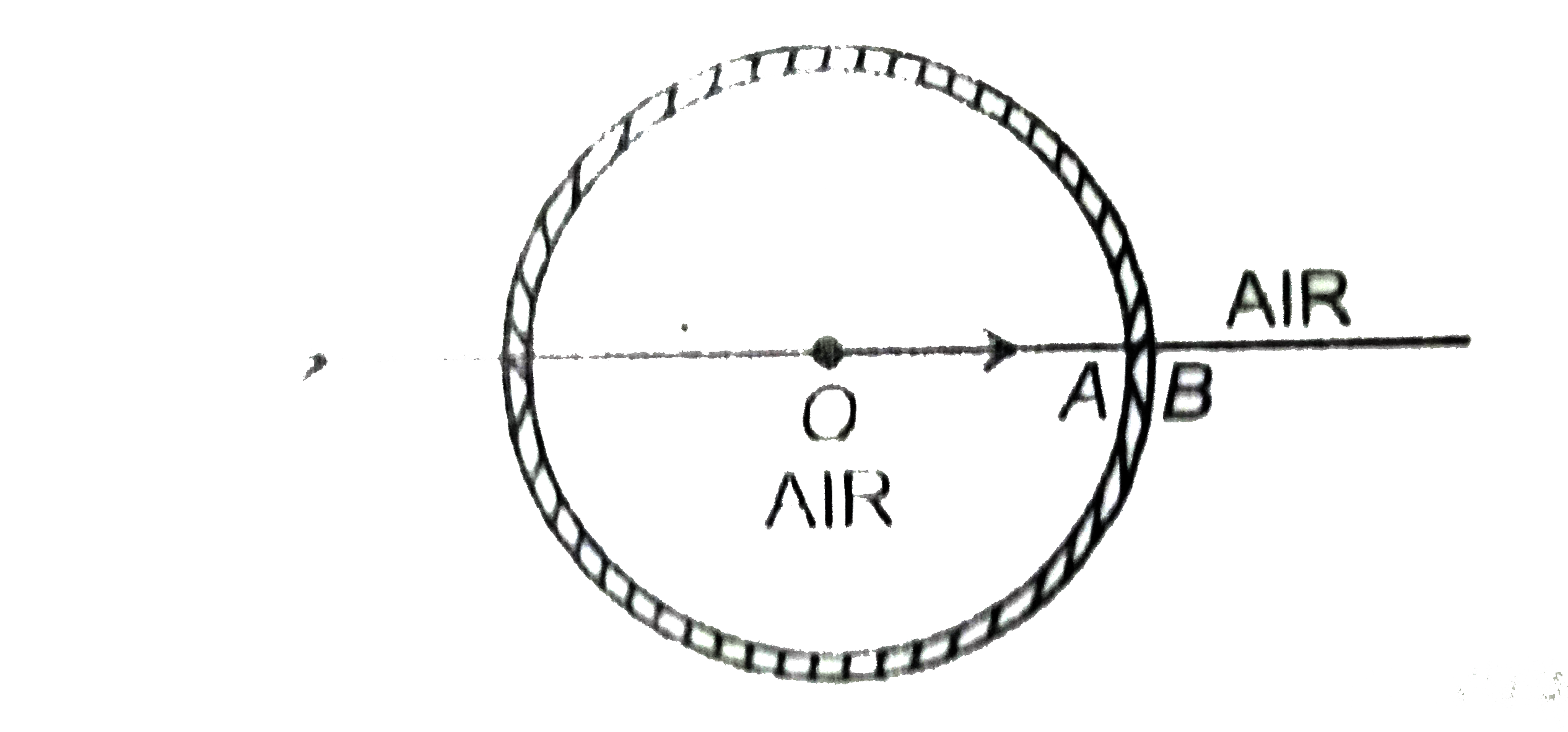
A small filament is at the centre of a hollow glass sphere of inner and outer radii 8 cm and 9 cm respectively. The refractive index of glass is 1.50. Calculate the position of the image of the filament when viewed from outside the sphere.

Video Solution

Text Solution

Verified by Experts

For refraction at the first surface, $μ_{1} = 1, μ_{2} = 1.50, u = - 8 c m$
$R = - c m$
$\frac{μ_{2}}{v} - \frac{μ_{1}}{u} = \frac{μ_{2} - μ_{1}}{R}$
$\frac{1.5}{v'} - \frac{100}{- 8 c m} = \frac{1.50 - 1.00}{- 8 c m}$
v = -8 cm
It means that due to the first surface the image is formed at the centre of the sphere.
For the second surface, $μ_{1} = 1.50, μ_{2} = 1, u = - 9 c m, R = - 9 c m$
$\frac{μ_{2}}{v} - \frac{μ_{1}}{u} = \frac{μ_{2} - μ_{1}}{R}$
$\frac{1}{v} - \frac{1.50}{- 9 c m} = \frac{1 - 1.50}{- 8 c m}$
v = -9 cm
Hence, the final image is formed at the centre of the sphere.

Updated on:21/07/2023

|

Class 12 PHYSICS GEOMETRICAL OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

A mark placed on the surface of a sphere is viewed through glass from a position directly opposite. If the diameter of the sphere is $10 c m$ and refractive index of glass is $1.5$ , find the position of the image.
A $- 20 c m$
B $30 c m$
C $40 c m$
D $- 10 c m$

AAKASH INSTITUTE ENGLISH-RAY OPTICS AND OPTICAL INSTRUMENTS-ASSIGNMENT (SECTION - D)

Home
Ask Doubt
Profile