represents a
wave travelling in the negative direction of x-axis
Wave travelling in the positive direction of x-axis
stationary wave
combination of two stationary waves
The correct Answer is:B
Step by step video & image solution for y(xt)=asin(kx-omegat+phi) represents a by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-B)35videos
WAVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Assignment (Section-C)11videos
WAVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVESEXERCISE - Try Yourself65videos
WAVES
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WAVE OPTICSEXERCISE - Assignment (Section-J (Aakash Challengers question))1videos
WAVE OPTICS
- BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - WORK, ENERGY AND POWEREXERCISE - Assignment (SECTION - D)13videos
WORK, ENERGY AND POWER
Similar Questions
The equation y=Asin2(kx−ωt) represents a wave motion with
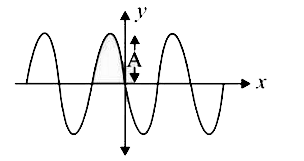
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:
 View Solution
View SolutionA progressive wave travelling along the positive x-direction is represented by y(x,t)=Asin(kx−ωt+ϕ). Its snapshot at t=0 is given in the figure.
For this wave, the phase is:
 View Solution
View SolutionIn a wave motion y=asin(kx−ωt),y can represent :-
View SolutionThe equation of a light wave is written as y=Asin(kx−ωt). Here y represents
View SolutionIn a wave motion y=asin(kx−ωt), y can represent
View SolutionA particle undergoing SHM has the equation x=Asin(2ωt+ϕ) , where x represents the displacement of the particle. The kinetic energy oscillates with time period
View SolutionLet y=Asin(ωt−kx) represent the variation of distance y of a particle with time t. Which of the following is not meaningful?
View SolutionThe function sin2(ωt) represents.
View SolutionA wave is represente by y=Asin2(kx−ωt+ϕ). The amplitude and wavelength of wave is given by
View Solution


