Three bodies A, B and C having masses 10kg, 5kg and 15kg respectively are projected from top of a tower with A vertically upward with 10m/s, B with 20m/s53∘ above east horizontal and C horizontally southward with 15m/s. Find
(a) Velocity of centre of mass of the system.
(b)Acceleration of centre of mass of the system.
To solve the problem, we will break it down into two parts: finding the velocity of the center of mass and finding the acceleration of the center of mass.
Given:
- Mass of A, mA=10kg
- Mass of B, mB=5kg
- Mass of C, mC=15kg
Velocities:
- Velocity of A, →VA=10^jm/s (upward)
- Velocity of B, →VB=20m/s at 53∘ above the east horizontal
- Resolving →VB:
→VB=20cos(53∘)^i+20sin(53∘)^j
→VB=20(35)^i+20(45)^j=12^i+16^jm/s
- Velocity of C, →VC=−15^jm/s (southward)
(a) Velocity of the Center of Mass
The velocity of the center of mass →VCM is given by the formula:
→VCM=mA→VA+mB→VB+mC→VCmA+mB+mC
Calculating the total mass:
mtotal=mA+mB+mC=10+5+15=30kg
Calculating the numerator:
mA→VA=10⋅10^j=100^jkg m/s
mB→VB=5⋅(12^i+16^j)=60^i+80^jkg m/s
mC→VC=15⋅(−15^j)=−225^jkg m/s
Now, summing these:
Total=(60^i+(100+80−225)^j)=60^i−45^jkg m/s
Now, substituting into the center of mass velocity formula:
→VCM=60^i−45^j30=2^i−1.5^jm/s
(b) Acceleration of the Center of Mass
The acceleration of the center of mass →aCM is given by:
→aCM=mA→aA+mB→aB+mC→aCmA+mB+mC
Since all bodies are under the influence of gravity, their accelerations are:
- →aA=−g^j
- →aB=−g^j
- →aC=−g^j
Thus, we can write:
→aCM=mA(−g^j)+mB(−g^j)+mC(−g^j)mA+mB+mC
=(−g)(mA+mB+mC)^jmA+mB+mC=−g^j
Final Answers:
(a) The velocity of the center of mass is:
→VCM=2^i−1.5^jm/s
(b) The acceleration of the center of mass is:
→aCM=−g^jm/s2(where g≈9.81m/s2)
- Mass of A, mA=10kg
- Mass of B, mB=5kg
- Mass of C, mC=15kg
- Velocity of A, →VA=10^jm/s (upward)
- Velocity of B, →VB=20m/s at 53∘ above the east horizontal
- Resolving →VB:
→VB=20cos(53∘)^i+20sin(53∘)^j
→VB=20(35)^i+20(45)^j=12^i+16^jm/s
- Velocity of C, →VC=−15^jm/s (southward)
The velocity of the center of mass →VCM is given by the formula:
→VCM=mA→VA+mB→VB+mC→VCmA+mB+mC
mtotal=mA+mB+mC=10+5+15=30kg
mA→VA=10⋅10^j=100^jkg m/s
mB→VB=5⋅(12^i+16^j)=60^i+80^jkg m/s
mC→VC=15⋅(−15^j)=−225^jkg m/s
Total=(60^i+(100+80−225)^j)=60^i−45^jkg m/s
→VCM=60^i−45^j30=2^i−1.5^jm/s
The acceleration of the center of mass →aCM is given by:
→aCM=mA→aA+mB→aB+mC→aCmA+mB+mC
- →aA=−g^j
- →aB=−g^j
- →aC=−g^j
→aCM=mA(−g^j)+mB(−g^j)+mC(−g^j)mA+mB+mC
=(−g)(mA+mB+mC)^jmA+mB+mC=−g^j
(a) The velocity of the center of mass is:
→VCM=2^i−1.5^jm/s
→aCM=−g^jm/s2(where g≈9.81m/s2)
Topper's Solved these Questions
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Illustration
4 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTIONBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - Assignment (Section - A) Objective Type Questions (One option is correct)
62 Videos
- SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - SEMICONDUCTOR ELECTRONICS: MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - Assignment (Section-D (Assertion and reason))
5 Videos
- TEST 1BOOK - AAKASH INSTITUTE ENGLISHCHAPTER - TEST 1EXERCISE - EXERCISE
21 Videos
Similar Questions
If two particles of masses m1 and m2 are projected vertically upwards with speed v1 and v2, then the acceleration of the centre of mass of the system is
View SolutionA particle of mass m is thrown horizontally from the top of a tower and anoher particle of mass 2m is thrown vertically upward. The acceleration of centre of mass is
View SolutionA particle of mass m is thrown horizontally from the top of a tower and anoher particle of mass 2m is thrown vertically upward. The acceleration of centre of mass is
View SolutionTwo particles of masses 2kg and 4kg are approaching towards each other with ccelerations 1m/s2 and 2m/s2, respectively, on a smooth horizontal suface. Then find the acceleration of centre of mass of the system and direction of acceleration of CM.
View SolutionTwo particles A and B of masses 1kg and 2kg respectively are projected in the directions shown in figure with speeds uA=200m/s and uB=50m/s. Initially they were 90m apart. They collide in mid air and stick with each other. Find the maximum height attained by the centre of mass of the particles. Assume acceleration due to gravity to be constant.
(g=10m/s2)

View SolutionA particle of mass 50 g is projected horizontally from the top of a tower of height 50 m with a velocity 20m/s. If g=10m/s2, then find the :-
View SolutionTwo particle of equal mass are projected simultaneously from the roof of a tower of height 20 m with same speed 20m//s, one horizontally and the other vertically upwards. Choose the correct alternative (s).
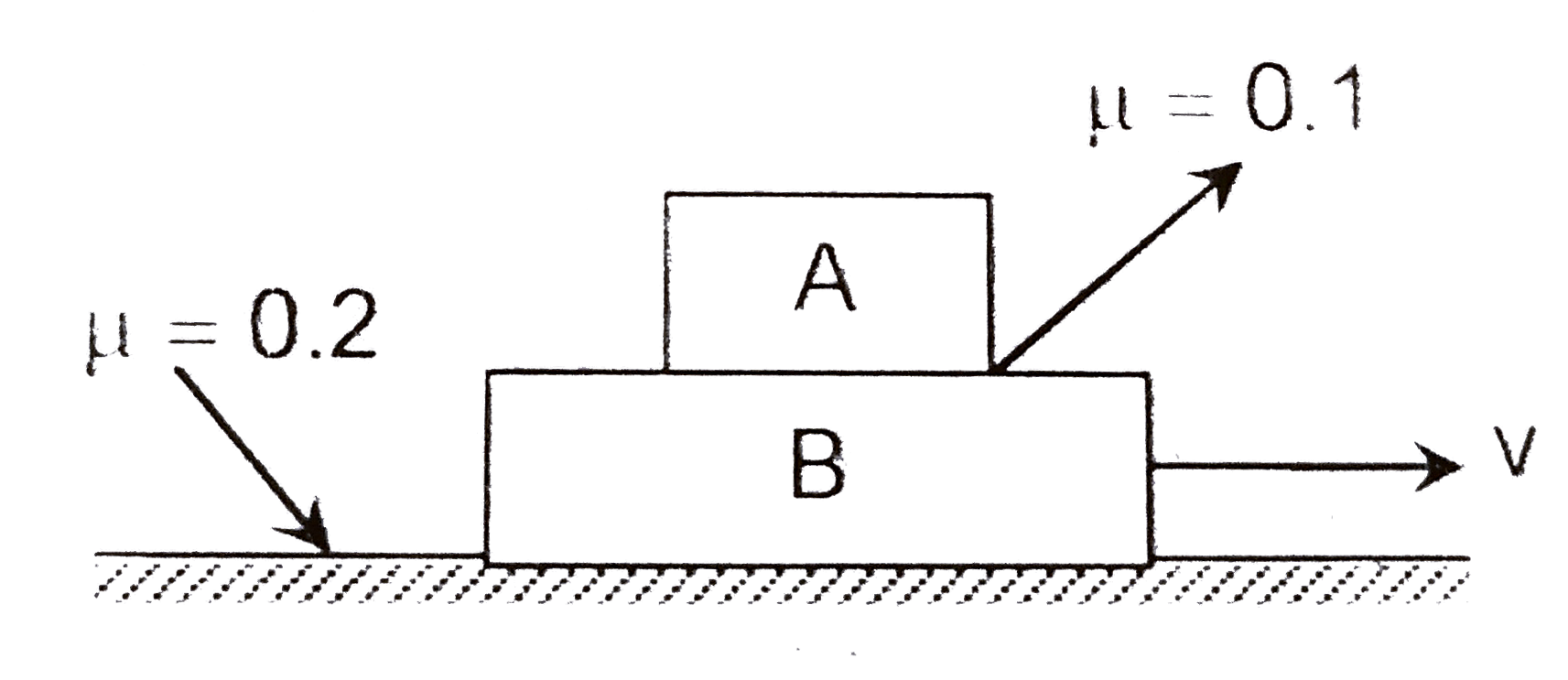
View SolutionBoth the blocks as shown in figure are given together a horizontal velocity towards right. The acceleration of the centre of mass of the system of block is (mA=2mB=2kg).
View SolutionFour particles of masses 1kg, 2kg, 3kg and 4kg are placed at the four vertices A, B, C and D of a square of side 1m. Find the position of centre of mass of the particles.
View SolutionA body is projected horizontally from the top of a tower with a velocity of 10m/s.If it hits the ground at an angle 45∘, then vertical component of velocity when it hits ground in m/s is
View Solution
