Question

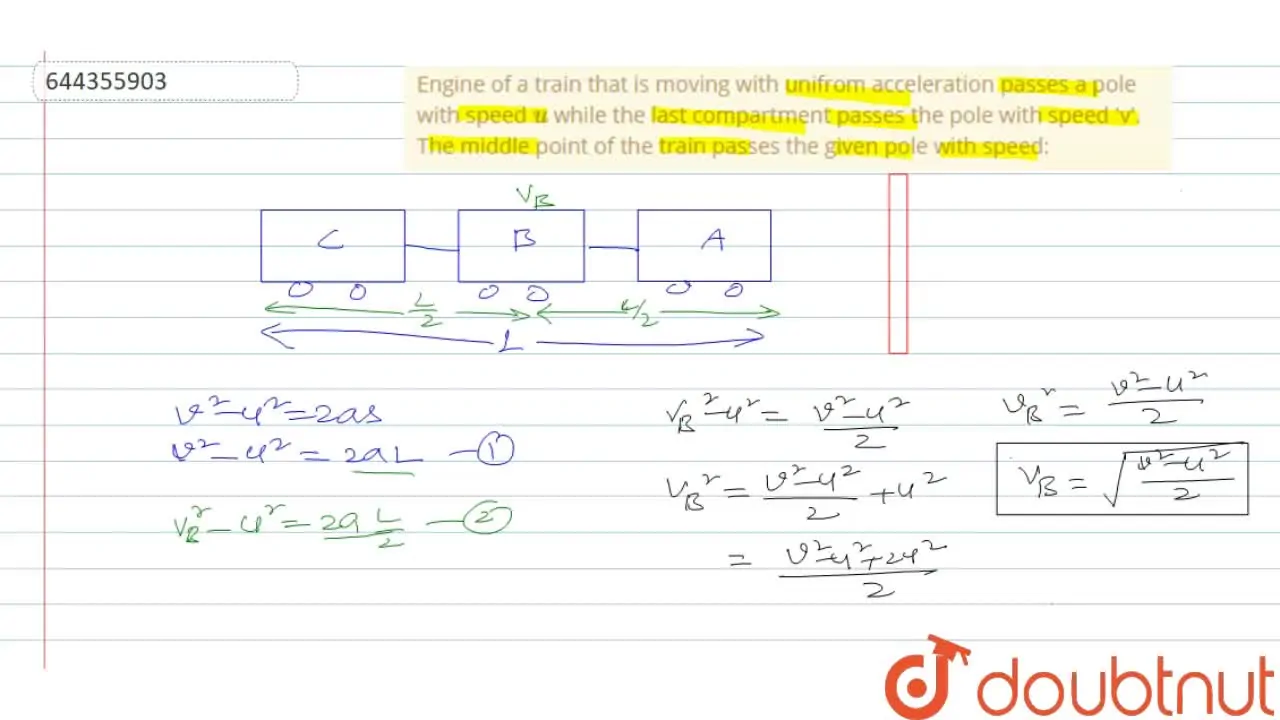
Engine of a train that is moving with unifrom acceleration passes a pole with speed $u$ while the last compartment passes the pole with speed 'v'. The middle point of the train passes the given pole with speed:

Explanation

To solve the problem, we need to find the speed of the middle point of the train as it passes the pole. We know the speed of the engine when it passes the pole is $$u$$ and the speed of the last compartment when it passes the pole is $$v$$.

1. Understanding the Setup:
- Let the length of the train be \( L \).
- The engine (first compartment) passes the pole with speed \( u \).
- The last compartment passes the pole with speed \( v \).
- The middle point of the train is located at \( \frac{L}{2} \) from both the engine and the last compartment.

2. Using the Kinematic Equation:
- We can use the kinematic equation:
\( V^2 = u^2 + 2as \)
- For the last compartment (speed \( v \)), when it passes the pole, the distance \( s \) covered is equal to the length of the train \( L \):
\( v^2 = u^2 + 2aL \quad \text{(Equation 1)} \)

3. Finding the Speed of the Middle Point:
- For the middle point of the train (let's denote its speed as \( V_b \)), when it passes the pole, the distance \( s \) covered is \( \frac{L}{2} \):
\( V_b^2 = u^2 + 2a\left(\frac{L}{2}\right) \)
- Simplifying this gives:
\( V_b^2 = u^2 + aL \quad \text{(Equation 2)} \)

4. Substituting for \( aL \):
- From Equation 1, we can express \( aL \):
\( aL = \frac{v^2 - u^2}{2} \)
- Substitute \( aL \) back into Equation 2:
\( V_b^2 = u^2 + \frac{v^2 - u^2}{2} \)

5. Simplifying:
- Combine the terms:
\( V_b^2 = u^2 + \frac{v^2}{2} - \frac{u^2}{2} \)
\( V_b^2 = \frac{2u^2}{2} + \frac{v^2}{2} - \frac{u^2}{2} \)
\( V_b^2 = \frac{v^2 + u^2}{2} \)

6. Final Result:
- Taking the square root to find \( V_b \):
\( V_b = \sqrt{\frac{v^2 + u^2}{2}} \)

Conclusion:
The speed of the middle point of the train as it passes the pole is:
\( V_b = \sqrt{\frac{v^2 + u^2}{2}} \)