An object is thrwon vertically upwards with a velocity of 60 m/s. After what time it strike the ground ? Use g=10m/s2.
To solve the problem of an object thrown vertically upwards with an initial velocity of 60 m/s and determining the time it takes to strike the ground, we can use the equations of motion. Here’s a step-by-step solution:
Step 1: Understand the motion
The object is thrown upwards with an initial velocity (u) of 60 m/s. It will rise to a maximum height and then fall back down to the ground. The total displacement when it strikes the ground is 0.
Step 2: Use the equation of motion
We can use the second equation of motion:
s=ut+12at2
Where:
- s is the displacement (0 in this case, since it returns to the same position),
- u is the initial velocity (60 m/s),
- a is the acceleration (which is -g, or -10 m/s², since gravity acts downwards),
- t is the time in seconds.
Step 3: Set up the equation
Since the displacement s=0:
0=ut+12at2
Substituting the known values:
0=60t−12⋅10⋅t2
0=60t−5t2
Step 4: Rearranging the equation
Rearranging gives:
5t2−60t=0
Step 5: Factor the equation
Factoring out t:
t(5t−60)=0
Step 6: Solve for t
Setting each factor to zero gives:
1. t=0 (the time at which the object was thrown)
2. 5t−60=0 → 5t=60 → t=605=12 seconds
Conclusion
The object will strike the ground after 12 seconds.
---
The object is thrown upwards with an initial velocity (u) of 60 m/s. It will rise to a maximum height and then fall back down to the ground. The total displacement when it strikes the ground is 0.
We can use the second equation of motion:
s=ut+12at2
Where:
- s is the displacement (0 in this case, since it returns to the same position),
- u is the initial velocity (60 m/s),
- a is the acceleration (which is -g, or -10 m/s², since gravity acts downwards),
- t is the time in seconds.
Since the displacement s=0:
0=ut+12at2
Substituting the known values:
0=60t−12⋅10⋅t2
0=60t−5t2
Rearranging gives:
5t2−60t=0
Factoring out t:
t(5t−60)=0
Setting each factor to zero gives:
1. t=0 (the time at which the object was thrown)
2. 5t−60=0 → 5t=60 → t=605=12 seconds
The object will strike the ground after 12 seconds.
Topper's Solved these Questions
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Try Yourself
40 Videos
- MOTION IN STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN STRAIGHT LINEEXERCISE - Assignment (SECTION - A)
50 Videos
- MOTION IN A STRAIGHT LINEBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOTION IN A STRAIGHT LINEEXERCISE - ASSIGNMENT (SECTION - D)
15 Videos
- MOVING CHARGE AND MAGNESIUMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - MOVING CHARGE AND MAGNESIUMEXERCISE - SECTION D
16 Videos
Similar Questions
If a ball is thrown vertically upwards with a velocity of 40m/s, then velocity of the ball after 2s will be (g=10m/s2)
(a) 15m/s (b) 20m/s (c) 25m/s (d) 28m/s
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionA pebble is thrown vertically upwards with a speed of 20 m s−1. How high will it be after 2 s? (Take g = 10 m s−2)
View SolutionA projectile moving vertically upwards with a velocity of 200 m/s breaks into two equal parts at the height of 490 m.one part starts moving vertically upwards with a velocity of 400 m/s.How much time after the break up wil the other part hit the ground?
View SolutionA ball of mas 2gm is thrown vertically upwards with a speed of 30m/s from a tower of height 35m. (Given g=10m/s2)
View SolutionA body is thrown vertically upwards with an initial velocity of 9.8ms−1. What is its speed and direction after 1 s (g=9.8 m/s2). Also, find the height to which it will rise.
View SolutionA particle P is projected upwards with 80m/s. One second later another particle Q is projected with initial velocity 70m/s. Before either of the particle srikes the ground (g=10m/s2)
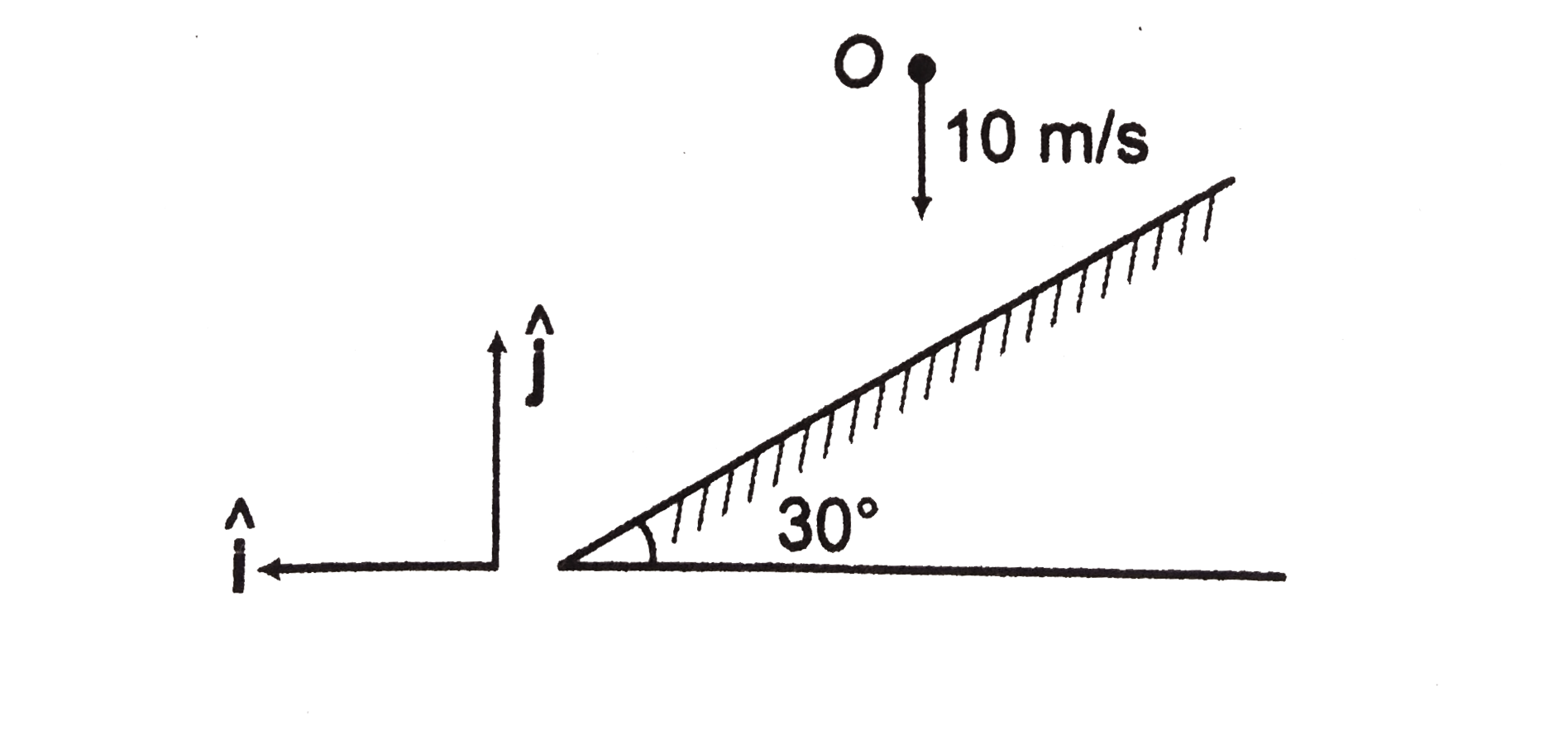
View SolutionAn object is falling vertically downwards with velocity 10m/s.In terms ofˆiandˆj find the image velocity. M
View SolutionA pebble is thrown vertically upwards from a bridge with an initial velocity of 4.9 m/s. It strikes the water after 2s. If acceleration due to gravity is 9.8m/s2(a) what is the height of the bridge? (b) with what velocity does that pebble strike the water?
View Solution
