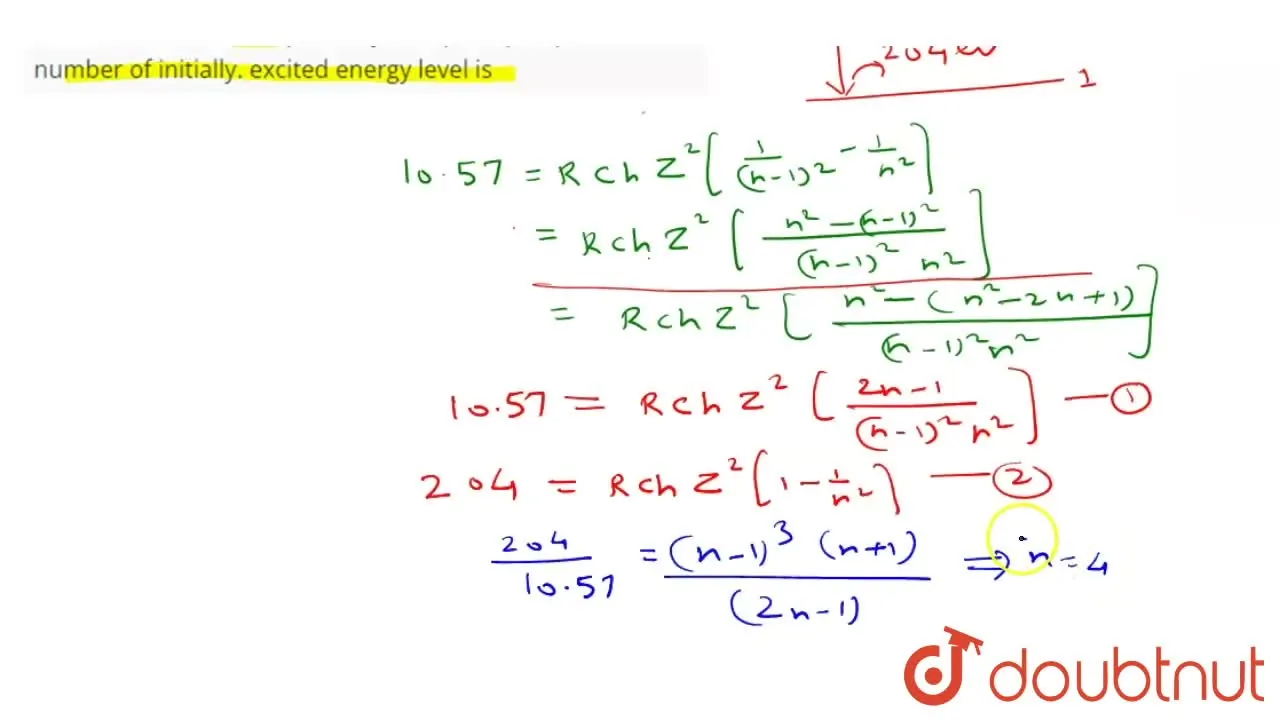
The atom of a hydrogen' like gas are in a particulạr excited energy level. When these ATOMS de-excite, they 'emit photons of different energies. The maximum and minimum energies of emitted photons are Emax=204eV and Emin=10.57eV, respectively. The principal quantum number of initially. excited energy level is
The correct Answer is:4
Step by step video, text & image solution for The atom of a hydrogen' like gas are in a particulạr excited energy level. When these ATOMS de-excite, they 'emit photons of different energies. The maximum and minimum energies of emitted photons are E_(max )=204 eV and E_(min )=10.57 eV, respectively. The principal quantum number of initially. excited energy level is by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
Similar Questions
In a sample of hydrogen atoms, all the atoms exist in two energy levels A and B. A is the ground level and B is some higher energy level. These atoms absorb photons of energy 2.7 eV and attain a higher energy level C.After this, these atoms emit photons of six different energies. Some of these photon energies are higher than 2.7 eV, some equal to 2.7 eV and some are loss than 2.7 eV.
The principal quantum number corresponding to energy level B isView SolutionIn a sample of hydrogen atoms, all the atoms exist in two energy levels A and B. A is the ground level and B is some higher energy level. These atoms absorb photons of energy 2.7 eV and attain a higher energy level C.After this, these atoms emit photons of six different energies. Some of these photon energies are higher than 2.7 eV, some equal to 2.7 eV and some are loss than 2.7 eV.
The principal quantum number corresponding to energy level C isView SolutionA gas of identical H-like atom has some atoms in the lowest (ground) energy level A and some atoms in a particular upper (excited) energy level B and there are no atoms in any other energy level. The atoms of the gas make transition to a higher energy level by aborbing monochromatic light of photon energy 2.7eV. Subsequently, the atoms emit radiation of only six different photons energies. Some of the emitted photons have energy 2.7eV. Some have more and some have less than 2.7eV.
(a) Find the principal quantum number of initially excitied level B.
(b) Find the ionisation energy for the gas atoms.
(c ) Find the maximum and the minimum energies of the emitted photons.View SolutionThe energy of an excited hydrogen atom is -3.4eV. The principal quantum number of the orbit is
View SolutionA sample of hydrogen gas has same atom in out excited state and same atom in other excited state it emits three difference photon.When the sample was irradiated with radiation of energy 2.85eV ,it emits 10 different photon all having energy in or less than 13.6eV ltbrtgt a. Find the principal quantum number of initially excited electrons
b. Find the maximum and minimum energies of the initially emitted photonView SolutionA gas of identical hydrogen-like atoms has some atoms in the lowest in lower (ground) energy level A and some atoms in a partical upper (excited) energy level B and there are no atoms in any other energy level.The atoms of the gas make transition to higher energy level by absorbing monochromatic light of photon energy 2.7eV.
Subsequenty , the atom emit radiation of only six different photon energies. Some of the emitted photons have energy 2.7eV some have energy more , and some have less than 2.7eV.
a Find the principal quantum number of the intially excited level B
b Find the ionization energy for the gas atoms.
c Find the maximum and the minimum energies of the emitted photons.View SolutionA sample of hydrogen gas has some atoms in one excited state. It emits three different photons. When the sample was irradiated with radiation of energy 2.85eV, it emits 10 different photon all having energy less than 13.6eV
a. Find the principal quantum number of initially excited electrons
b. Find the maximum and minimum energies of the initially emitted photons.View SolutionFind the maximum number of photons number of photons emitted by an H-atom, if atom is excited to atates with principal quantum number four.
View SolutionA gas of identical hydrogen-like atoms has some atoms in the lowest in lower (ground) energy level A and some atoms in a partical upper (excited) energy level B and there are no atoms in any other energy level.The atoms of the gas make transition to higher energy level by absorbing monochromatic light of photon energy 2.7eV.
Subsequenty , the atom emit radiation of only six different photon energies. Some of the emitted photons have energy 2.7eV some have energy more , and some have less than 2.7eV.
a Find the principal quantum number of the intially excited level B
b Find the ionization energy for the gas atoms.
c Find the maximum and the minimum energies of the emitted photons.View SolutionIn a sample of hydrogen atoms, all the atoms exist in two energy levels A and B. A is the ground level and B is some higher energy level. These atoms absorb photons of energy 2.7 eV and attain a higher energy level C.After this, these atoms emit photons of six different energies. Some of these photon energies are higher than 2.7 eV, some equal to 2.7 eV and some are loss than 2.7 eV.
The longest wavelength emitted in the radiation spectrum observed isView Solution