A flat, circular coil of radius 10cm has 100 turns of wire. Auniform magnetic field exists in a direction perpendicular to the plane of the coil. This ficld is increasing at the rate of 0.1Ts. Calculate the magnitude of emf induced (in volt) in the coil.
The correct Answer is:0.31
To solve the problem, we will use Faraday's law of electromagnetic induction, which states that the induced electromotive force (emf) in a coil is equal to the negative rate of change of magnetic flux through the coil. The formula for induced emf (ε) is given by:
ϵ=−NdΦdt
Where:
- N = number of turns in the coil
- dΦdt = rate of change of magnetic flux
Step 1: Calculate the area of the coil
The area A of a circular coil is given by the formula:
A=πr2
Where r is the radius of the coil. Given that the radius r=10cm=0.1m:
A=π(0.1)2=π(0.01)=0.01πm2
Step 2: Calculate the rate of change of magnetic flux
The magnetic flux Φ through the coil is given by:
Φ=B⋅A
Where B is the magnetic field. The rate of change of magnetic flux is:
dΦdt=AdBdt
Given that dBdt=0.1T/s:
dΦdt=A⋅dBdt=(0.01π)⋅(0.1)=0.001πWb/s
Step 3: Calculate the induced emf
Now, substituting the values into the induced emf formula:
ϵ=−NdΦdt
Given that N=100:
ϵ=−100⋅(0.001π)=−0.1πV
Step 4: Calculate the numerical value
Using the value of π≈3.14:
ϵ≈−0.1⋅3.14=−0.314V
Thus, the magnitude of the induced emf is:
ϵ≈0.314V
Final Answer:
The magnitude of the induced emf in the coil is approximately 0.314 V.
---
- N = number of turns in the coil
- dΦdt = rate of change of magnetic flux
The area A of a circular coil is given by the formula:
The magnetic flux Φ through the coil is given by:
Now, substituting the values into the induced emf formula:
Using the value of π≈3.14:
The magnitude of the induced emf in the coil is approximately 0.314 V.
Topper's Solved these Questions
Similar Questions
Shows a coil placed in a decreasing magnetic field applied perpendicular to the plane of the coil. The magnetic field is decreasing at a rate of 10Ts−1. Find out current in magnitude and direction of current.

View SolutionA circular coil of radius 2.00 cm has 50 turns. A uniform magnetic field B= 0.200 T exists in the space is a direction parallel to the axis of the loop. The coil is now rotated about a diameter through an angle of 60.0∘. The operation takes 0.100 s. (a) find the average emf induced in the coil. (b) if the coil is a closed one(with the two ends joined together) and has a resistance of 4.00Ω. calculate the net charge crossing a cross- section of the wire of the coil.
View Solution
Knowledge Check
A coil of area 10 cm2 has 200 turns. Magnetic field of 0.1 Wb/m2 is perpendicular to the plane of the coil. The field is reduced to zero in 0.1 s, the induced emf in the coil is
A1VB0.2VC2VD0A circular coil of radius 10 cm having 100 turns carries a current of 3.2 A. The magnetic field at the center of the coil is
A2.01×10−3TB5.64×10−3TC2.64×10−3TD5.64×10−3TA circular coil of radius 2.00cm has 50 turns. A uniform magnetic field B=0.2T exists in the space in a direction parallel to the axis of rthe loop. The coil is now rotated about a diameter through an angle of 60∘ . The operation takes 0.Is . The average emf induced in the coil is
A6.28×10−2VB6.28×10−3VC62.8×10−2VD628×10−2V
Find the emf induced in the coil shown in figure.The magnetic field is perpendicular to the plane of the coil and is constant.
View SolutionFind the emf induced in the coil shown in figure. The magnetic field is perpendicular to the plane of the coil and is constant.
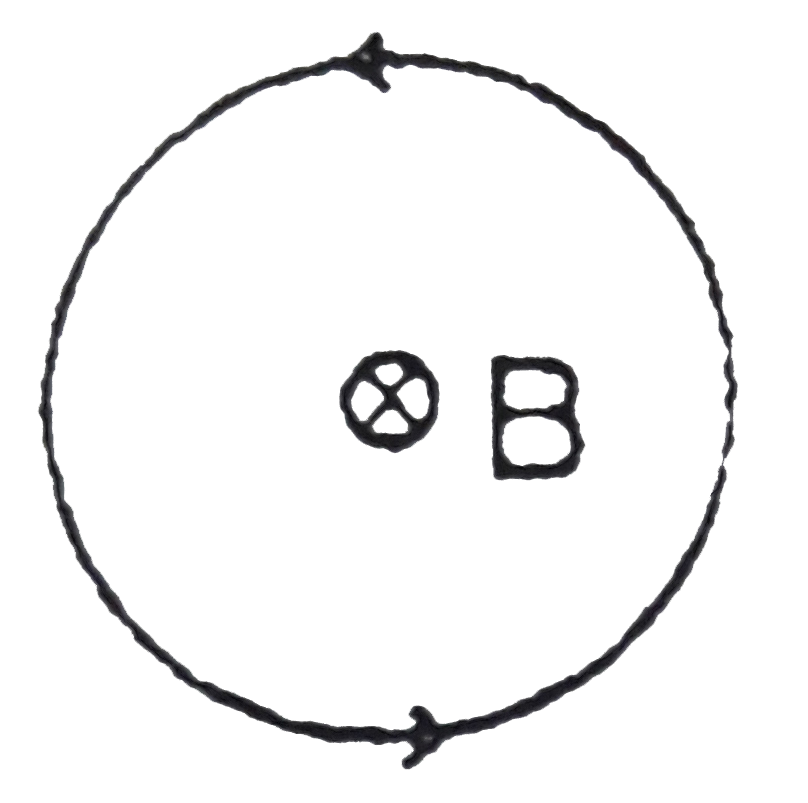
View SolutionFind the direction of induced current in the coil shown in figure.Magnetic field is perpendicular to the plane of coil and it is increasing with time.
View SolutionA rectangular coil is placed in a region having a uniform magnetic field B perpendicular to the plane of the coil. An emf will not be induced ion the coil if the

View SolutionA circular coil of radius 10cmand100 turns carries a current 1A. What is the magnetic moment of the coil?
View Solution
