Two identical magnetic dipoles of magnetic moment 2Am2 are placed at a separation of 2m with their axes perpendicular to each other in air. The resultant magnetic field at a midpoint between the dipoles is x√y×10−7T.F∈d(x+y).
The correct Answer is:7
To solve the problem, we need to calculate the resultant magnetic field at the midpoint between two identical magnetic dipoles placed at a separation of 2 meters, with their axes perpendicular to each other. The magnetic moment of each dipole is given as 2Am2.
Step 1: Understand the Configuration
We have two magnetic dipoles, m1 and m2, each with a magnetic moment m=2Am2. They are placed at a distance of d=2m apart, and their axes are perpendicular to each other.
Step 2: Determine the Midpoint
The midpoint between the two dipoles is at a distance of 1m from each dipole.
Step 3: Calculate the Magnetic Field Due to One Dipole
The magnetic field B at a point along the axis of a magnetic dipole is given by the formula:
B=μ04π⋅2mr3
where:
- μ0=4π×10−7T m/A (permeability of free space),
- m is the magnetic moment,
- r is the distance from the dipole to the point of interest.
For our case, at the midpoint (1 m from each dipole):
B1=μ04π⋅2⋅213=μ04π⋅41=4μ04π=μ0π
Step 4: Calculate the Magnetic Field Due to the Second Dipole
Since the second dipole is perpendicular to the first, the magnetic field at the midpoint due to the second dipole will also be calculated using the same formula, but it will be perpendicular to the first dipole's field:
B2=μ04π⋅41=μ0π
Step 5: Calculate the Resultant Magnetic Field
Since B1 and B2 are perpendicular to each other, we can find the resultant magnetic field BR using the Pythagorean theorem:
BR=√B21+B22=√(μ0π)2+(μ0π)2=√2(μ0π)2=μ0π√2
Step 6: Substitute the Value of μ0
Now, substituting μ0=4π×10−7:
BR=4π×10−7π√2=4×10−7√2T
Step 7: Express in the Required Form
The problem states that the resultant magnetic field can be expressed as x√y×10−7T. Here, we have:
BR=4√2×10−7T
Thus, x=4 and y=2.
Step 8: Find x+y
Finally, we calculate:
x+y=4+2=6
Final Answer
The answer is 6.
We have two magnetic dipoles, m1 and m2, each with a magnetic moment m=2Am2. They are placed at a distance of d=2m apart, and their axes are perpendicular to each other.
The midpoint between the two dipoles is at a distance of 1m from each dipole.
The magnetic field B at a point along the axis of a magnetic dipole is given by the formula:
B=μ04π⋅2mr3
where:
- μ0=4π×10−7T m/A (permeability of free space),
- m is the magnetic moment,
- r is the distance from the dipole to the point of interest.
B1=μ04π⋅2⋅213=μ04π⋅41=4μ04π=μ0π
Since the second dipole is perpendicular to the first, the magnetic field at the midpoint due to the second dipole will also be calculated using the same formula, but it will be perpendicular to the first dipole's field:
B2=μ04π⋅41=μ0π
Since B1 and B2 are perpendicular to each other, we can find the resultant magnetic field BR using the Pythagorean theorem:
BR=√B21+B22=√(μ0π)2+(μ0π)2=√2(μ0π)2=μ0π√2
Now, substituting μ0=4π×10−7:
BR=4π×10−7π√2=4×10−7√2T
The problem states that the resultant magnetic field can be expressed as x√y×10−7T. Here, we have:
BR=4√2×10−7T
Thus, x=4 and y=2.
Finally, we calculate:
x+y=4+2=6
The answer is 6.
Topper's Solved these Questions
Similar Questions
Two identical magnetic dipoles of magnetic moments 1⋅0Am2 each are placed at a separation of 2m with their axes perpendicular to each other. What is the resultant magnetic field at a point midway between the dipoles?
View SolutionFigure shows two identical magnetic dipoles aandb of magnetic moments M each, placed at a separation d, with their axes perpendicular to each other. Find the magnetic field at the point P midway between the dipoles.
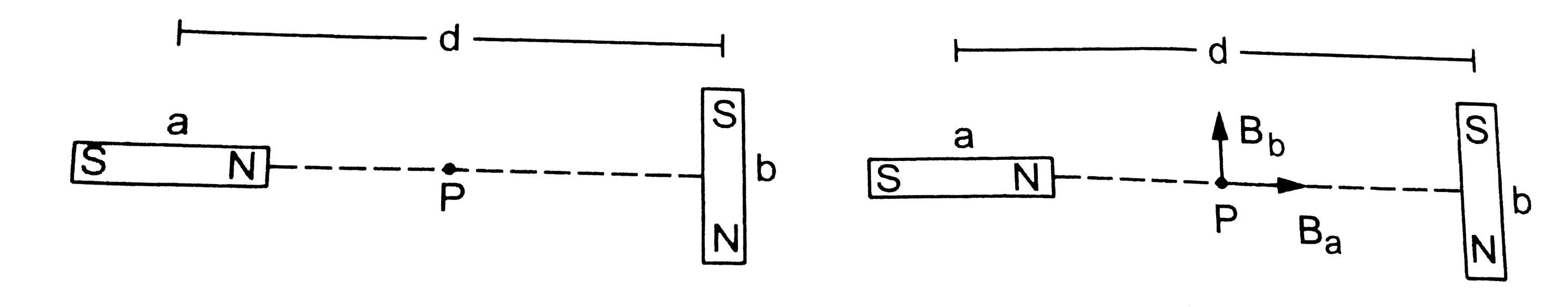
View Solution
Knowledge Check
Iwo identical magnetic dipoles of magnetic moment 2 A m2 are placed at a separation of 2 m with their axes perpendicular to each other in air. The resultant inagnetic field at a midpoint between the dipoles is
A4√5×10−5TB2√5×10−5TC4√5×10−7TD2√5×10−7TTwo identical magnetic dipoles of magnetic moments 2Am2 are placed at a separation of 2m with their axes perpendicular to each other in air. The resultant magnetic field at a mid point between the dipole is
A4√5×10−5TB2√5×10−5TC4√5×10−7TD2√5×10−7TTwo identical magnetic dipoles of magnetic moments 4A−m2 each, are placed at a separation of 4 m with their axis perpendicular to each other. The resultant magnetic field at a point midway between the dipoles is
A5×10−7TB√5×10−7TC10−7TD√52×10−7T
Two identical short bar magnets each of magnetic moment 12⋅5Am2 are placed at a separation of 10cm between their centres, such that their axes are perpendicular to each other. Find the magnetic field at a point midway between the two magnets.
View SolutionTwo identical bar magnets of magnetic pole strength 10 Am and length 10 cm are placed 2 m apart such that their axes are perpendicular to each other. Calculate the resultant magnetic field at a point midway between the magnets.
View SolutionTwo identcal magnetic dipole of magnetic moment 1.0A−m2 each, placed at a separation of 2 m with their axis perpendicular to each other. The resultant magnetic field at a point midway between the dipole is
View SolutionTwo identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View SolutionTwo identical short bar magnets, each having magnetic moment M, are placed a distance of 2d apart with axes perpendicular to each other in a horizontal plane. The magnetic induction at a point midway between them is
View Solution
