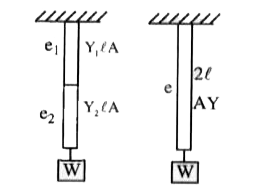
Two rods of identical dimensions with Young's modulus Y1 and Y2 are joined end to end. The equivalent Young's modulus for the composite rod isSolution in Bengali
(A) 2Y1Y2Y1+Y2
(B) Y1Y2Y1+Y2
(C) 12(Y1+Y2)
(D) Y1+Y2
The correct Answer is:A
Step by step video, text & image solution for Two rods of identical dimensions with Young's modulus Y_1 and Y_2 are joined end to end. The equivalent Young's modulus for the composite rod is by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
The velocity of the hands of the watch
View SolutionA solid sphere and a hollow sphere of equal mass and radius are placed over rough horizontal surface after rotating it about its centre of mass with some angular velocity w_0 once the pure rolling starts let V_1 and V_2 be the linear speeds of their centres of mass then
View SolutionTwo wires of equal length and cross sectional area suspended as shown in Their Young's modulii are Y_1 = 2xx10^(11) Pa and Y_2 = 0.90 xx 10^(11) Pa respectively. What will be the equivalent Young's modulus of combination?
View SolutionWhat are dimensions of Young's modulus of elasticity?
View SolutionYoung moduli of two rods of equal length and equal cross section and Y1 and Y2. These rods are joined end to end forming a composite rod-system. Prove that the equivalent Young's modulus of the composite system of rods =2Y1Y2Y1+Y2
View SolutionTwo rods of equal length and cross- sectional area have their Young's moduli Y_1 and Y_2 respectively. IF the rods are joined end to end, then the equivalent Young's modulus of the combined rod system is
View SolutionTwo rods of different materials but of the same sectional area have lengths L_1 and L_2 . IF their Young's moduli are Y_1 and Y_2 respectively. Then calculate the equivalent Young's modulus of the composite system when the two rods are joined along their lengths.
View SolutionState the units and dimensions of young's modulus.
View SolutionTwo wires of same length and radius are joined end to end and loaded. The Young.s modulii of the materials of the two wires are Y1andY2. If the combination behaves as a single wire them its Young.s modulus is
View SolutionGive the dimension of young's modulus.
View SolutionGive the dimension of Young's modulus.
View SolutionThe dimensions of Young's modulus of elasticity is
View SolutionYoung's Modulus||Modulus OF Rigidity||Bulk Modulus||Energy Density||Elongation OF Rod Due to Own Weight
View SolutionWhat will be the Young's modulus of the rod
View SolutionTwo wires of equal length and cross sectional area suspended as shown in Their Young's modulii are Y_1 = 2xx10^(11) Pa and Y_2 = 0.90 xx 10^(11) Pa respectively. What will be the equivalent Young's modulus of combination?
View Solution