A body of mass m is moving in a circle of radius r with a constant speed v, The force on the body is mv2r and is directed towards the centre what is the work done by the from in moving the body over half the circumference of the circle?
mv2πr2
zero
mv2r2
πr2mv2
The correct Answer is:B
To solve the problem, we need to determine the work done by the centripetal force on a body of mass m moving in a circle of radius r with a constant speed v as it moves over half the circumference of the circle.
1. Understanding the Forces Involved:
- The body is moving in a circular path, and the force acting on it is the centripetal force, given by F=mv2r.
- This force is always directed towards the center of the circle.
2. Identifying the Direction of Motion:
- As the body moves along the circular path, its displacement is tangential to the circle at any point.
- When the body moves from one point on the circle to the opposite point (half the circumference), the displacement is tangential to the circle.
3. Calculating Work Done:
- Work done W by a force is defined as:
W=∫→F⋅d→r
- Here, →F is the centripetal force directed towards the center, and d→r is the displacement vector along the path of motion.
4. Angle Between Force and Displacement:
- The angle θ between the centripetal force (which is radial) and the displacement (which is tangential) is 90∘.
- Therefore, the dot product →F⋅d→r can be expressed as:
W=F⋅dr⋅cos(θ)=F⋅dr⋅cos(90∘)
- Since cos(90∘)=0, we have:
W=F⋅dr⋅0=0
5. Conclusion:
- The work done by the centripetal force in moving the body over half the circumference of the circle is:
W=0
Final Answer:
The work done by the force in moving the body over half the circumference of the circle is 0.
- The body is moving in a circular path, and the force acting on it is the centripetal force, given by F=mv2r.
- This force is always directed towards the center of the circle.
- As the body moves along the circular path, its displacement is tangential to the circle at any point.
- When the body moves from one point on the circle to the opposite point (half the circumference), the displacement is tangential to the circle.
- Work done W by a force is defined as:
W=∫→F⋅d→r
- Here, →F is the centripetal force directed towards the center, and d→r is the displacement vector along the path of motion.
- The angle θ between the centripetal force (which is radial) and the displacement (which is tangential) is 90∘.
- Therefore, the dot product →F⋅d→r can be expressed as:
W=F⋅dr⋅cos(θ)=F⋅dr⋅cos(90∘)
- Since cos(90∘)=0, we have:
W=F⋅dr⋅0=0
- The work done by the centripetal force in moving the body over half the circumference of the circle is:
W=0
The work done by the force in moving the body over half the circumference of the circle is 0.
Similar Questions
Knowledge Check
A body of mass m is moving in a circle of radius r with a constant speed v. The work done by the centripetal force in moving the body over half the circumference of the circle is
Amv2/rBzeroCmv2/rDr2/mv2
The acceleration of an object moving in a circle of radius R with uniform speed v is
View SolutionA block of mass m is moving in a circle of radius R with speed v inside a smooth cone as shown in figure. Choose the wrong options
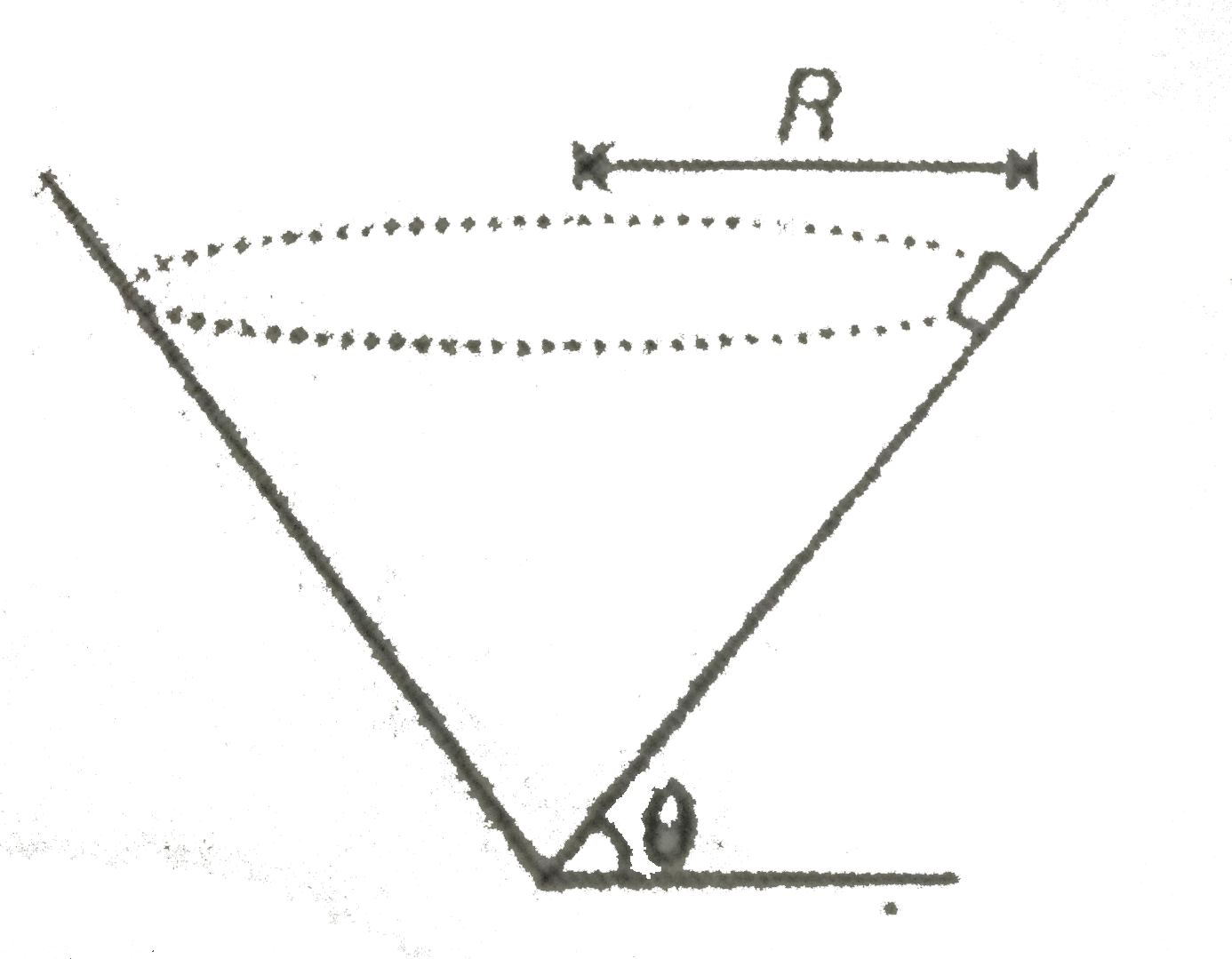
View SolutionWhen a body moves with a constant speed along a circle
View SolutionIf a particle of mass m is moving in a horizontal circle of radius r with a centripetal force (−1/r2) , the total energy is
View SolutionA train of mass M is moving on a circular track of radius R with constant speed v. The length of the train is half of the perimeter of the track. The linear momentum of the train will be
View SolutionA train of mass M is moving on a circular track of radius R with constant speed v. The length of the train is half of the perimeter of the track. The linear momentum of the train will be
View SolutionA particle is moving in a circle of radius r having centre at O with a constant speed v . The magnitude of change in velocity in moving from A to B is
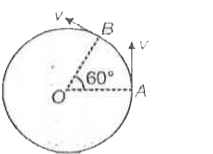
View Solution
