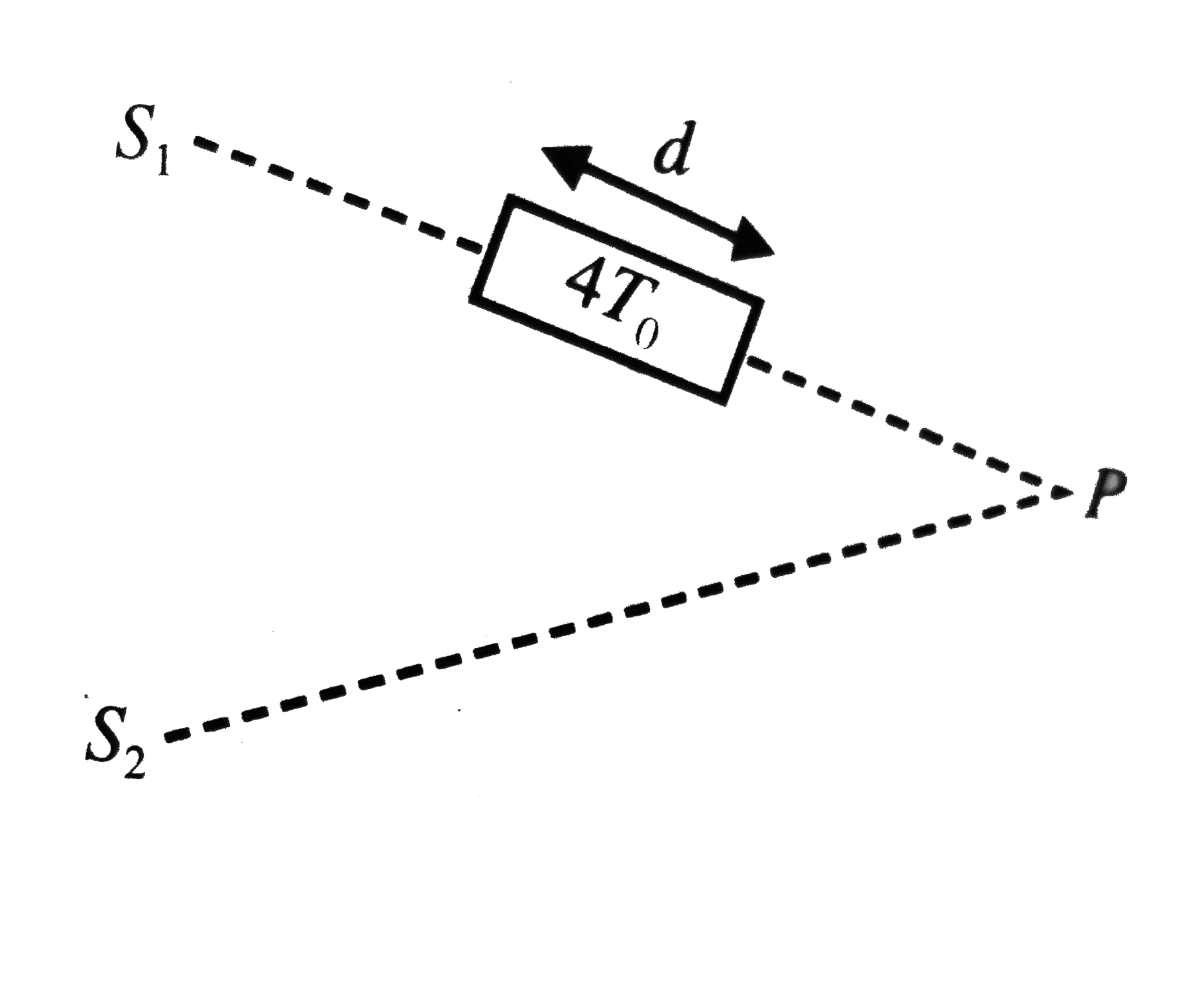
Sound from coherent sources S1andS2 are sent in phase and detected at point P equidistant from both the sources . Speed of sound in normal air in V0, but in some part in path S1 , there is a zone of hot air having temperature 4× , the normal temperature , and width d. What should be minimum frequency of sound , so that minima can be found at P?
The correct Answer is:fmax=Vd
Path differnce =(μ−1)d
μ=V0V'=√γR(T0)m0√γR(4T0)m0=12
(i) |pathd⇔ernce|=∣∣∣(12−1)d∣∣∣=d2
For minima , path difference =(2n+1)λ2=d2
d(2n+1)=λ=Vf
f=V(2n+1)d
fmin=Vd
Topper's Solved these Questions
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Single Correct
144 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Multiple
26 Videos
- SUPERPOSITION AND STANDING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SUPERPOSITION AND STANDING WAVESEXERCISE - Exercise 7.2
32 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - THERMODYNAMICSEXERCISE - 24
1 Videos
Similar Questions
Knowledge Check
In the case of light waves from two coherent sources S1 and S2, there will be constructive interference at an arbitrary point P, the path difference S1P−S2P is
A(n+12)λBnλC(n−12)λDλ2
The speed of sound in air is 330 ms−1 and in water is 1650 ms−1. It takes 2 s for sound to reach a certain distance from the source placed in air. Find the distance.
View SolutionSpeed of sound in air is 330m/s.Find maximum and minimum wavelength of audible sound in air.
View SolutionAt normal temperature and pressure, the speed of sound in air is 332ms−1. What will be the speed of sound in hydrogen (i) at normal temperature and pressure (ii) at 819∘C temperature and 4 atmospheric pressure. Given air is 16 times heavier than hydrogen.
View SolutionTwo identical sources of sound S1andS2 produce intensity I0 at a point P equidistant from each source .
(i) Determine the intensity of each at the point P.
(ii) If the power of S1 is reduced to 64% and phase difference between the two sources is varied continuously , then determine the maximum and minimum intensities at the point P.
(iii) If the power of S1 is reduced by 64% , then determine the maximum and minimum intensities at the point P.
View SolutionAn echo is returned in 3s. What is the distance of the reflecting surface from the source, given that the speed of sound is 342m/s.
View SolutionThe speed of sound in air is 330 ms−1 and in water is 1650 ms−1. It takes 2 s for sound to reach a certain distance from the source placed in air. How much time will it take for sound to reach the same distance when the source is in water ?
View SolutionIn Quincke's experiment the sound detected is changed from a maximum to a minimum when the sliding tube is moved through a distance of 2.50 cm. Find the frequency of sound if the speed of sound in air is 340ms−1.
View Solution
