A point source of sound is placed in a non-absorbing medium two points A and B are at the distance of 1 m and 2 m, respectively, from the source. The ratio of amplitudes of waves at A to B is
1:1
1:4
1:2
2:1
The correct Answer is:d
To solve the problem of finding the ratio of amplitudes of waves at points A and B, we can follow these steps:
Step 1: Understand the relationship between intensity and distance
The intensity (I) of sound from a point source is inversely proportional to the square of the distance (R) from the source. This can be expressed mathematically as:
I∝1R2
Step 2: Define the distances for points A and B
Given:
- Distance from the source to point A, R1=1m
- Distance from the source to point B, R2=2m
Step 3: Calculate the intensity ratio
Using the relationship from Step 1, we can find the ratio of intensities at points A and B:
I1I2=R22R21
Substituting the values:
I1I2=(2)2(1)2=41
Thus, the intensity ratio I1I2=4:1.
Step 4: Relate intensity to amplitude
The intensity of a wave is directly proportional to the square of its amplitude (A). This can be expressed as:
I∝A2
Thus, we can write the ratio of intensities in terms of amplitudes:
I1I2=A21A22
Step 5: Calculate the amplitude ratio
From the intensity ratio calculated in Step 3, we can express the amplitude ratio:
A21A22=41
Taking the square root of both sides gives:
A1A2=√41=2
Thus, the ratio of amplitudes A1:A2=2:1.
Final Answer
The ratio of amplitudes of waves at points A to B is:
2:1
---
The intensity (I) of sound from a point source is inversely proportional to the square of the distance (R) from the source. This can be expressed mathematically as:
I∝1R2
Given:
- Distance from the source to point A, R1=1m
- Distance from the source to point B, R2=2m
Using the relationship from Step 1, we can find the ratio of intensities at points A and B:
I1I2=R22R21
Substituting the values:
I1I2=(2)2(1)2=41
Thus, the intensity ratio I1I2=4:1.
The intensity of a wave is directly proportional to the square of its amplitude (A). This can be expressed as:
I∝A2
Thus, we can write the ratio of intensities in terms of amplitudes:
I1I2=A21A22
From the intensity ratio calculated in Step 3, we can express the amplitude ratio:
A21A22=41
Taking the square root of both sides gives:
A1A2=√41=2
Thus, the ratio of amplitudes A1:A2=2:1.
The ratio of amplitudes of waves at points A to B is:
2:1
Topper's Solved these Questions
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Single Correct Answer
1 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Multiple Correct
25 Videos
- TRAVELLING WAVESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRAVELLING WAVESEXERCISE - Subjective
16 Videos
- TRANSMISSION OF HEATBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - TRANSMISSION OF HEATEXERCISE - Single correct
9 Videos
- VECTORSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A point source emits sound equally in all directions in a non-absorbing medium. Two points P and Q are at the distance of 9meters and 25 meters respectively from the source. The ratio of amplitudes of the waves at P and Q is…..
View SolutionA point source emits sound equally in all directions in a non-absorbing medium. Two point P and Q are at distance of 2m and 3m respectively from the source. The ratio of the intensities of the wave at P and Q is :
View SolutionAn isotropic point source 'S' of sound emits constant power. Two points A and B are situated at distance x and 2x from S. the difference between the loudness of points A and B is about (log 2=0.3)
View SolutionA source oscillates with a frequency 25Hz and the wave propagates with 300m/s. Two points A and B are located at distances 10mand16m away from the source. The phase difference between AandB is
View SolutionThe period of oscillations of a point is 0.04 s and the velocity of propagation of oscillation is 300 m/s. The difference of phases between the oscillations of two points at distance 10 m and 16 m respectively from the source of oscillations is
View SolutionA point sound source is situated in a medium of dulk modulus 1.6×105N/m2. An observer standing at a distance 10mfrom the source writes down the equeation for the wave as y=Asin(15πx−6000πt). Here y and x are in meter and t is in second. The maximum pressure ampulitude received to the observer's ear is (24π) pa, then find.
(a) the density of the medium,
(b) the displacement ampulitude A of the wave recived by the observer and
(c ) the power of the sound source.
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is
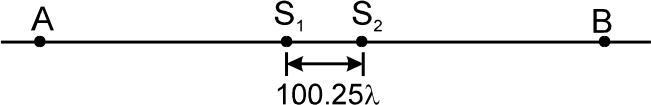
View SolutionS1andS2 are two coherent sources of radiations separated by distance 100.25λ, where λ is the wave length of radiation. S1 leads S2 in phase by π/2.A and B are two points on the line joining S1andS2 as shown in figure.The ratio of amplitudes of component waves from source S1andS2 at A and B are in ratio 1:2. The ratio of intensity at A to that of B (IAIB) is

View SolutionA point source of sound emits a constant power with intensity inversely proportinal to the square of the distance from the source . By how many decible does the sound intensity level drops when you move from point P1 to P2 ? Distance of P2 from the source is two times the distance of source from P1 .
View SolutionIf the amplitude of waves at a distance r from line source is A. The amplitude at a distance 4r will be
View Solution
