A bimetallic strip is formed out of two identical strips one of copper and the other of brass. The temperature of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
proportional to ΔT
inversely proportional to ΔT
proportional to |αB−αC|
inversely proportional to |αB−αC|
The correct Answer is:B, D
To solve the problem regarding the bimetallic strip made of copper and brass, we need to analyze how the radius of curvature R relates to the change in temperature ΔT and the coefficients of linear expansion of the two metals.
1. Understand the Bimetallic Strip: A bimetallic strip consists of two different metals bonded together. When the temperature changes, each metal expands by different amounts due to their different coefficients of linear expansion.
2. Define Coefficients of Linear Expansion: Let αC be the coefficient of linear expansion for copper and αB be the coefficient of linear expansion for brass.
3. Change in Length: When the temperature increases by ΔT, the change in length for each metal can be expressed as:
- For copper: ΔLC=LαCΔT
- For brass: ΔLB=LαBΔT
4. Bending of the Strip: The difference in expansion causes the strip to bend. The curvature of the strip is related to the difference in expansion between the two metals.
5. Radius of Curvature Formula: The radius of curvature R of the bimetallic strip is given by the formula:
R=d|αB−αC|ΔT
where d is the thickness of the strip.
6. Analyze the Proportionality:
- From the formula, we see that R is inversely proportional to |αB−αC| and ΔT.
- This means that as ΔT increases, R decreases, and as the difference in coefficients |αB−αC| increases, R also decreases.
7. Conclusion: Therefore, we conclude that:
- R is inversely proportional to ΔT.
- R is also inversely proportional to |αB−αC|.
Final Answer:
- R is inversely proportional to |αB−αC| and ΔT.
- For copper: ΔLC=LαCΔT
- For brass: ΔLB=LαBΔT
R=d|αB−αC|ΔT
where d is the thickness of the strip.
- From the formula, we see that R is inversely proportional to |αB−αC| and ΔT.
- This means that as ΔT increases, R decreases, and as the difference in coefficients |αB−αC| increases, R also decreases.
- R is inversely proportional to ΔT.
- R is also inversely proportional to |αB−αC|.
- R is inversely proportional to |αB−αC| and ΔT.
Topper's Solved these Questions
- ARCHIVES 1 VOLUME 6Book:CENGAGE PHYSICS ENGLISHChapter:ARCHIVES 1 VOLUME 6Exercise:Assertion-Reasoning
Explore 1 Video
- ARCHIVES 1 VOLUME 6Book:CENGAGE PHYSICS ENGLISHChapter:ARCHIVES 1 VOLUME 6Exercise:Comprehension
Explore 3 Videos
- ARCHIVES 1 VOLUME 6Book:CENGAGE PHYSICS ENGLISHChapter:ARCHIVES 1 VOLUME 6Exercise:Single Correct
Explore 54 Videos
- ARCHIVES 2 VOLUME 6Book:CENGAGE PHYSICS ENGLISHChapter:ARCHIVES 2 VOLUME 6Exercise:Integer
Explore 4 Videos
Similar Questions
A bimetallic strip is formed out of two identical strips one of copper and the other of brass. The coefficients of linear expansion of the strip goes up by ΔTand the strip bends to from an arc of radius of curvature R. Then R is.
View SolutionA bimetallic strip is formed out of two identical strips one of copper and the other of brass. The co-efficients of linear expansion of the two metals are αC and αB. On heating, the temperature of the strip goes up by △T the strip bends to form an are of radius of curvature R. Then R is
View SolutionTwo identical rectangular strips. One of copper and the other of steel, are riveted together to from a bimetallic strip. On heating. the strip will
(αcopper>αsteel)
View SolutionA bimetallic strip of brass and iron is straight at room temperature. When this bimetallic strip is heated it bends, such that:
View Solution
Figure. Shows the graphs of elongation versus temperature for two different metals. If these metals are employed to form a straight bimetallic strip of thickness 6 cm and heated, it bends in the form of an arc, the radius of cuvature chaging with temperature approximately as shown in the figure. The linear expansivities of the two metals are
View SolutionWhat is a bimetallic strip? Why does a bimetallic strip of brass and iron bend on heating?
View SolutionTwo identical thin metal strips one of aluminium and the other of iron are riveted together to form a bimetallic strip. The temperature is raised by 50∘C. If the central planes of the two strips are separated by 2nm and the coefficients of thermal expansion of aluminium and iron are respectively 30×10−6/∘C and 10×10−6/∘C the average radius of curvature of the bimetallic strip is about
View SolutionA mica strip and a polysterence strip are fitted on the two slite of a double slit apparatus. The thickness of the strips is 0.50 mm and the separation between the slits is 0.12 cm. The refractive index of mica and polysterene are 1.58 and 1.55 respectively for the light of wavelength 590 nm which is used in the experiment. The interference is observed on a screen a distance one meter away. (a) What would be the fringe- width ? (b) At what distance from the centre will the first maximum be located ?
View SolutionA solid sphere of radius R/2 is cut out of a solid sphere of radius R such that the spherical cavity so formed touches the surface on one side and the centre of the sphere on the other side, as shown. The initial mass of the solid sphere was M. If a particle of mass m is placed at a distance 2.5R from the centre of the cavity, then what is the gravitational attraction on the mass m?
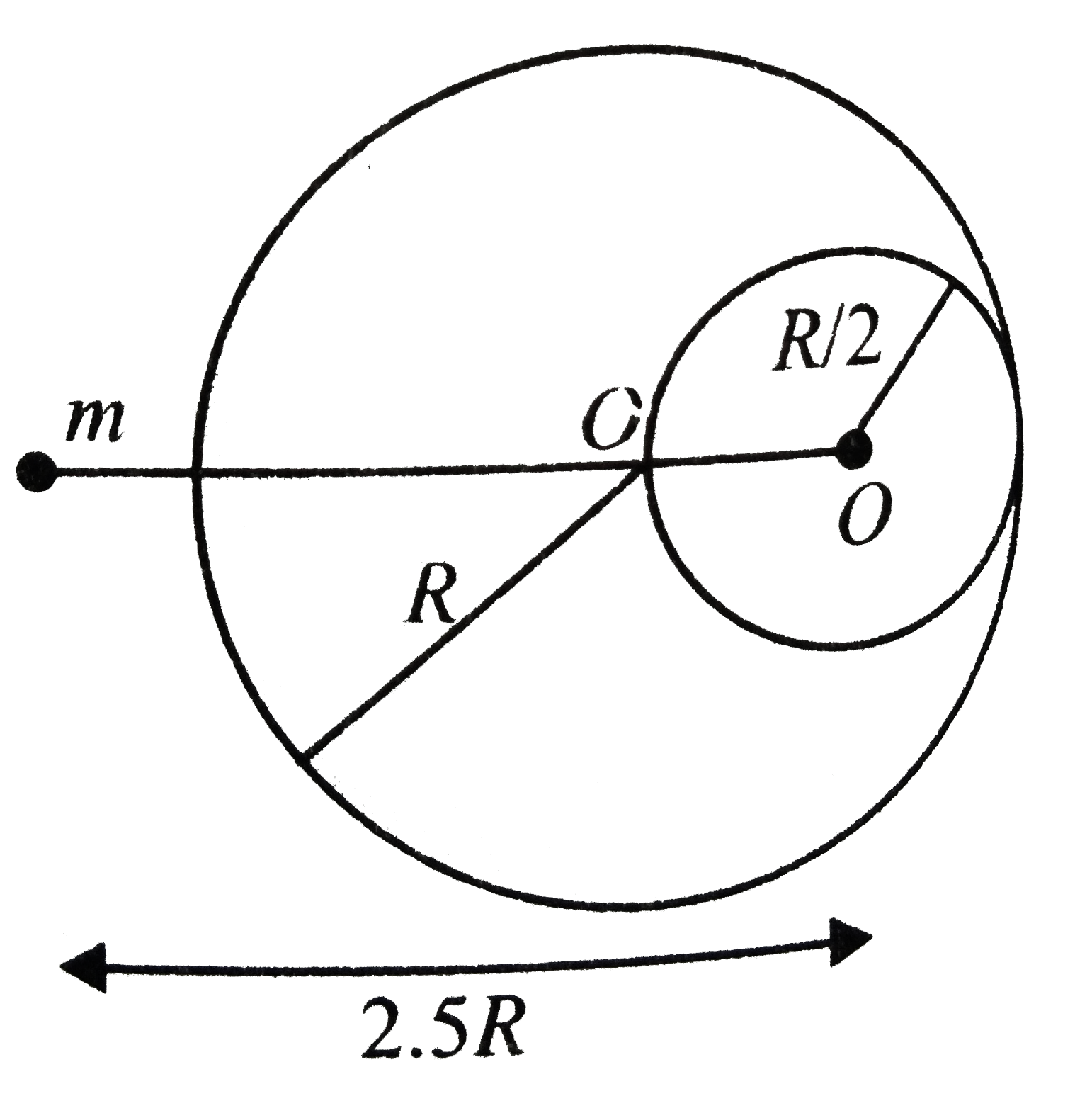
View SolutionA solid sphere of radius R/2 is cut out of a solid sphere of radius R such that the spherical cavity so formed touches the surface on one side and the centre of the sphere on the other side, as shown. The initial mass of the solid sphere was M. If a particle of mass m is placed at a distance 2.5R from the centre of the cavity, then what is the gravitational attraction on the mass m?

View Solution