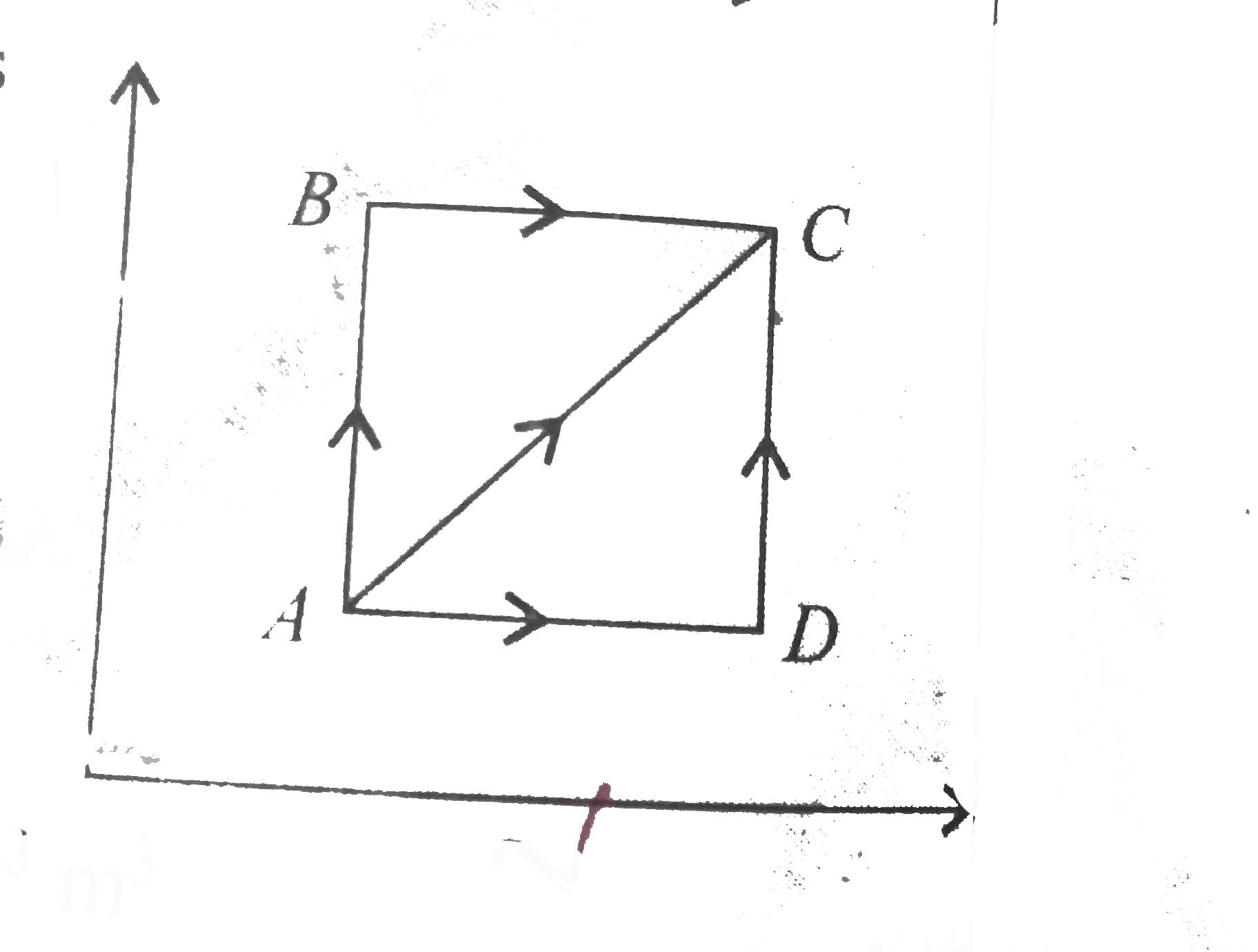
Determine the work done by an ideal gas doing 1→4→3→2→1.
Given P1=105Pa,P0=3×105Pa,P3=4×105Pa and V2−V1=10L.

From Fig.
V4−V3V2−V1=P3−P0P0−P1⇒V4−V310=4×105−3×1053×105−105
V4−V3=5L
Now, work done
W=(12×10×2×105−15×5×1×105)×10−3=750J
Topper's Solved these Questions
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Solved Examples
14 Videos
- KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASES AND FIRST LAW OF THERMODYNAMICSEXERCISE - Exercise 2.1
20 Videos
- KINETIC THEORY OF GASESBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINETIC THEORY OF GASESEXERCISE - Compression
2 Videos
- LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - LINEAR AND ANGULAR SIMPLE HARMONIC MOTIONEXERCISE - Single correct anwer type
14 Videos
Similar Questions
A thermodynamic process is shown in Fig. The pressures and volumes corresponding to some points in the figure are : PA=3×104Pa,PB=8×104Pa and VA=2×10−3m3,VD=5×10−3m.
In process AB,600J of heat is added to the system and in process BC,200J of heat is added to the system. The change in internal energy of the energy of the process AC would be.

View SolutionA thermodynamic process is shown in the following figure. The process and volumes corresponding to some points in the figure are:
PA=3×103Pa,
VA=2×10−3m−3,
PB=3×104Pa,VD=2×10−3m3
In process AB,600J of heat is added to the system and in the process BC,200J of heat is added to the system. the change in internla energy of the system in the process AC would be
View Solution(14)−10×(25)−10
View SolutionIdentify the reaction order from each of the following rate constants
(i)k=3×10−5s−
(ii)k=9×10−4mol−litre s−
(iii)k=6×10−2 litre mol−s−
(iv)k=2.3×10−5Lmol−1s−1
(v)k=3×10−3molL−1s−1
View SolutionIdentify the reaction order from each of the following rate constants
(i)k=3×10−5s−
(ii)k=9×10−4mol−litre s−
(iii)k=6×10−2 litre mol−s−
(iv)k=2.3×10−5Lmol−1s−1
(v)k=3×10−3molL−1s−1
View SolutionIdentify the reaction order from each of the following rate constants
(i)k=3×10−5s−
(ii)k=9×10−4mol−litre s−
(iii)k=6×10−2 litre mol−s−
(iv)k=2.3×10−5Lmol−1s−1
(v)k=3×10−3molL−1s−1
View SolutionWhen temperature of a gas is 20∘C and pressure is changed from p1=1.01×105Pa to p2=1.165×105Pa, the volume changes by 10%. The bulk modulus is
View SolutionRate constant k=1.2×103mol−1Ls−1 and Ea=2.0×102kJmol−1. When T→∞:
View SolutionAn air filled balloon is at a depth of 1km below the water level in an ocean . The normal stress of the balloon (in Pa) is
(Given,ρwater=103kgm−3,g=9.8ms−2 and Patm=105Pa)
View SolutionCalculate the speed of longitudinal waves in the following gases at 0∘C and 1atm(=105pa):
(a) oxygen for which the bulk modulus is 1.41×105 pa and density is 1.43kg/m3 .
(b) helium for which the bulk modulus is 1.7×105 pa and density is 0.18kg/m3 .
View Solution
