A cube and a sphere of equal edge and radius, made of the same substance are allowed to cool under identical conditions. Determine which of the two will cool at a faster rate.
To determine which of the two objects—a cube and a sphere of equal edge and radius, made of the same substance—will cool at a faster rate, we can use the principles of heat transfer and the geometry of the shapes involved.
1. Understanding the Cooling Process:
Both the cube and the sphere are cooling under identical conditions. The rate of cooling can be analyzed using Newton's Law of Cooling or Stefan-Boltzmann Law, which states that the rate of heat loss of a body is proportional to its surface area and inversely proportional to its volume.
2. Define the Shapes:
- For the cube:
- Edge length = a
- Surface Area (A1) = 6a²
- Volume (V1) = a³
- For the sphere:
- Radius = a
- Surface Area (A2) = 4πa²
- Volume (V2) = (4/3)πa³
3. Calculate the Surface Area to Volume Ratios:
- For the cube:
A1V1=6a2a3=6a
- For the sphere:
A2V2=4πa243πa3=3a
4. Compare the Ratios:
- Now we compare the two ratios:
A1V1=6aandA2V2=3a
- Clearly, 6a>3a.
5. Conclusion:
Since the ratio of surface area to volume is greater for the cube than for the sphere, the cube will cool at a faster rate than the sphere. This is because a larger surface area relative to volume allows for more heat to be lost per unit time.
Final Answer:
The cube will cool at a faster rate than the sphere.
Both the cube and the sphere are cooling under identical conditions. The rate of cooling can be analyzed using Newton's Law of Cooling or Stefan-Boltzmann Law, which states that the rate of heat loss of a body is proportional to its surface area and inversely proportional to its volume.
- For the cube:
- Edge length = a
- Surface Area (A1) = 6a²
- Volume (V1) = a³
- For the sphere:
- Radius = a
- Surface Area (A2) = 4πa²
- Volume (V2) = (4/3)πa³
- For the cube:
A1V1=6a2a3=6a
- For the sphere:
A2V2=4πa243πa3=3a
- Now we compare the two ratios:
A1V1=6aandA2V2=3a
- Clearly, 6a>3a.
Since the ratio of surface area to volume is greater for the cube than for the sphere, the cube will cool at a faster rate than the sphere. This is because a larger surface area relative to volume allows for more heat to be lost per unit time.
The cube will cool at a faster rate than the sphere.
Topper's Solved these Questions
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Subjective
25 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Single Correct
148 Videos
- CALORIMETRYBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CALORIMETRYEXERCISE - Exercise 1.2
22 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
- CENTRE OF MASSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - INTEGER_TYPE
1 Videos
Similar Questions
A cube, sphere and a cylinder made of same material shown in figure are allowed to cool under identical conditions. Determine which of these will cool at a faster rate?
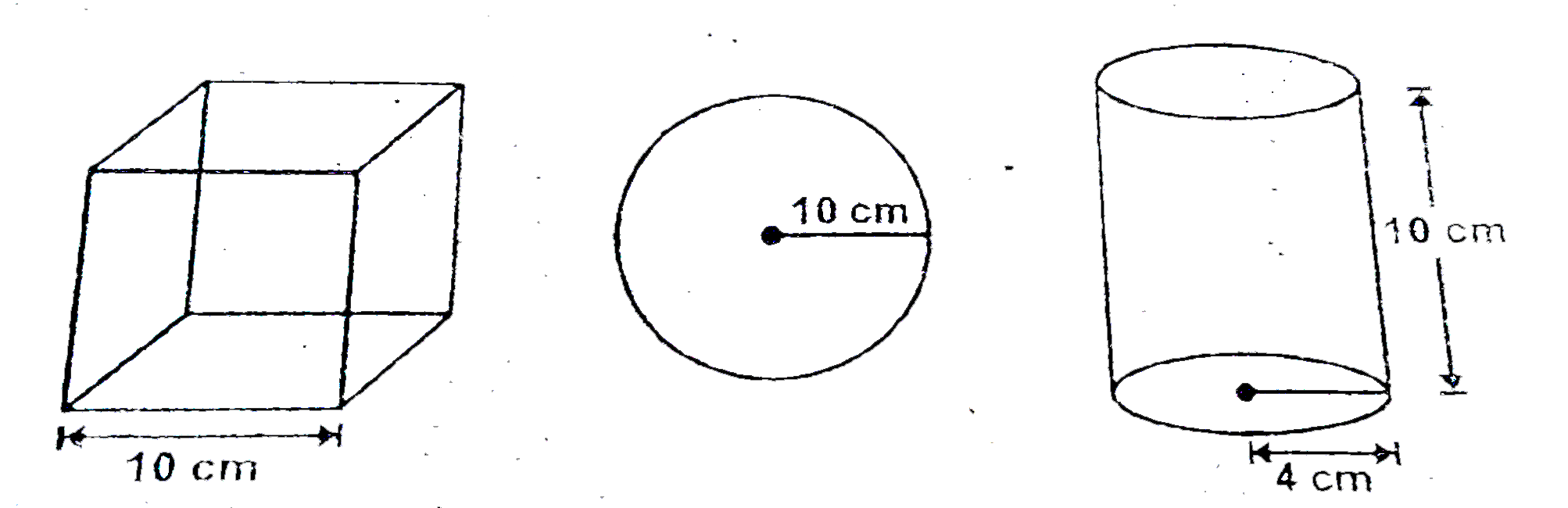 .
.
View SolutionTwo solid spheres of radii R1 and R2 are made of the same material and have similar surfaces. These are raised to the same temperature and then allowed to cool under identical conditions. The ratio of their initial rates of loss of heat are
View Solution
Knowledge Check
A sphere, a cube and a thin circular plate, all made of the same material and having the same mass are initially heated to a temperature of 3000∘C. Which of these will cool fastest ?
AsphereBcubeCplateDnone of these
A solid aluminium sphere and a solid copper sphere of twice the radius are heated to the same temperature and are allowed to cool under identical surrounding temperatures. Assume that the emisssivity of both the spheres is the same. Find ratio of (a) the rate of heat loss from the aluminium sphere to the rate of heat loss from the copper sphere and (b) the rate of fall of temperature of the aluminium sphere to the rate of fall of temperature of copper sphere. The specific heat capacity of aluminium =900Jkg−1C−1 . and that of copper =390Jkg−1C−1 . The density of copper =3.4 times the density of aluminium.
View Solution"A solid sphere of copper of radius R and a hollow sphere of the same material of inner radius r and outer radius R are heated to the same temperature and allowed to cool in the same environment. Which of them starts cooling faster ?''
View SolutionTwo circular disc A and B with equal radii are blackened. They are heated to same temperature and are cooled under identical conditions. What inference do your draw from their cooling curves?
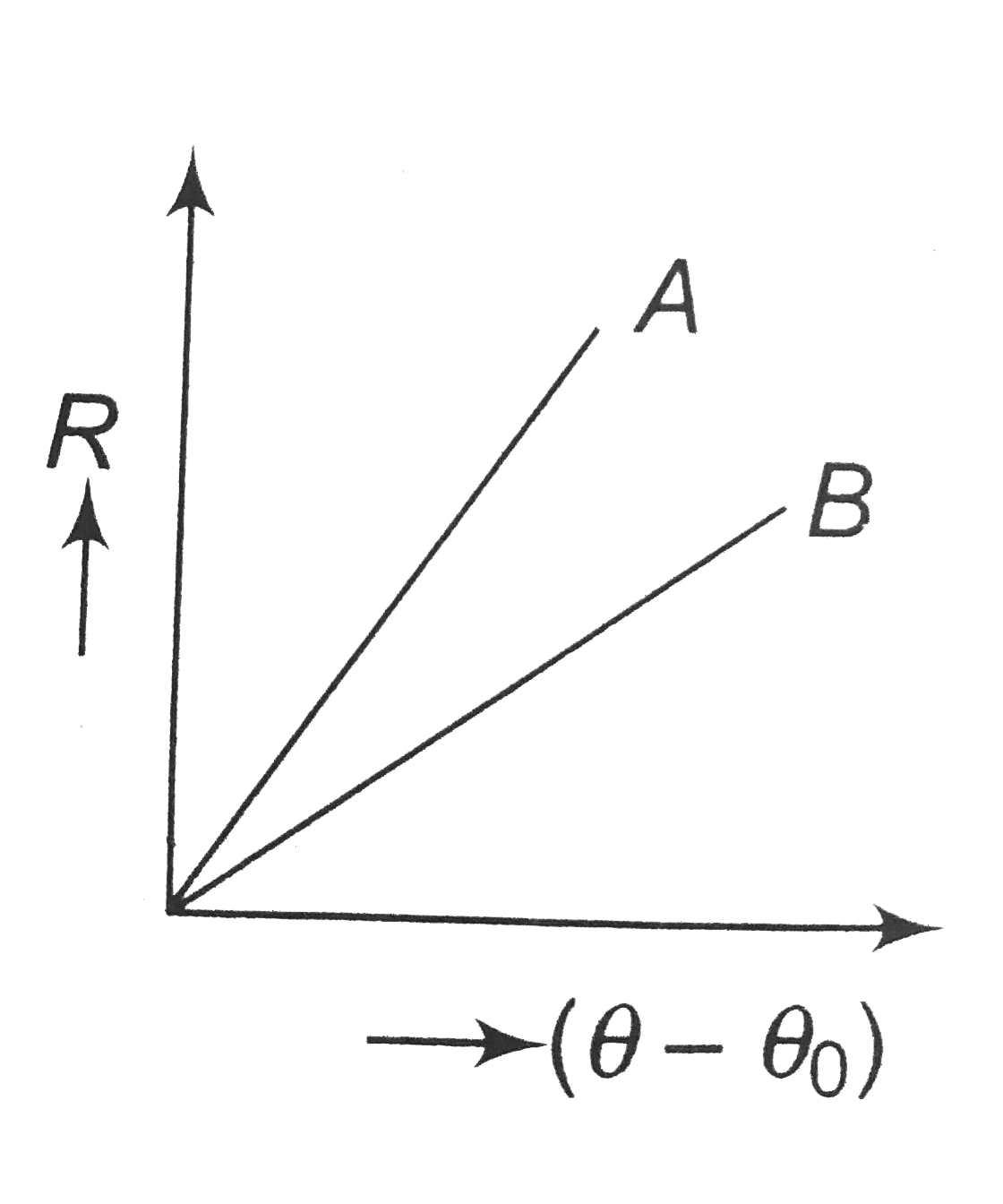
View SolutionA solid cylinder of length L and radius r is heat upto same temperature as that of a cube of edge length a. If both have same material, volume and allowed to cool under similar conditions, then ratio of amount of radiations radiated will be (Neglect radiation emitted from flat surfaces of the cylinder)
View SolutionTwo spheres of same material and radius r and 2r are heated to same temperature and are kept in identical surroundings, ratio of their rate of loss of heat is
View SolutionA sphere, a cube and a thin circular pate are heated to the same temperature. If they are made of same material and have equal masses, determine which of these three object cools the fastest and which one cools the slowest?
View SolutionTwo spheres made of same substance have diameters in the ratio 1:2. their thermal capacities are in the ratio of
View Solution
