The height of a mercury column measured with a brass scale, which is correct and equal to at , is at ? The coefficient of linear expansion of brass is and the coefficient of linear expansion of brass is and the corfficient of volume expansion of mercury is . Relate and .
To solve the problem, we need to relate the height of the mercury column at two different temperatures, H0 at 0∘C and H1 at t∘C, considering the thermal expansions of both the brass scale and the mercury.
1. Understand the Expansion of Brass and Mercury:
- The height H0 is measured with a brass scale at 0∘C.
- At a temperature t∘C, the brass scale expands due to thermal expansion.
- The linear expansion of brass is given by:
L=L0(1+αΔT)
- For the brass scale, the change in temperature ΔT=t−0=t, so the new height H1 can be expressed as:
H1=H0(1+αt)
2. Consider the Volume Expansion of Mercury:
- The volume expansion of mercury is given by:
V=V0(1+γΔT)
- Since the height of the mercury column is affected by its volume expansion, we need to express the change in the density of mercury due to temperature change. The density at temperature t is:
ρt=ρ01+γt
3. Apply the Hydrostatic Pressure Condition:
- The pressure exerted by the mercury column at two different temperatures must be equal:
ρ0gH0=ρtgH1
- Substituting for ρt:
ρ0gH0=ρ01+γtgH1
- Canceling g and ρ0 from both sides gives:
H0=H11+γt
4. Combine the Results:
- From the expansion of the brass scale, we have:
H1=H0(1+αt)
- Substitute H1 back into the equation:
H0=H0(1+αt)1+γt
- Simplifying this gives:
1=(1+αt)(1+γt)
5. Final Relation:
- Rearranging gives the final relation between H0 and H1:
H0(1+γt)=H1(1+αt)
- Thus, we can express H0 in terms of H1:
H0=H1(1+αt)(1+γt)
- The height H0 is measured with a brass scale at 0∘C.
- At a temperature t∘C, the brass scale expands due to thermal expansion.
- The linear expansion of brass is given by:
L=L0(1+αΔT)
- For the brass scale, the change in temperature ΔT=t−0=t, so the new height H1 can be expressed as:
H1=H0(1+αt)
- The volume expansion of mercury is given by:
V=V0(1+γΔT)
- Since the height of the mercury column is affected by its volume expansion, we need to express the change in the density of mercury due to temperature change. The density at temperature t is:
ρt=ρ01+γt
- The pressure exerted by the mercury column at two different temperatures must be equal:
ρ0gH0=ρtgH1
- Substituting for ρt:
ρ0gH0=ρ01+γtgH1
- Canceling g and ρ0 from both sides gives:
H0=H11+γt
- From the expansion of the brass scale, we have:
H1=H0(1+αt)
- Substitute H1 back into the equation:
H0=H0(1+αt)1+γt
- Simplifying this gives:
1=(1+αt)(1+γt)
- Rearranging gives the final relation between H0 and H1:
H0(1+γt)=H1(1+αt)
- Thus, we can express H0 in terms of H1:
H0=H1(1+αt)(1+γt)
Topper's Solved these Questions
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Exercise 1.3
Explore 18 Videos
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Subjective
Explore 25 Videos
- CALORIMETRYBook:CENGAGE PHYSICS ENGLISHChapter:CALORIMETRYExercise:Exercise 1.1
Explore 23 Videos
- BASIC MATHEMATICSBook:CENGAGE PHYSICS ENGLISHChapter:BASIC MATHEMATICSExercise:Exercise 2.6
Explore 20 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICS ENGLISHChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
Can we related the thermal coefficient of linear expansion, α, to the thermal coefficient of volume expansion?
View SolutionLet γ : coefficient of volume expansion of the liquid and α : coefficient of linear expansion of the material of the tube
View Solution
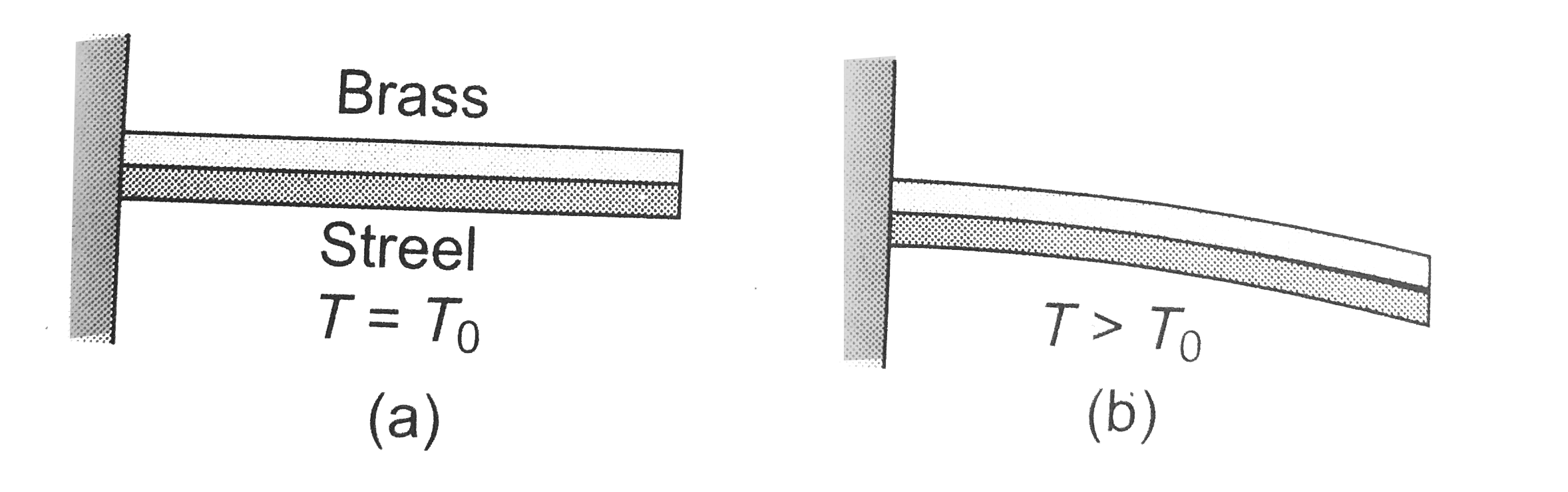
In figure which strip (brass or steel) has higher coefficient of linear expansion.
View SolutionIn figure which strip brass or steel have higher coefficient of linear expansion.
View SolutionThe coefficient of linear expansion of crystal in one direction is α1 and that in every direction perpendicular to it is α2. The coefficient of cubical expansion is
View SolutionThe coefficient of linear expansion of crystal in one direction is α1 and that in every direction perpendicular to it is α2. The coefficient of cubical expansion is
View SolutionThe apparent coefficient of expansion of a liquid when heated in a brass vessel is X and when heated in a tin vessel is Y. If α is the coefficient of linear expansion for brass, the coefficient of linear expansion of tin is
View SolutionCoefficient of areal expansion of a solid is 2×10−5.∘C−1. Calculate its coefficient of linear expansion.
View SolutionThe real coefficient of volume expansion of glycerine is 0.000597per∘C and linear coefficient of expansion of glass is 0.000009par∘C . Then the apparent volume coefficient of glycerine is
View SolutionThe real coefficient of volume expansion of glycerine is 0.000597per∘C and linear coefficient of expansion of glass is 0.000009par∘C . Then the apparent volume coefficient of glycerine is
View Solution